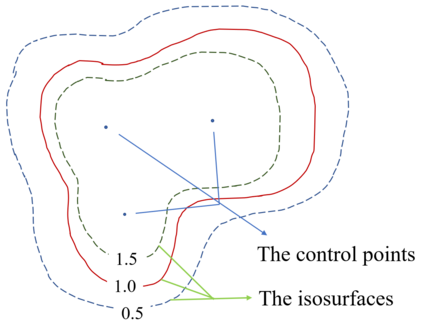
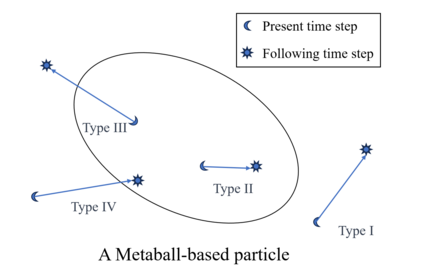
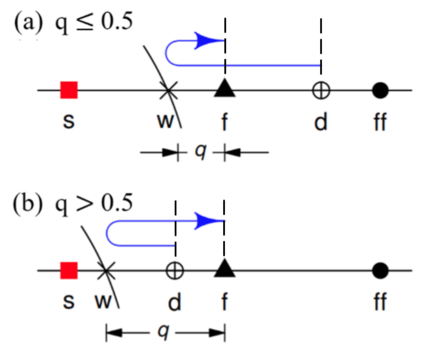
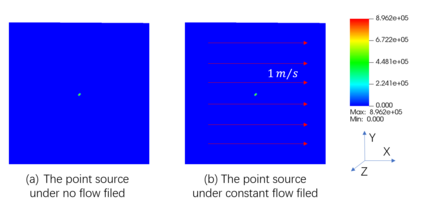
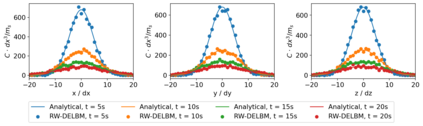
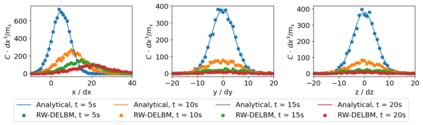
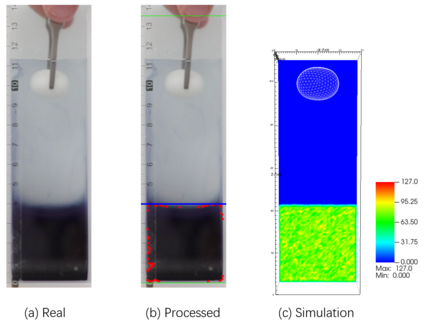
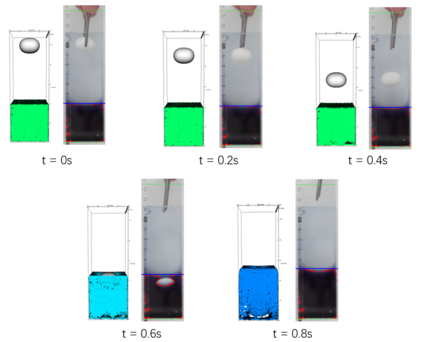
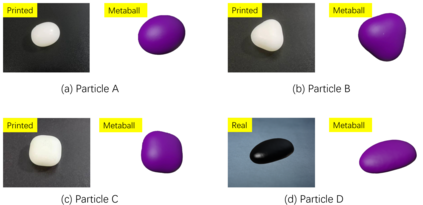
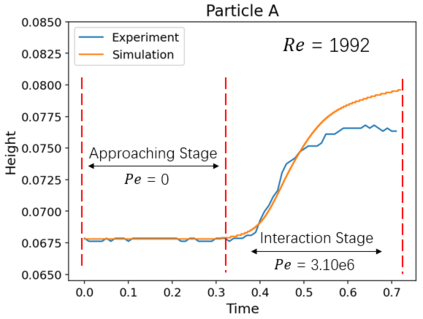
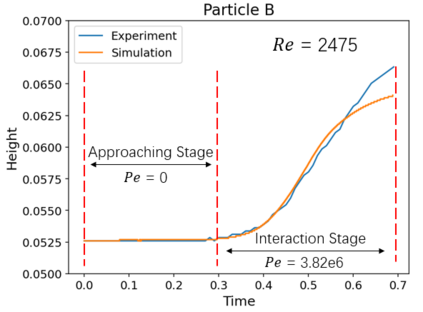
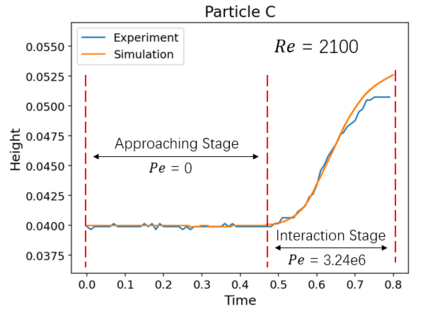
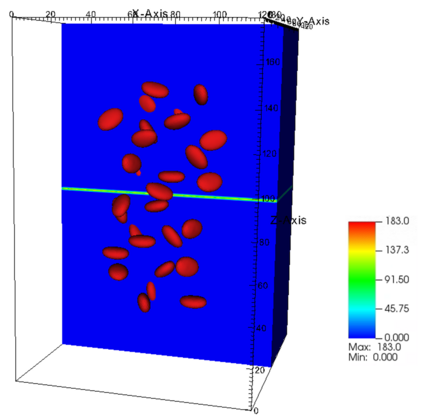
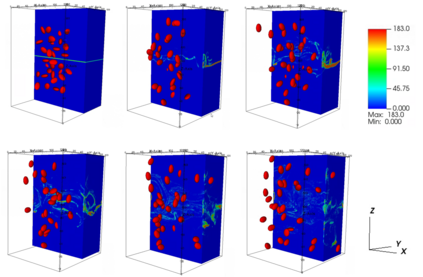
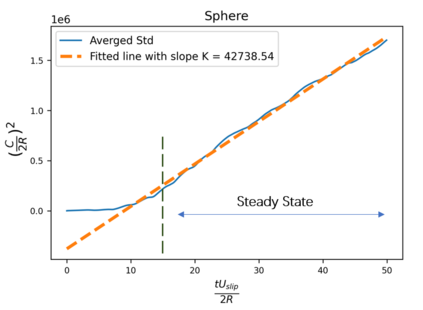
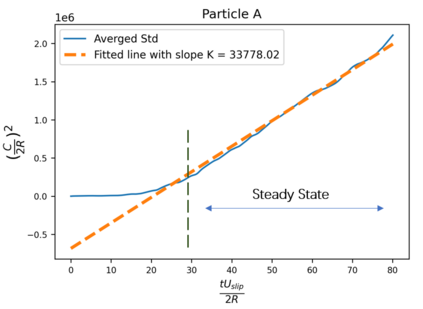
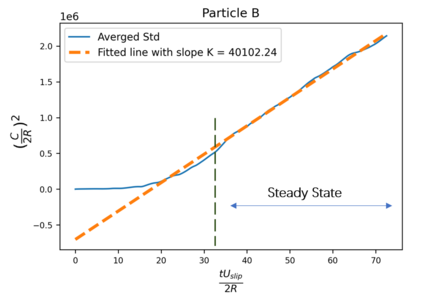
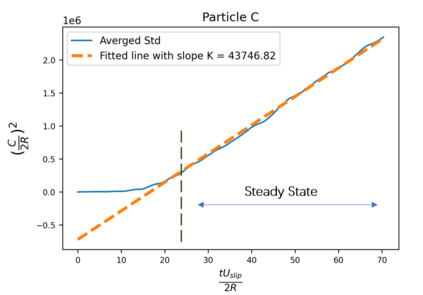
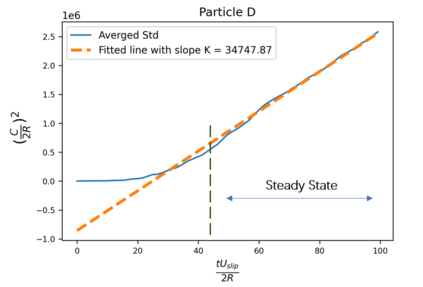
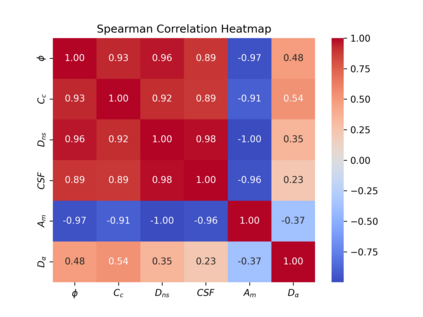
Solute transport in fluid-particle systems is a fundamental process in numerous scientific and engineering disciplines. The simulation of it necessitates the consideration of solid particles with intricate shapes and sizes. To address this challenge, this study proposes the Random-Walk Metaball-Imaging Discrete Element Lattice Boltzmann Method (RW-MI-DELBM). In this model, we reconstruct particle geometries with the Metaball-Imaging algorithm, capture the particle behavior using the Discrete Element Method (DEM), simulate fluid behavior by the Lattice Boltzmann Method (LBM), and represent solute behavior through the Random Walk Method (RWM). Through the integration of these techniques with specially designed boundary conditions, we achieve to simulate the solute transport in fluid-particle systems comprising complex particle morphologies. Thorough validations, including analytical soluutions and experiments, are performed to assess the robustness and accuracy of this framework. The results demonstrate that the proposed framework can accurately capture the complex dynamics of solute transport under strict mass conservation. In particular, an investigation is carried out to assess the influence of particle morphologies on solute transport in a 3D oscillator, with a focus on identifying correlations between shape features and dispersion coefficients. Notably, all selected shape features exhibited strong correlations with the dispersion coefficient, indicating the significant influence of particle shapes on transport phenomena. However, due to the complexity of the relationship and the limited number of simulations, no clear patterns could be observed. Further comprehensive analyses incorporating a broader range of shape features and varying conditions are necessary to fully comprehend their collective influence on the dispersion coefficient.
翻译:暂无翻译