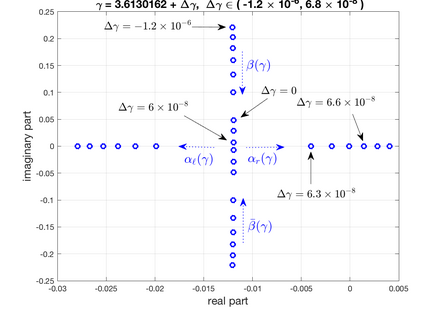
This paper focuses on studying the bifurcation analysis of the eigenstructure of the $\gamma$-parameterized generalized eigenvalue problem ($\gamma$-GEP) arising in three-dimensional (3D) source-free Maxwell's equations with Pasteur media, where $\gamma$ is the magnetoelectric chirality parameter. For the weakly coupled case, namely, $\gamma < \gamma_{*} \equiv$ critical value, the $\gamma$-GEP is positive definite, which has been well-studied by Chern et.\ al, 2015. For the strongly coupled case, namely, $\gamma > \gamma_{*}$, the $\gamma$-GEP is no longer positive definite, introducing a totally different and complicated structure. For the critical strongly coupled case, numerical computations for electromagnetic fields have been presented by Huang et.\ al, 2018. In this paper, we build several theoretical results on the eigenstructure behavior of the $\gamma$-GEPs. We prove that the $\gamma$-GEP is regular for any $\gamma > 0$, and the $\gamma$-GEP has $2 \times 2$ Jordan blocks of infinite eigenvalues at the critical value $\gamma_{*}$. Then, we show that the $2 \times 2$ Jordan block will split into a complex conjugate eigenvalue pair that rapidly goes down and up and then collides at some real point near the origin. Next, it will bifurcate into two real eigenvalues, with one moving toward the left and the other to the right along the real axis as $\gamma$ increases. A newly formed state whose energy is smaller than the ground state can be created as $\gamma$ is larger than the critical value. This stunning feature of the physical phenomenon would be very helpful in practical applications. Therefore, the purpose of this paper is to clarify the corresponding theoretical eigenstructure of 3D Maxwell's equations with Pasteur media.
翻译:本文侧重于研究对美元=gamma$- 参数化通用乙基值($gamma$- GEP) 在三维(3D) 无源的Maxwell 的方程式中, $\gamma$是磁电动手风力参数。 相弱结合的个案, 即 $\ gamma < gamma\\\\\\ equev$ 关键值, $gamma- GEP是肯定的。 美元=gma- gP是肯定的。 美元=g- gmape值在三维( 3D) 中, 美元=GEGEP 在纸质化的快速分析中, 美元=creal- mamamaial $; 美元= 美元=qualmamamax 。 美元= = 美元= 美元= 美元=xxxx。 美元= 美元= 美元= 美元= 美元=xgmamamamama = = = = = 美元= 美元=xxx =xxxxxxxxxxx =x =xx =xxx =xxxxxxxxxxxxxxxxxxxxxxxxx =xxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxxx