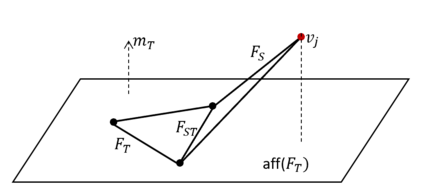
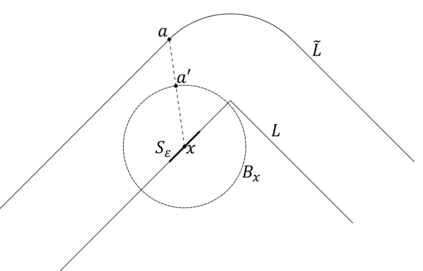
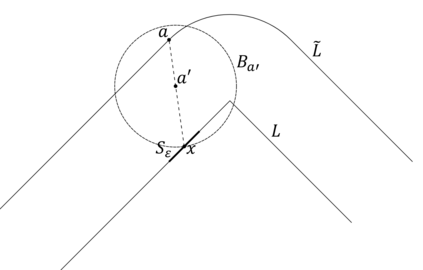
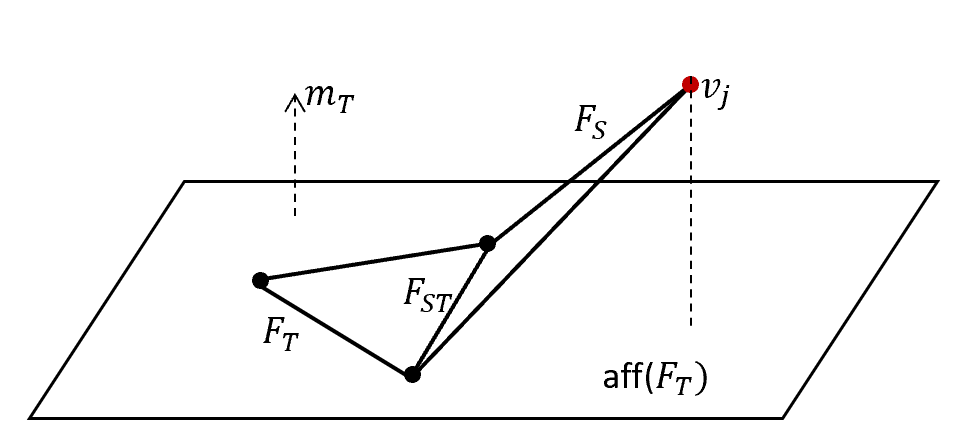
We prove upper bounds on the graph diameters of polytopes in two settings. The first is a worst-case bound for polytopes defined by integer constraints in terms of the height of the integers and certain subdeterminants of the constraint matrix, which in some cases improves previously known results. The second is a smoothed analysis bound: given an appropriately normalized polytope, we add small Gaussian noise to each constraint. We consider a natural geometric measure on the vertices of the perturbed polytope (corresponding to the mean curvature measure of its polar) and show that with high probability there exists a "giant component" of vertices, with measure $1-o(1)$ and polynomial diameter. Both bounds rely on spectral gaps -- of a certain Schr\"odinger operator in the first case, and a certain continuous time Markov chain in the second -- which arise from the log-concavity of the volume of a simple polytope in terms of its slack variables.
翻译:在两个设置中,我们证明多元托盘的图形直径为上界。 首先是对以整数限制定义的多元托盘最坏的框框, 以整数限制的高度和约束矩阵的某些子确定性来界定, 在某些情况下, 这会改善先前已知的结果。 第二个是平滑的分析 : 如果有一个适当标准化的多色谱, 我们在每个限制中添加小高斯音。 我们考虑在环形聚点的顶部( 与极点的平均曲线测量值相对应) 上进行自然几何测量, 并显示在非常可能的情况下, 存在一个有1°(1)美元和多色直径的脊椎的“ 巨形组成部分 ” 。 两者都依赖于光谱的缺口 -- 在第一个案例中, 一个特定的Schr\" ocinger运算器操作员, 在第二个限制中, 以及一个持续的时间 Markov 链 -- 这是由于一个简单的多色的量的软质变量的正对调而产生的。