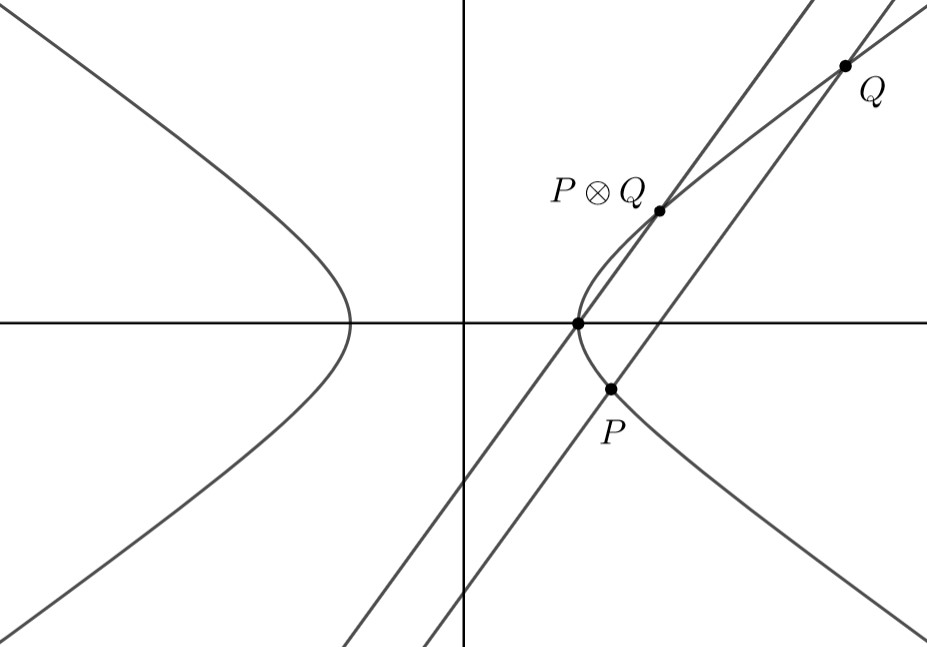
We present a study on the use of Pell hyperbolas in cryptosystems with security based on the discrete logarithm problem. Specifically, after introducing the group's structure over generalized Pell conics (and also giving the explicit isomorphisms with the classical Pell hyperbolas), we provide a parameterization with both an algebraic and a geometrical approach. The particular parameterization that we propose appears to be useful from a cryptographic point of view because the product that arises over the set of parameters is connected to the R\'edei rational functions, which can be evaluated in a fast way. Thus, we exploit these constructions for defining three different public key cryptosystems based on the ElGamal scheme. We show that the use of our parameterization allows to obtain schemes more efficient than the classical ones based on finite fields.
翻译:我们根据离散对数问题,在加密系统中安全地使用Pell perbolas(Pell perbolas) 。 具体地说, 在将该组的结构置于通用的 Pell 二次曲线之上( 并用古典Pell 双曲线给出明确的等式) 之后, 我们用代数法和几何法提供参数化。 我们建议的特殊参数化从加密角度看似乎是有用的, 因为在一组参数上产生的产品与R\'edei 理性功能相关, 后者可以快速评估。 因此, 我们利用这些构造来定义基于ElGamal 方案的三种不同的公用钥匙加密系统。 我们显示,我们参数化的使用使得获得比基于有限字段的古典系统更有效率的计划。