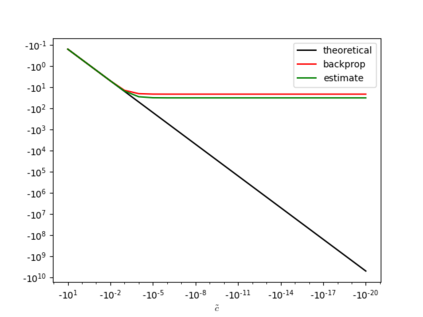
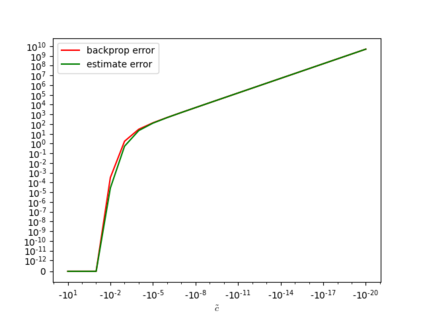
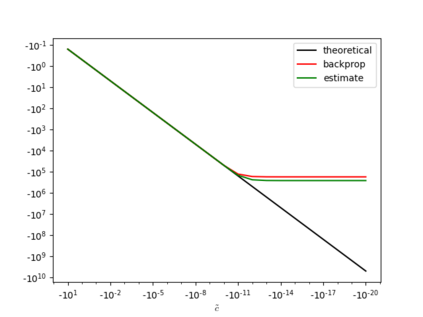
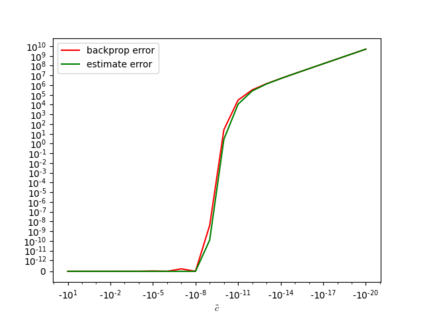
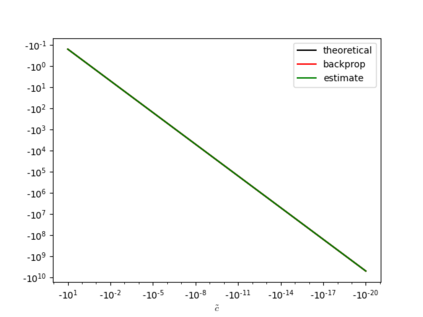
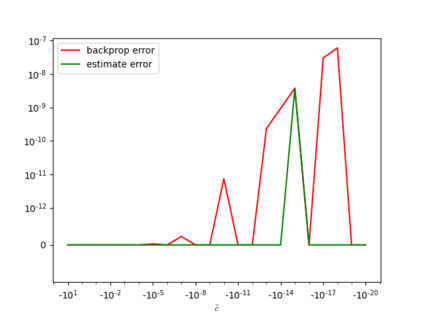
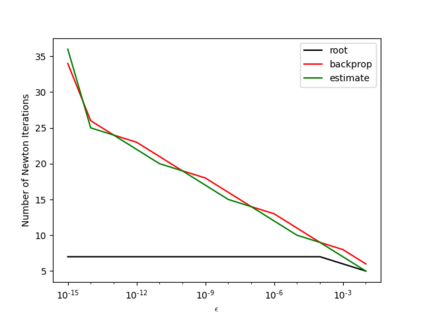
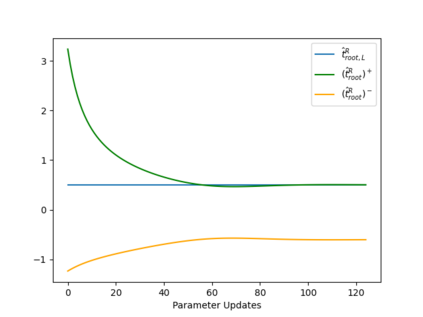
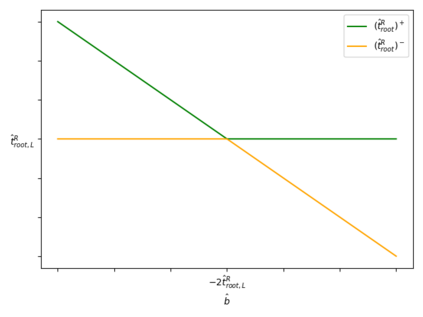
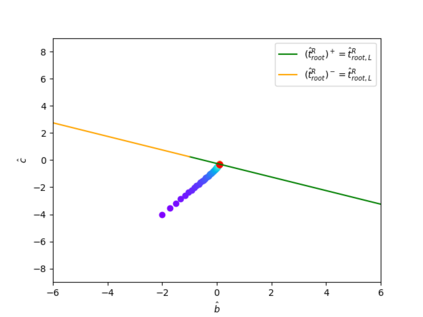
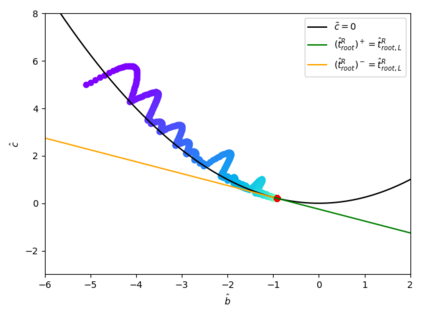
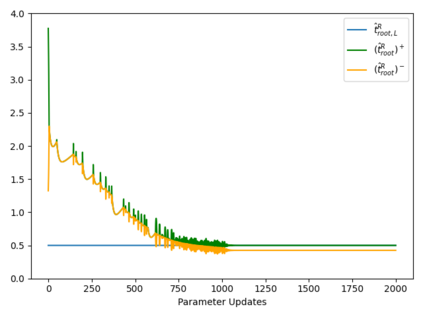
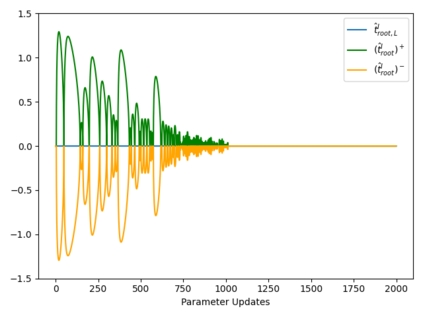
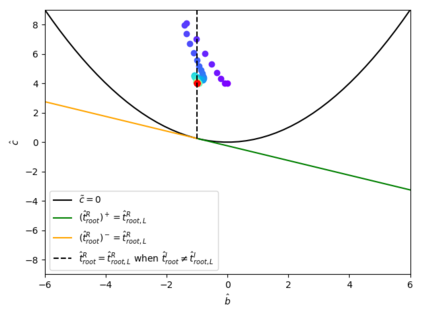
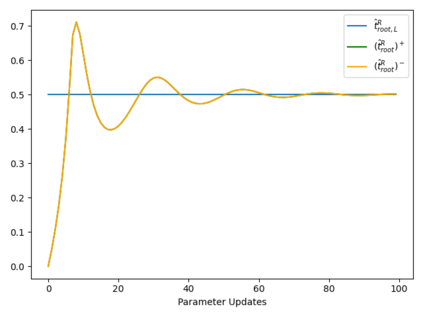
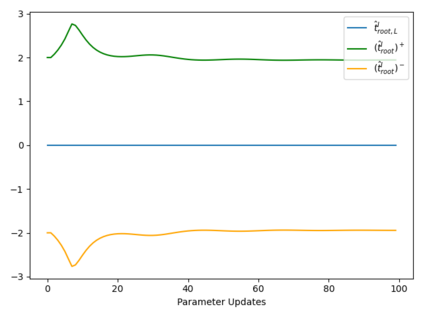
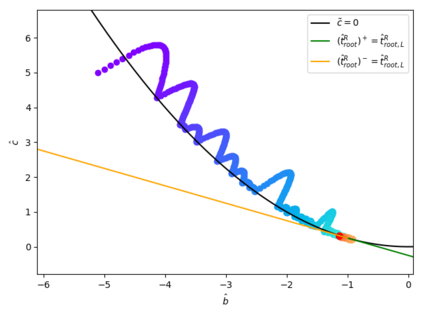
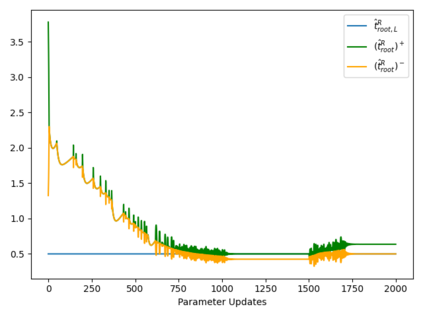
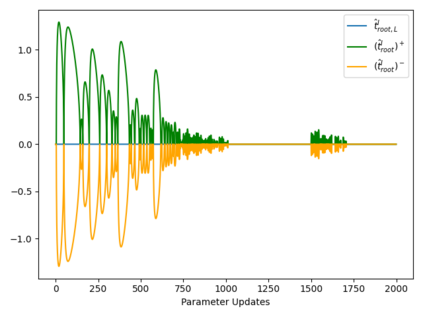
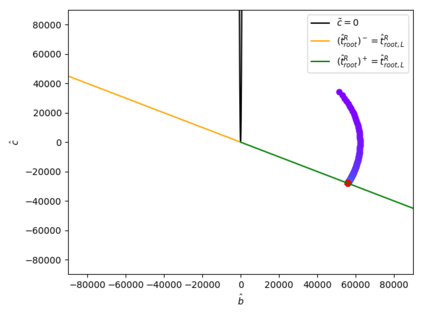
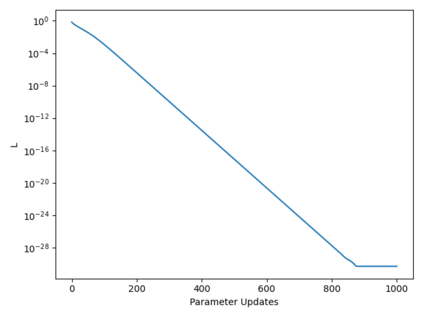
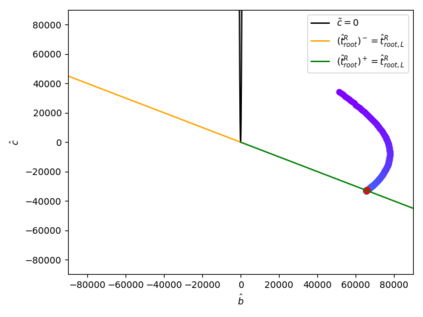
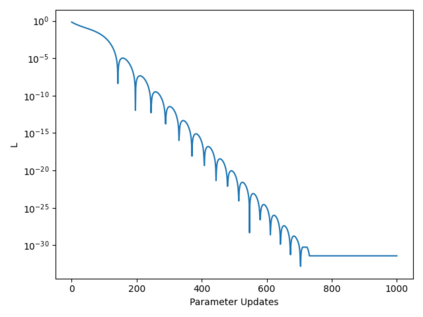
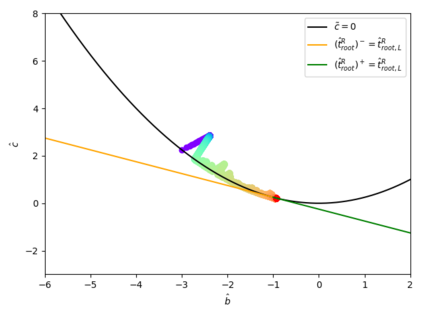
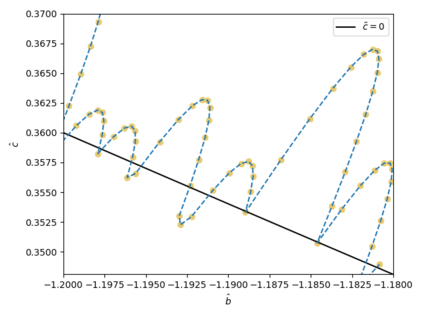
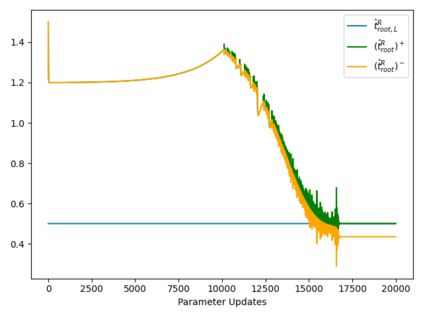
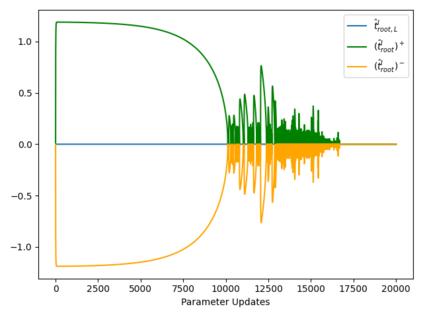
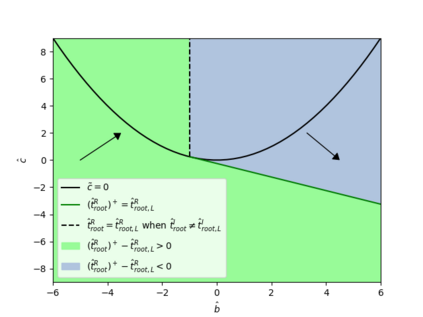
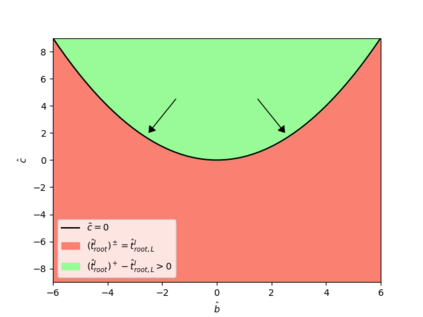
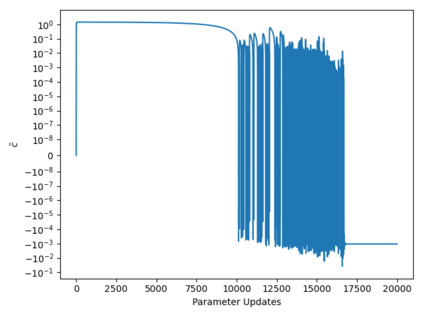
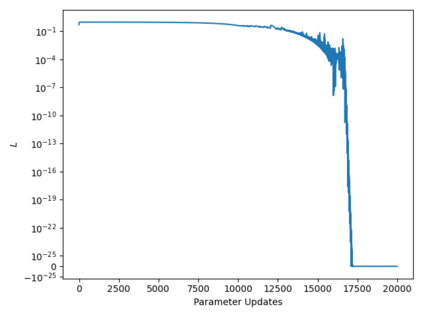
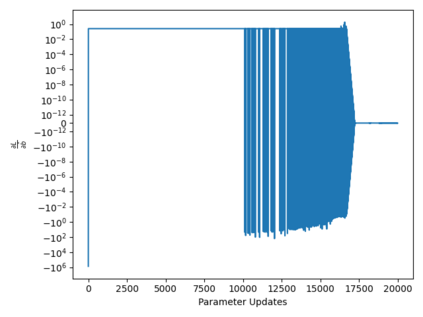
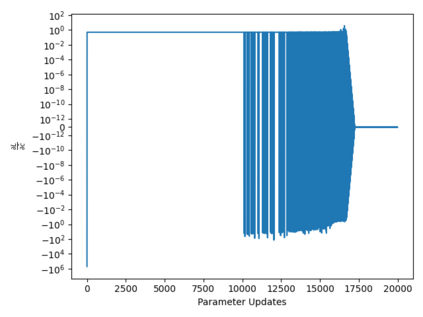
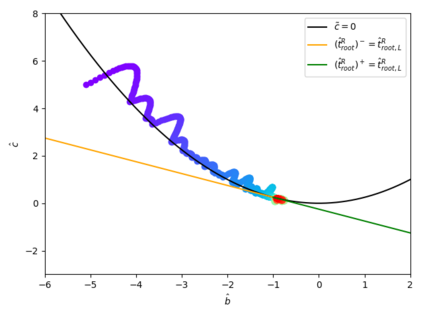
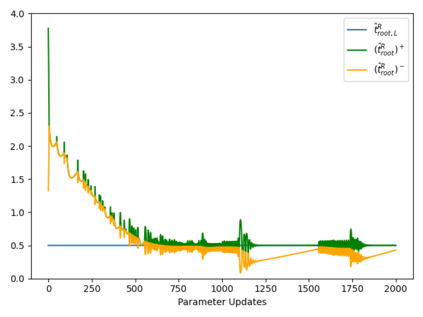
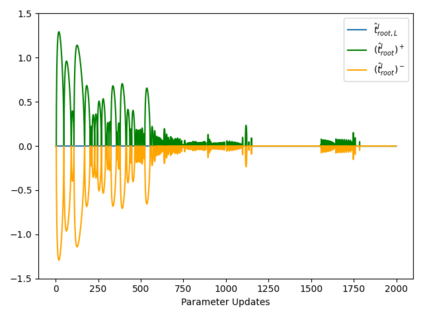
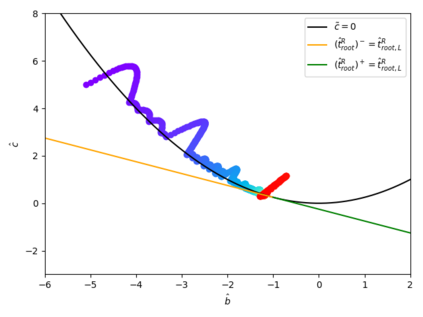
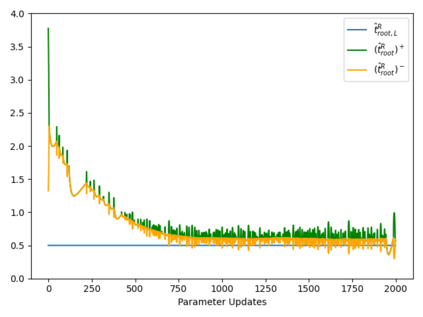
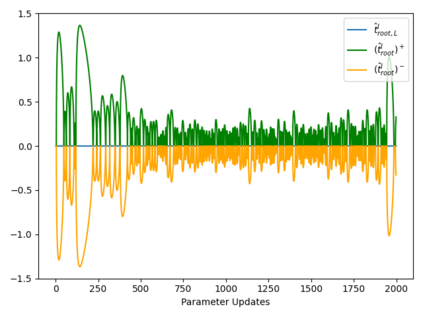
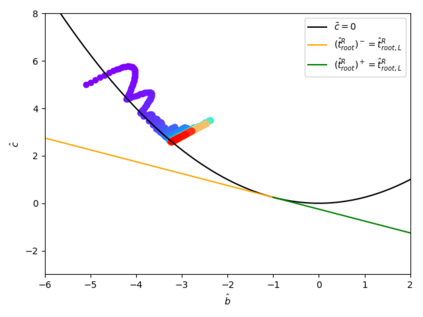
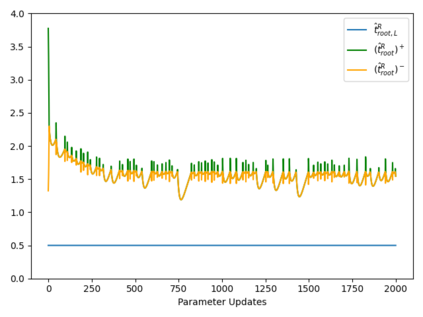
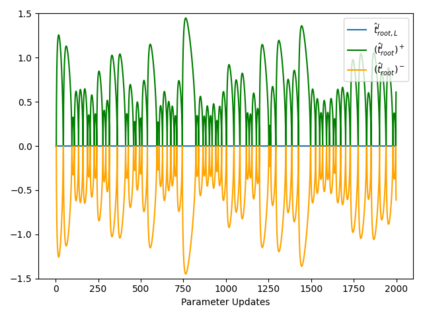
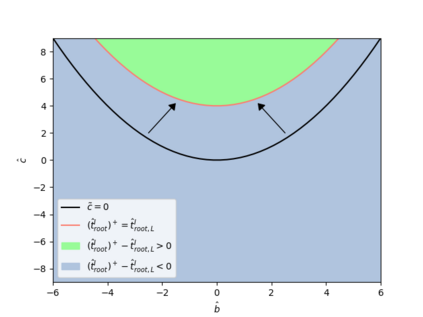
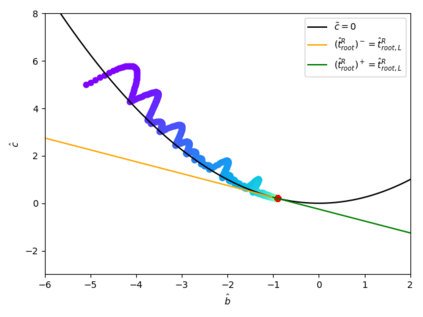
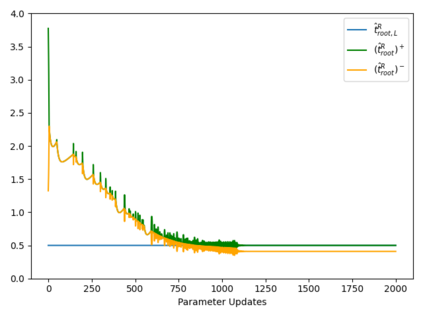
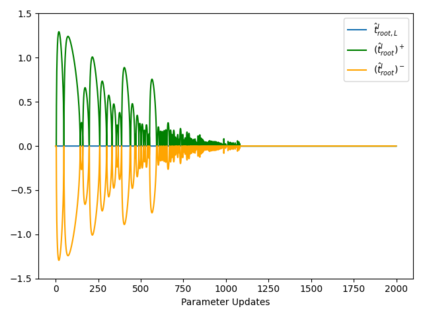
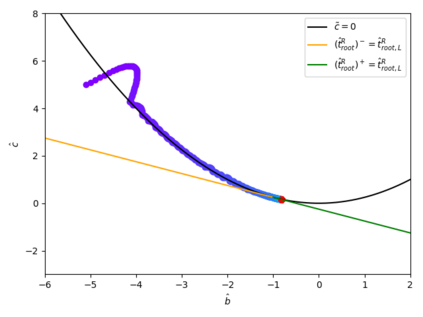
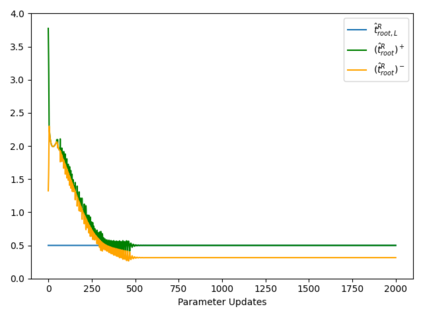
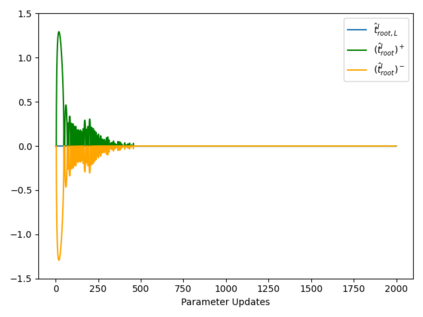
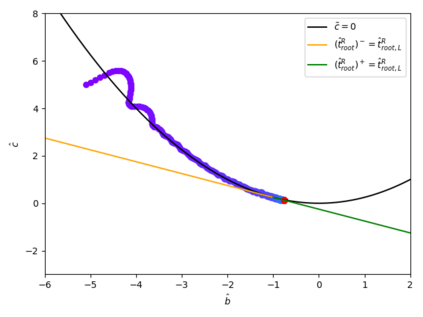
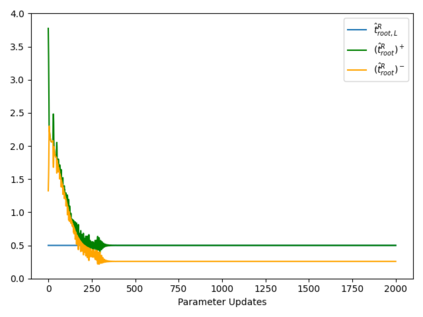
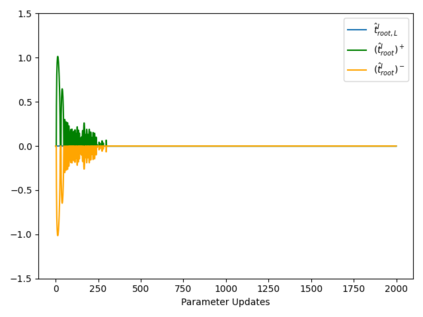
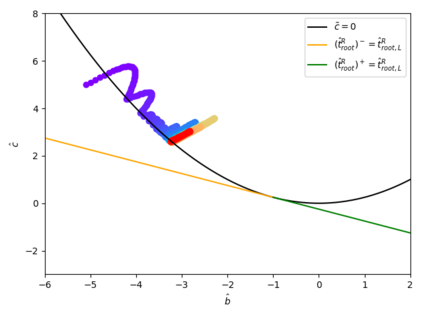
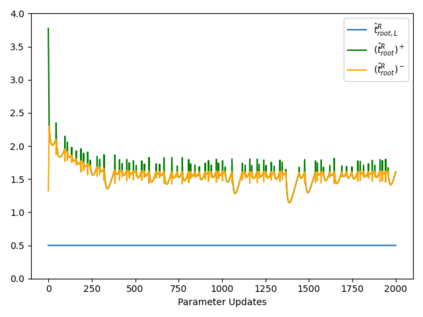
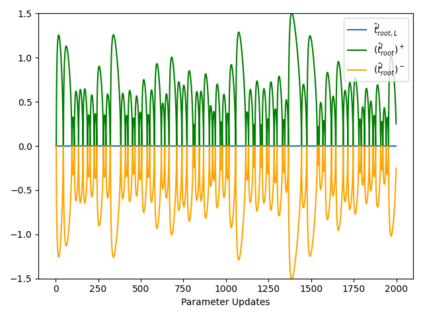
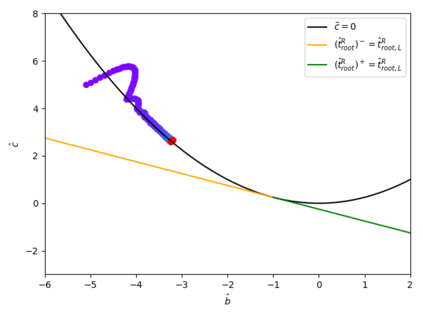
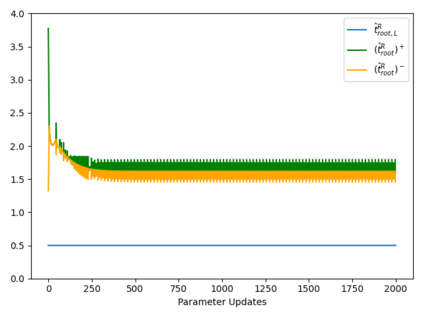
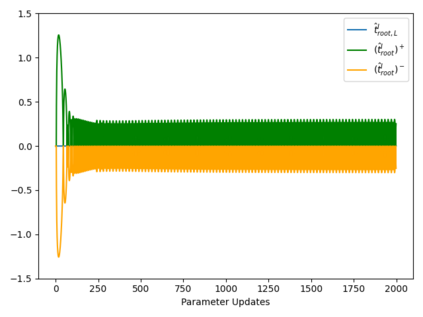
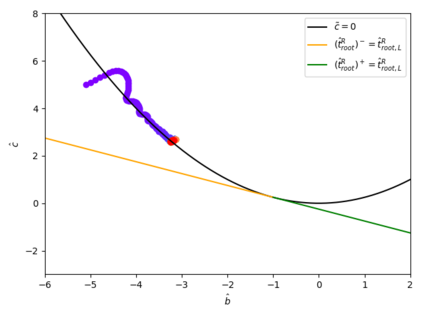
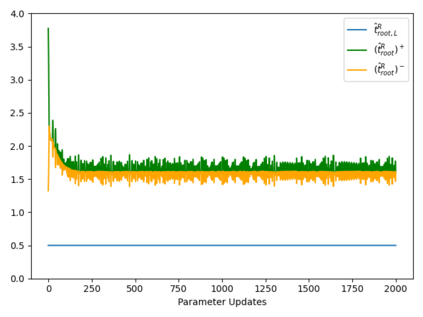
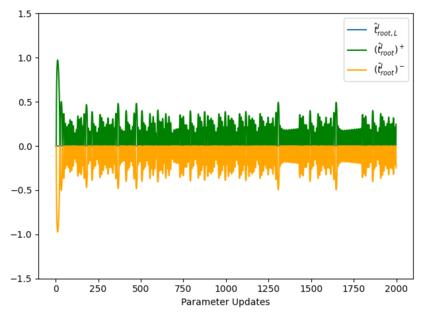
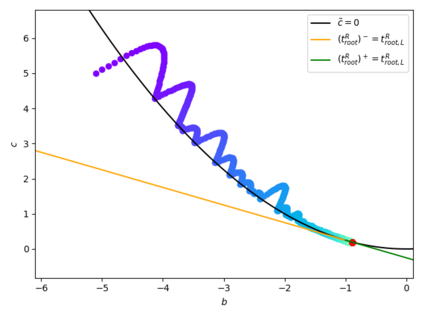
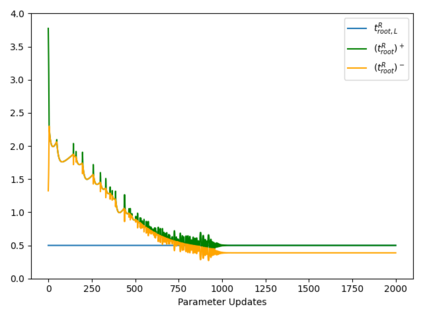
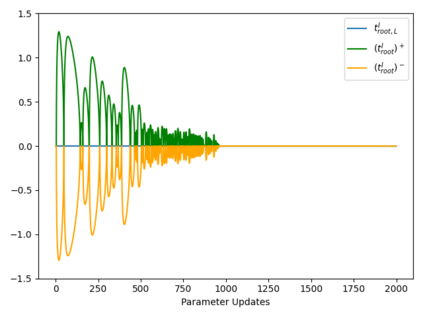
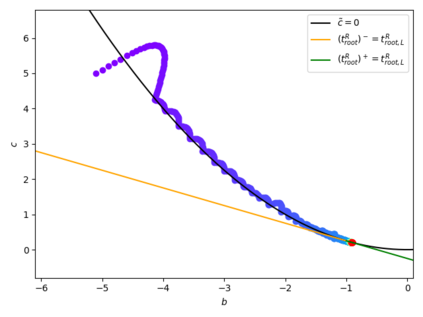
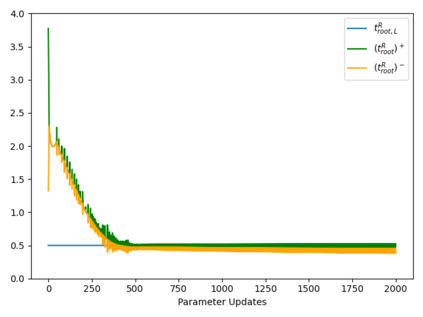
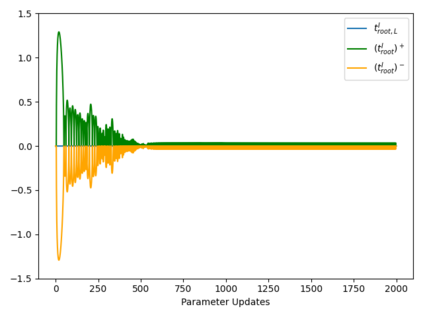
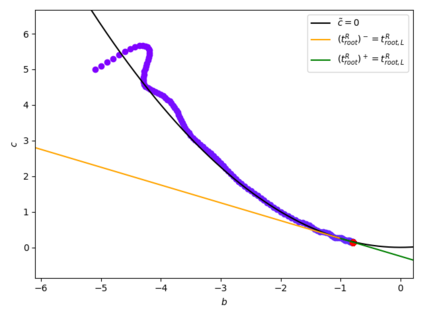
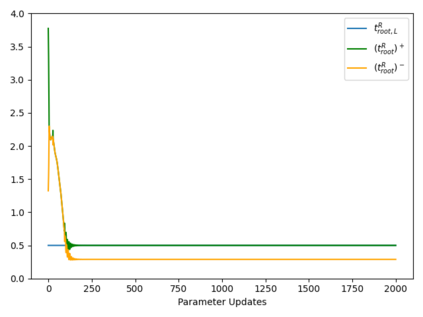
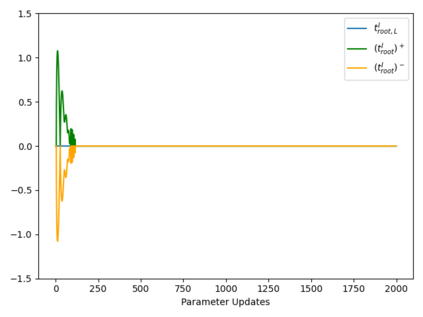
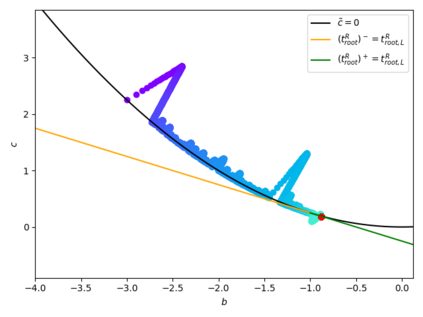
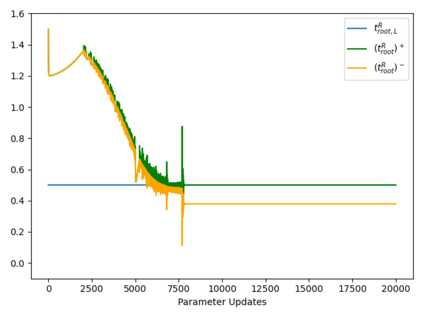
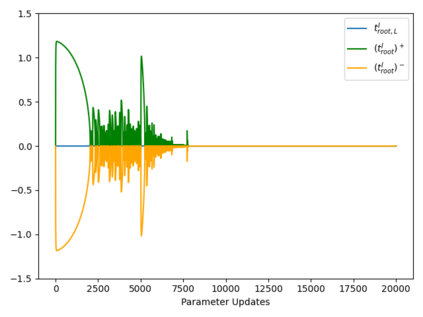
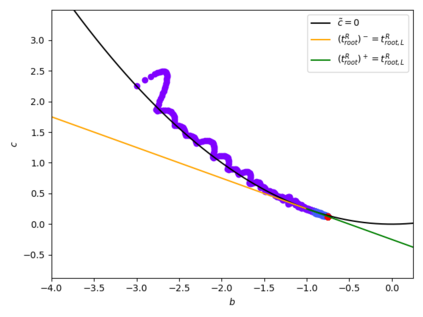
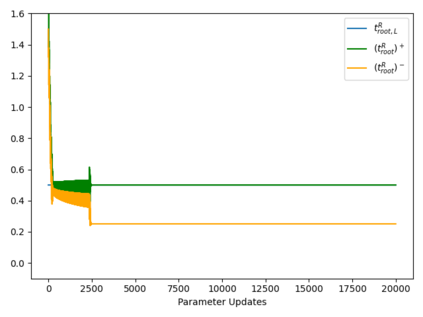
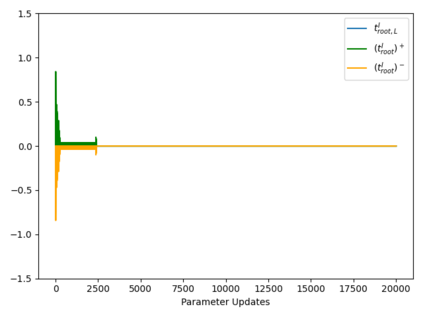
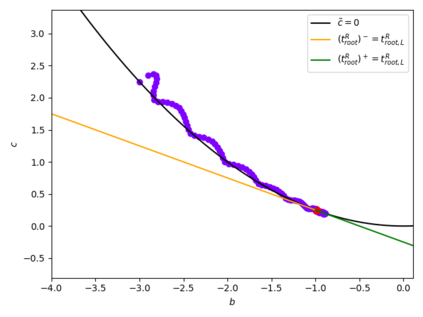
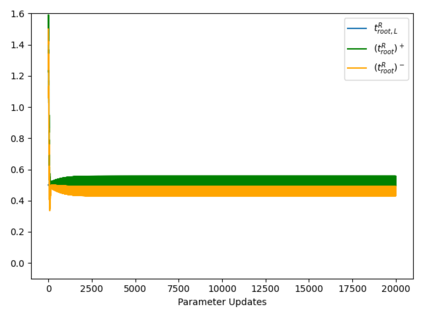
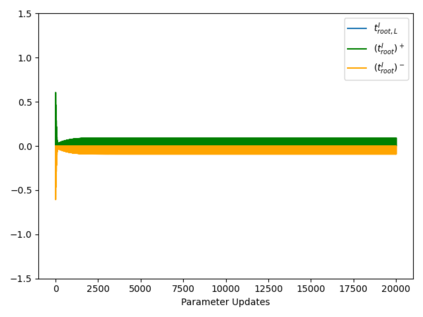
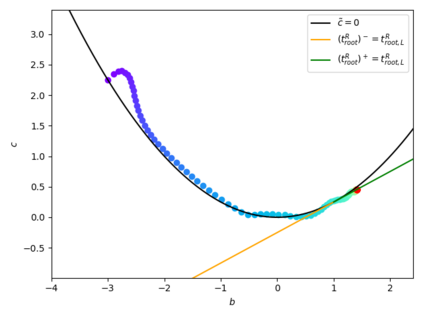
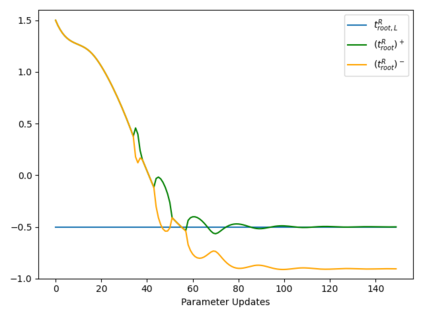
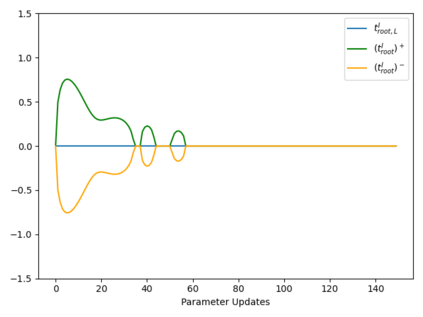
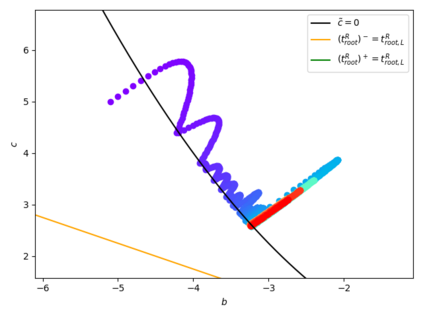
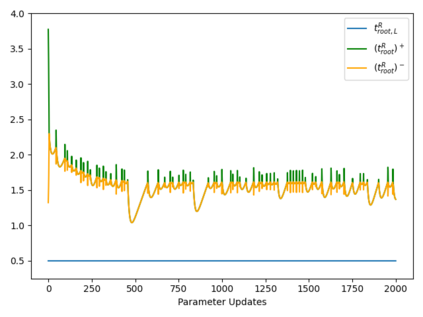
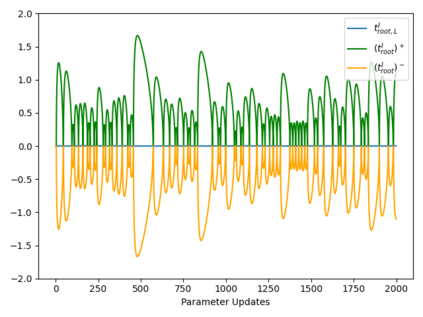
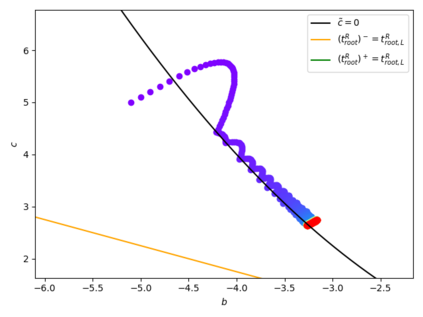
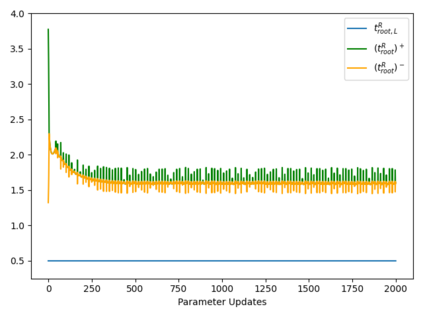
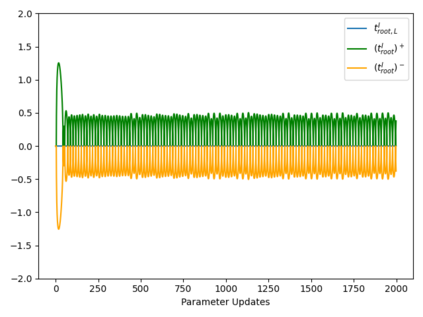
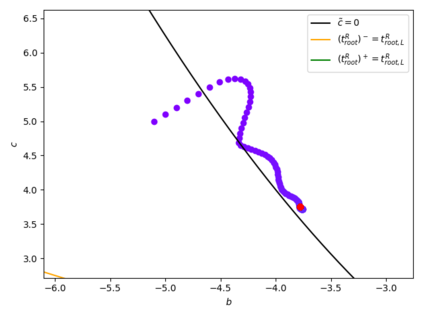
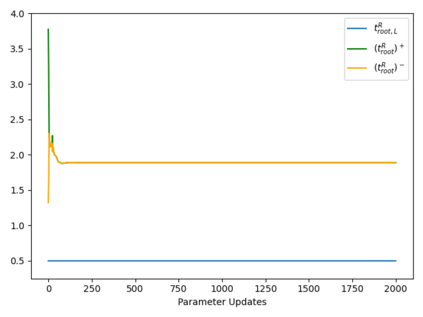
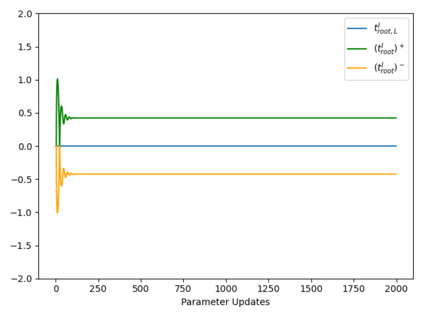
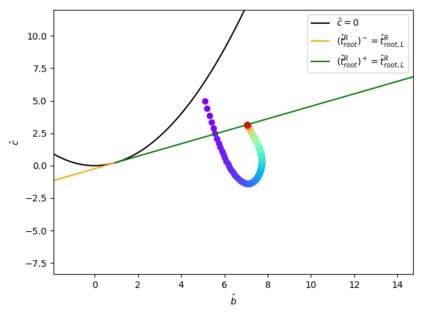
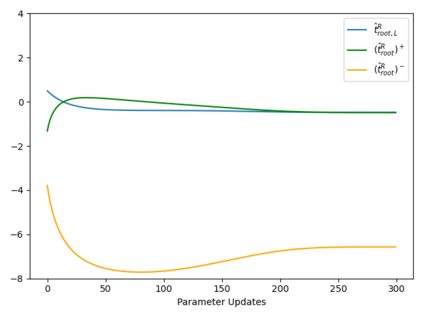
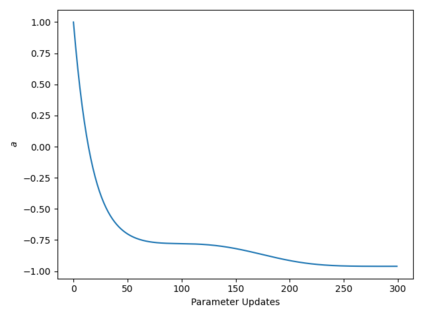
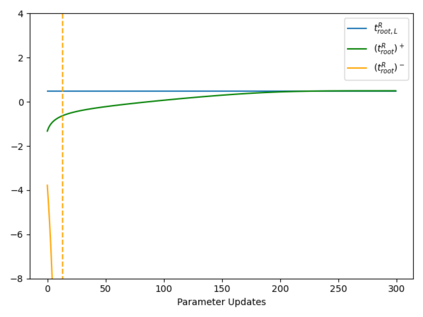
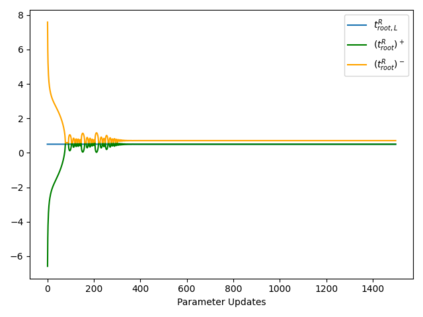
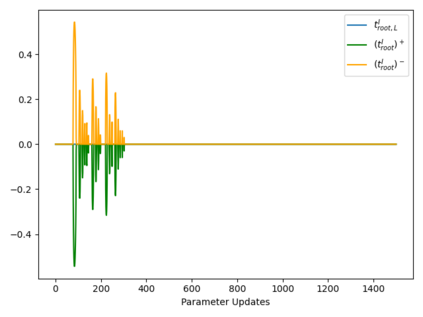
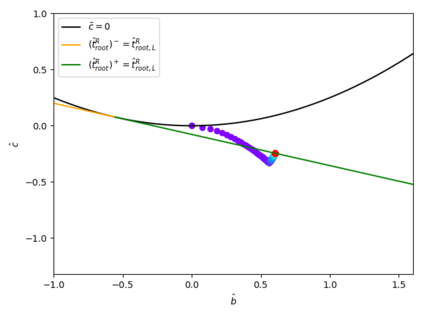
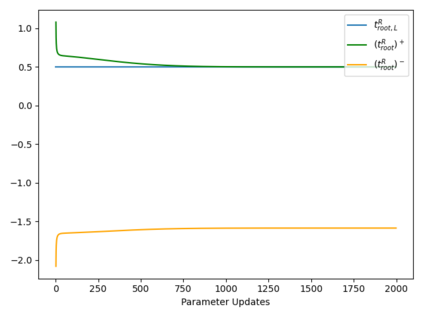
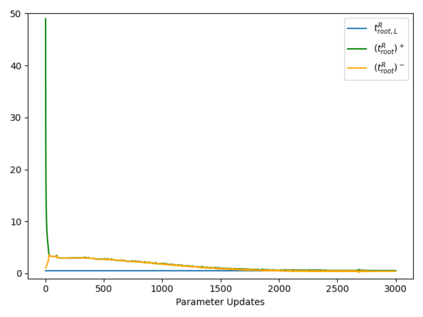
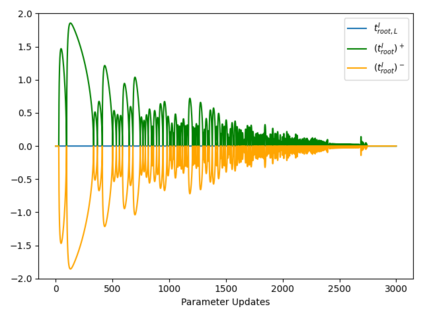
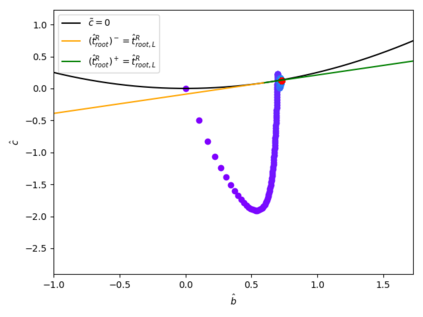
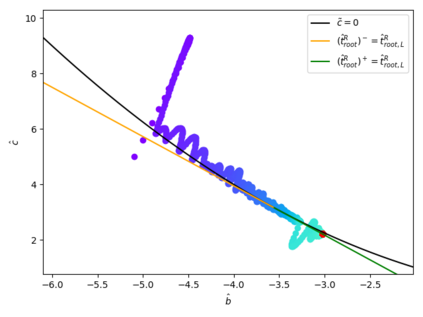
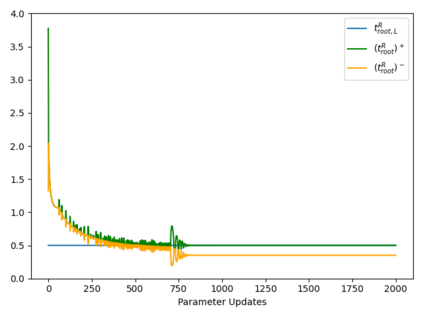
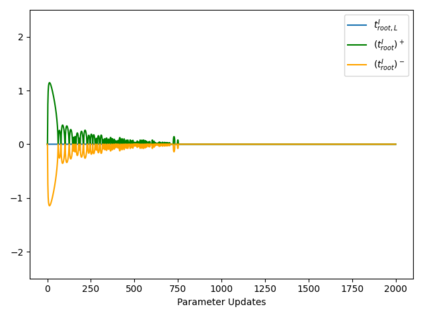
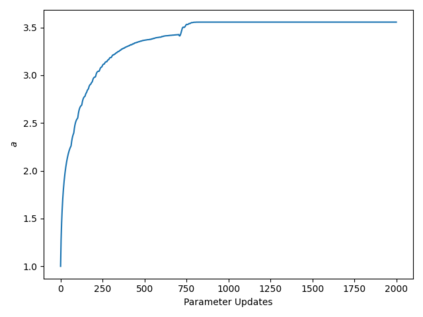
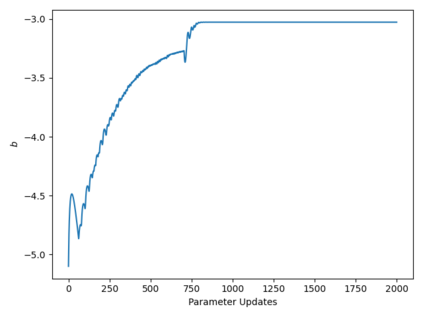
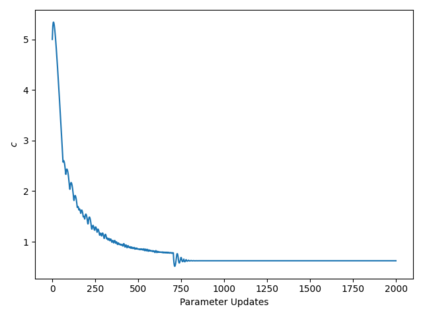
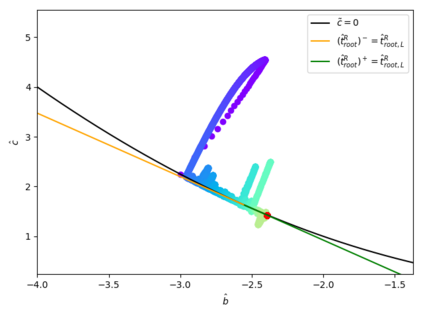
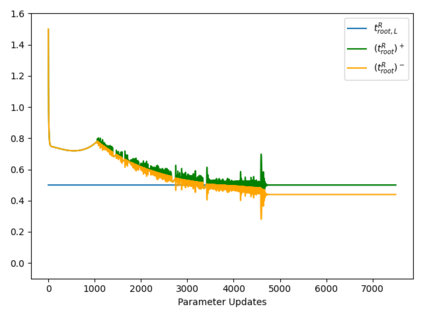
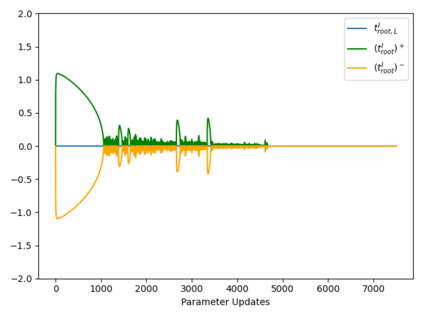
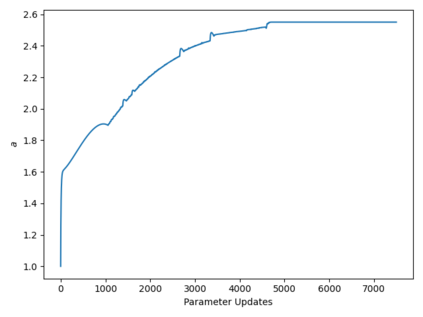
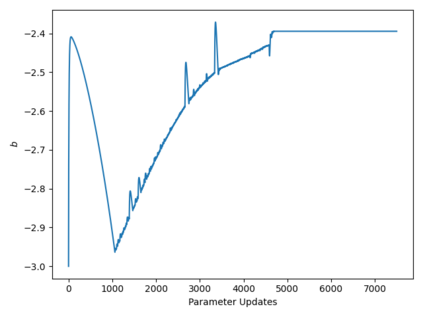
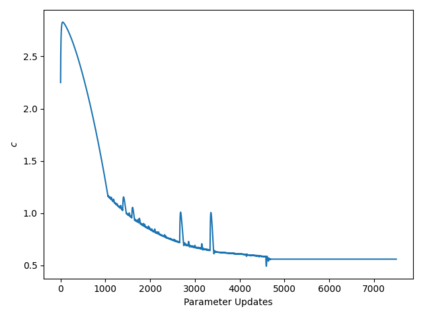
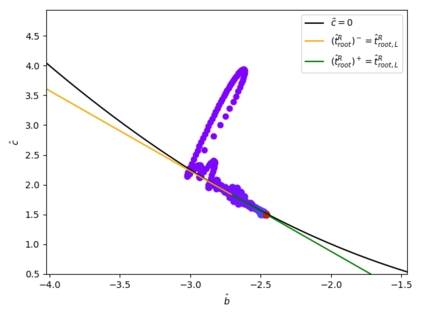
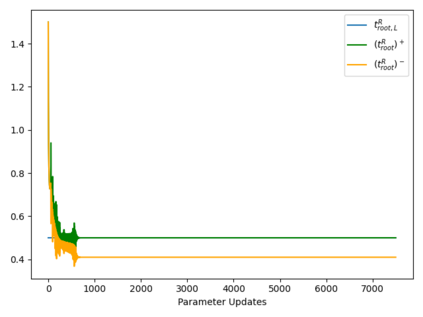
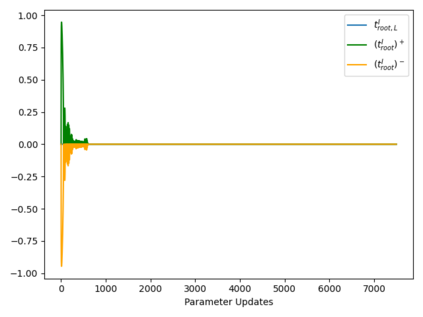
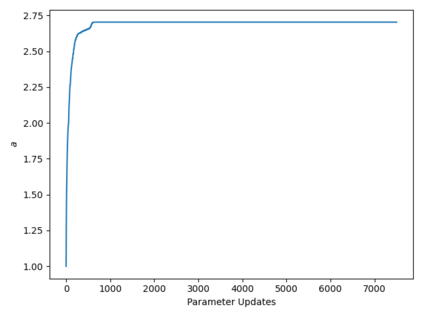
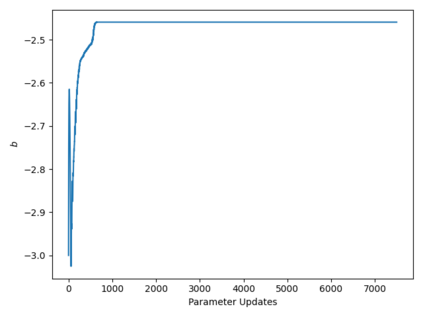
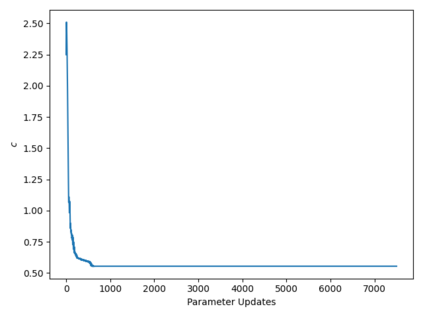
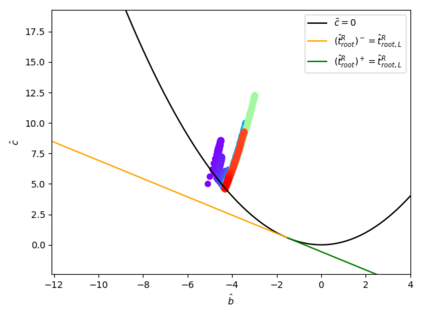
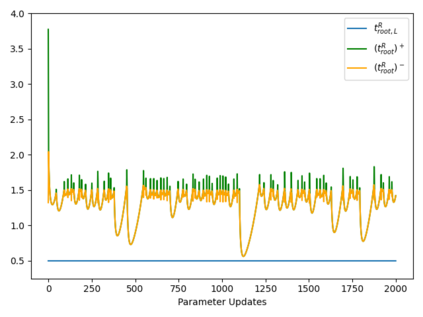
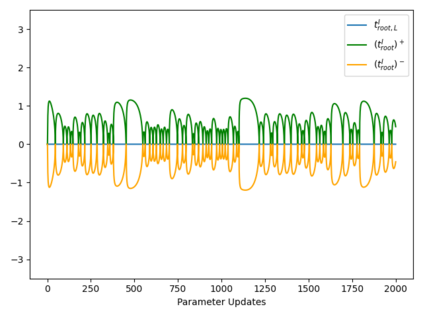
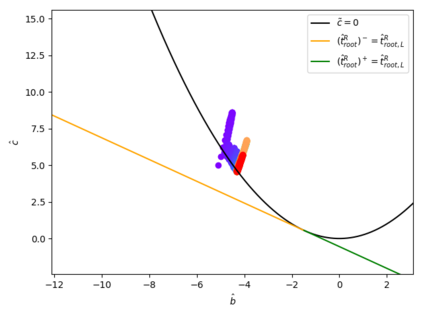
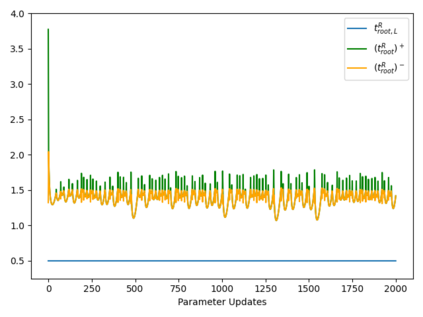
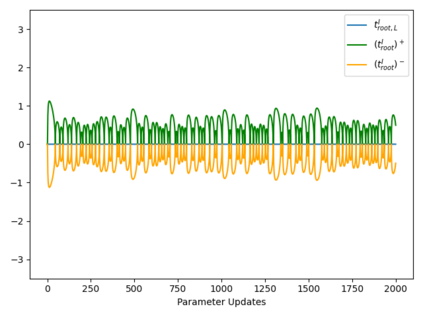
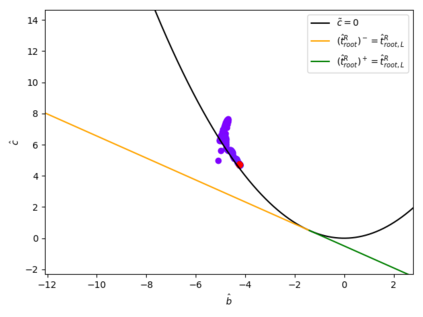
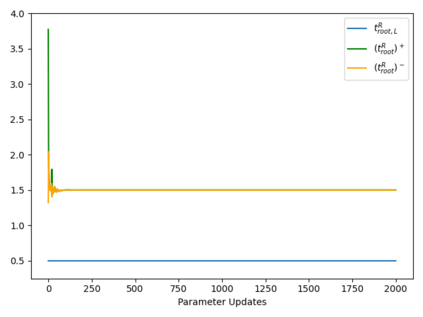
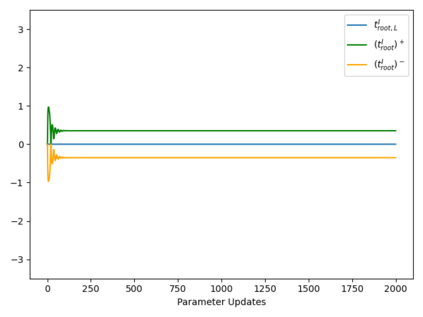
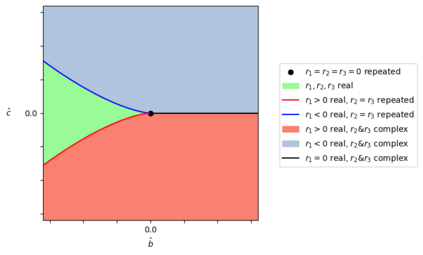
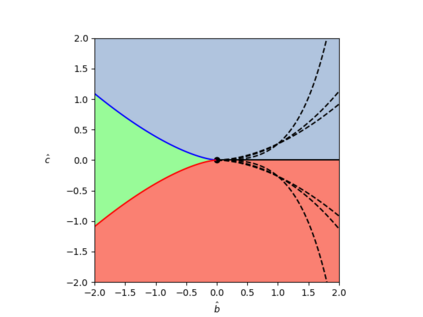
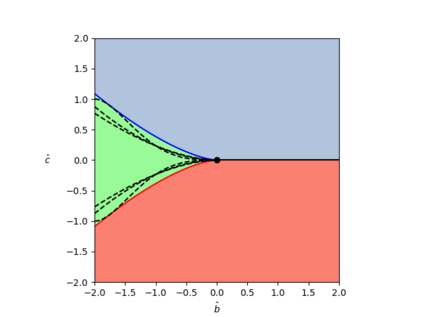
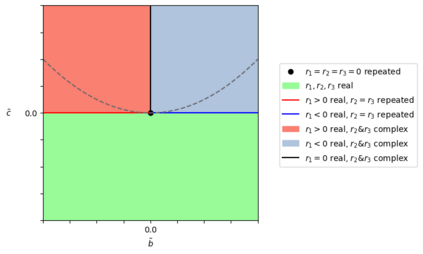
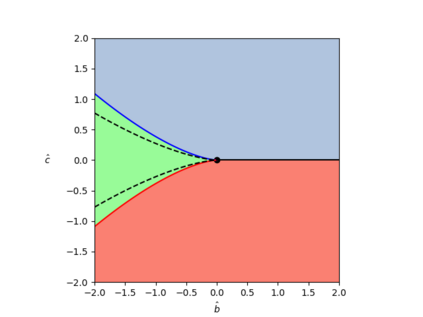
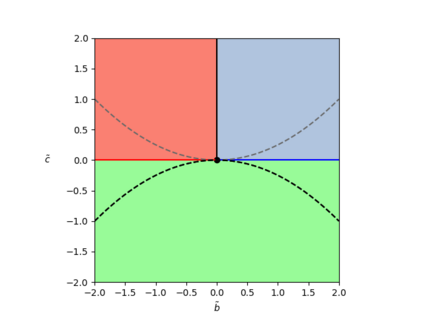
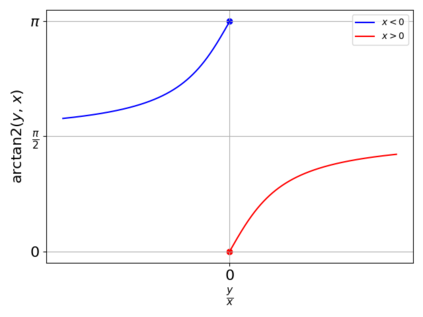
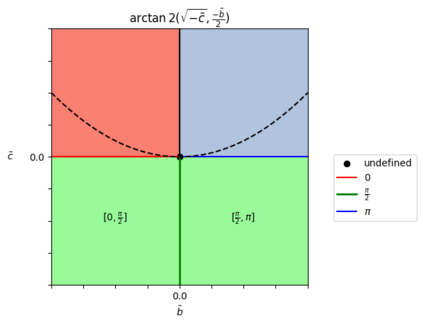
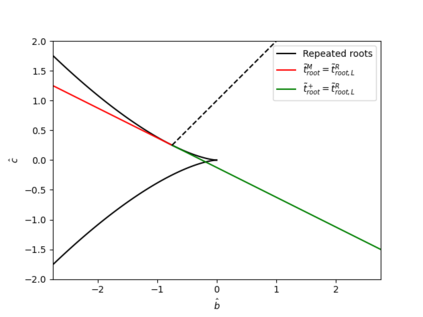
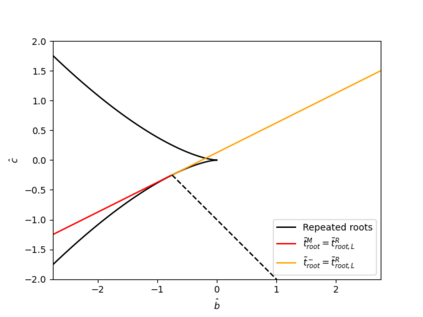
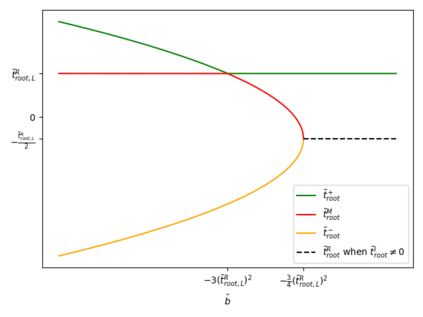
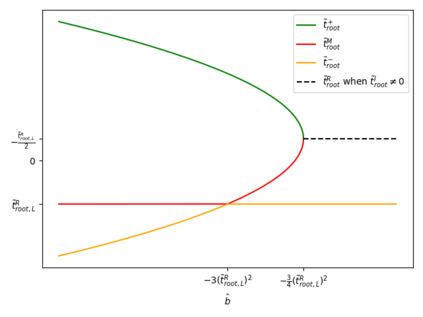
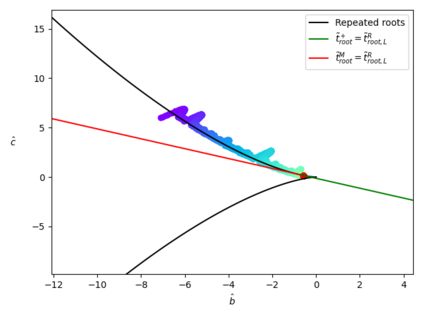
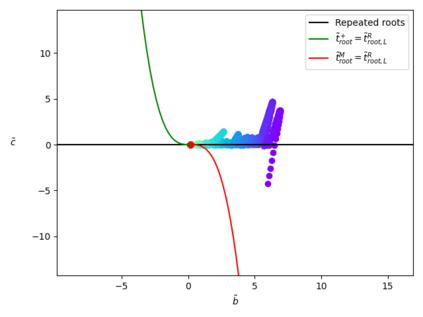
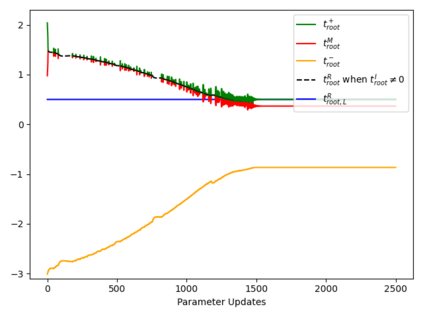
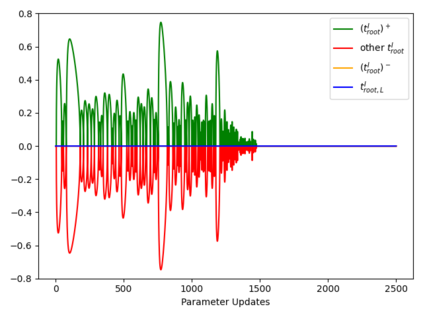
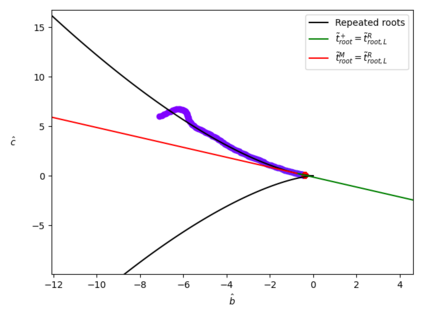
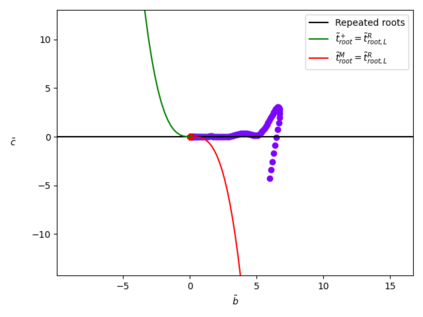
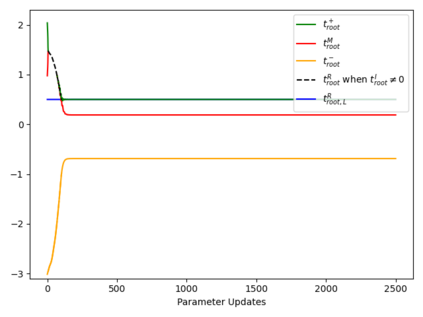
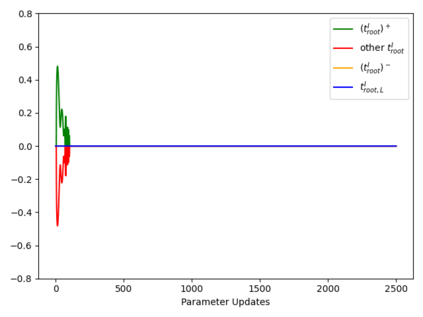
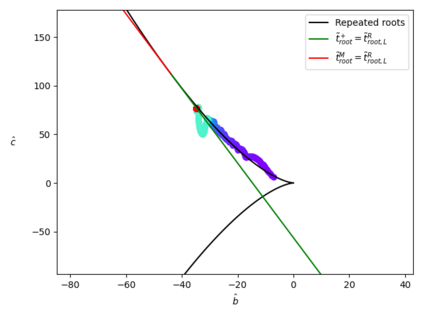
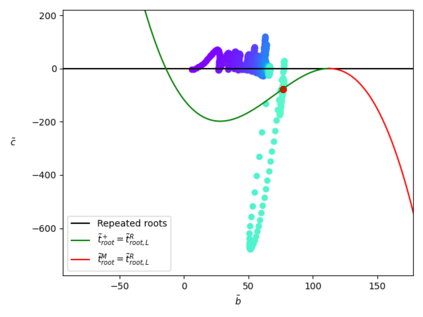
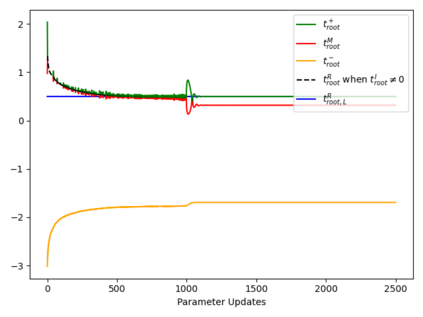
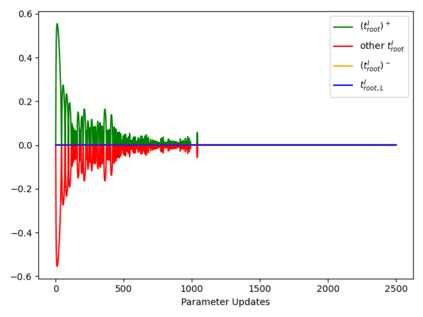
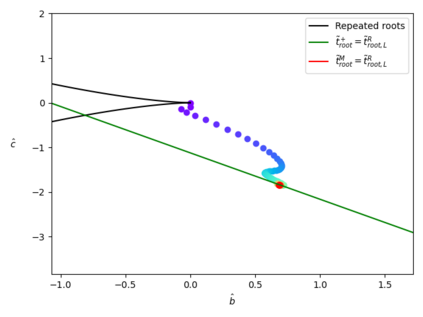
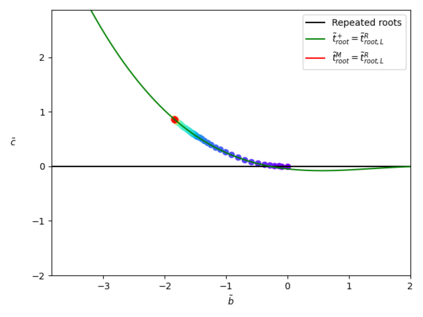
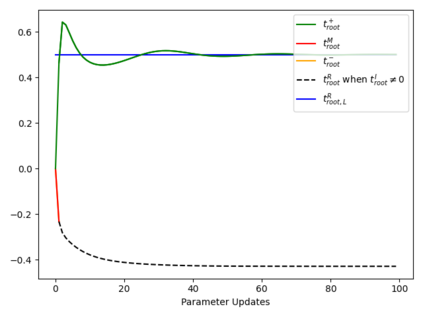
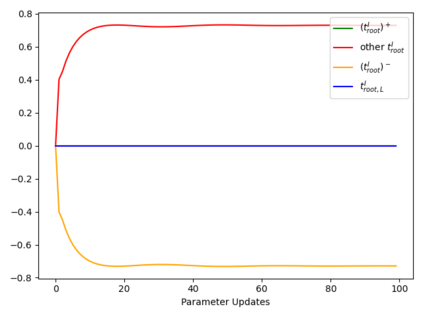
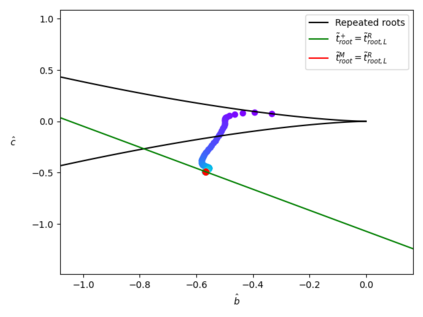
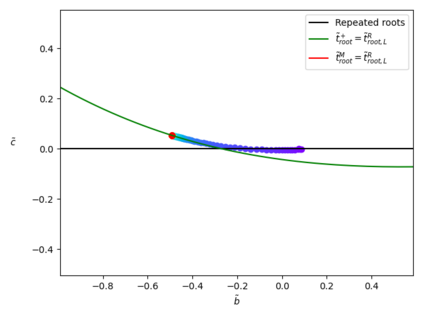
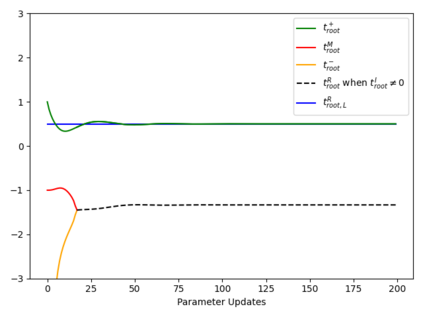
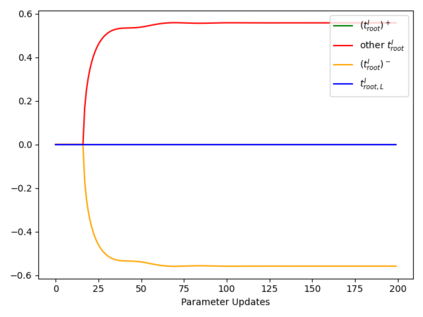
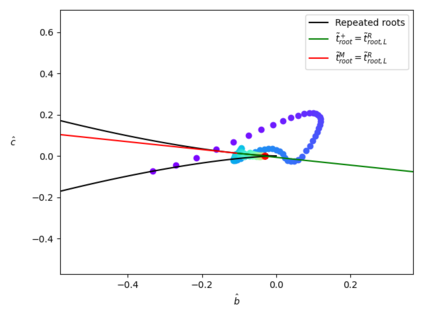
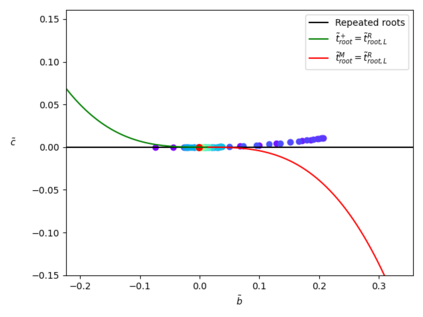
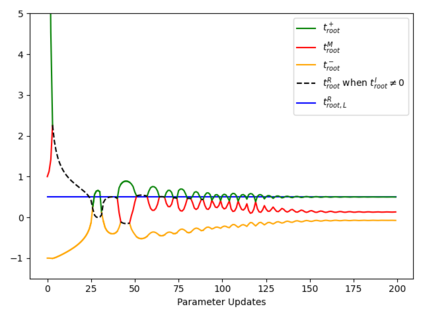
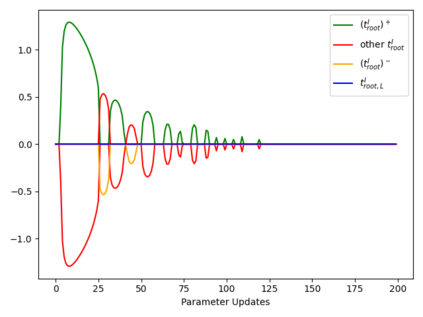
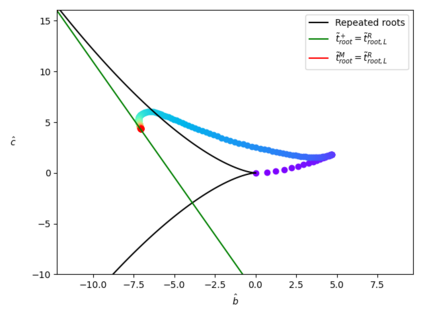
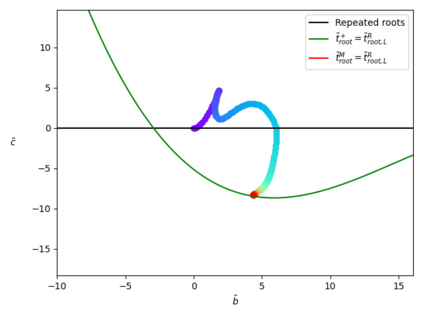
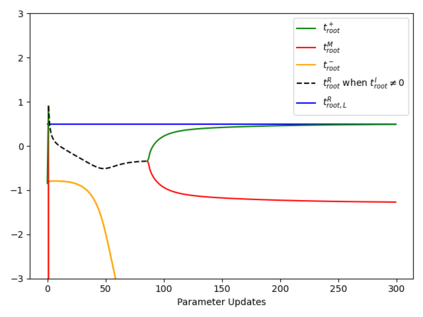
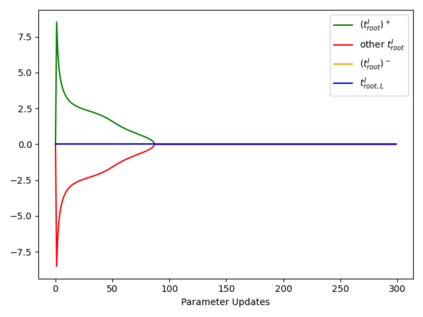
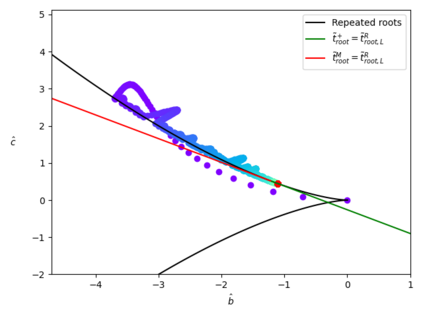
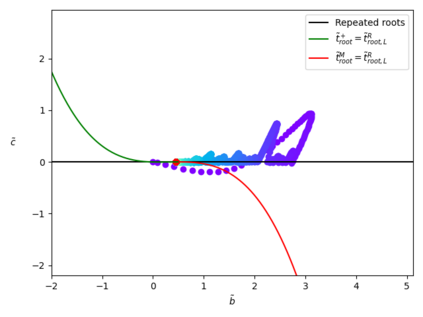
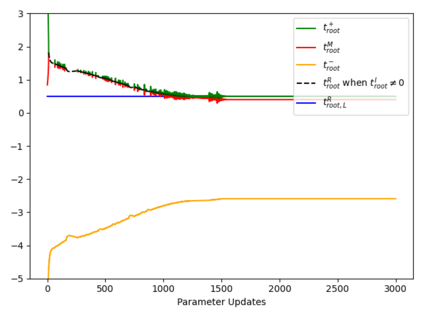
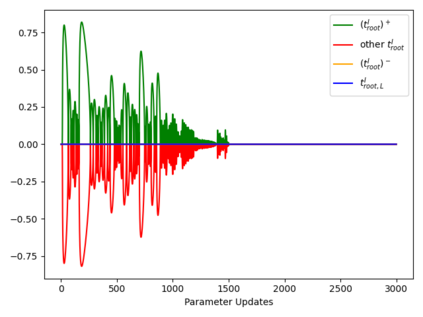
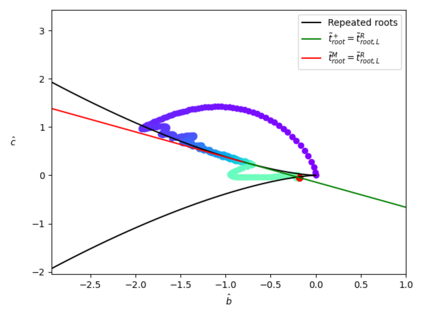
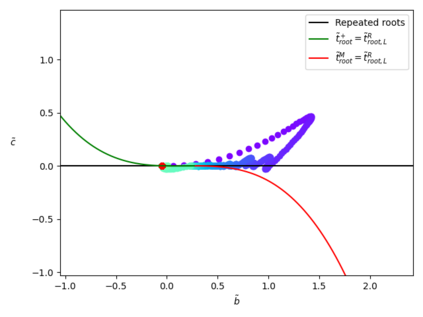
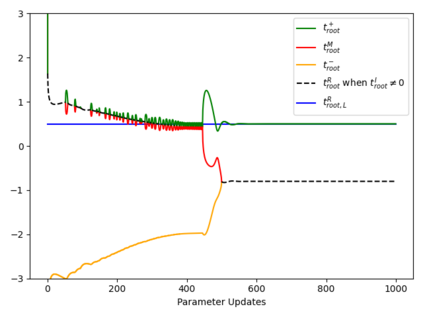
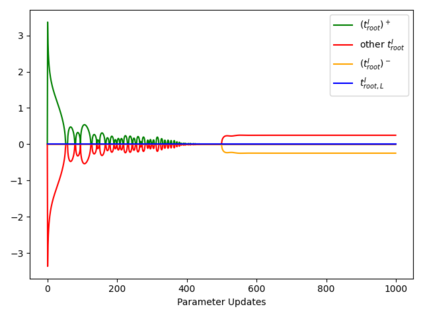
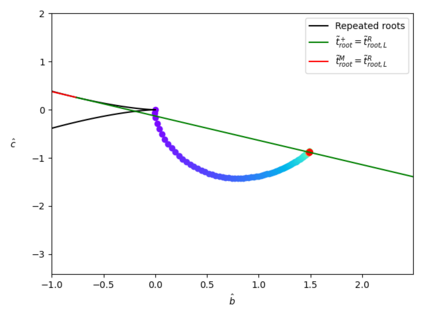
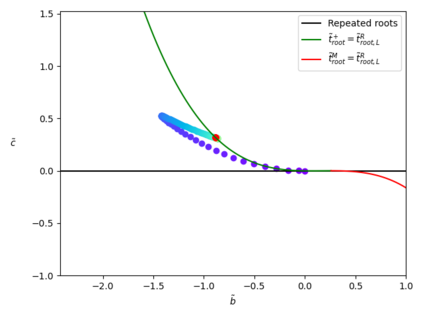
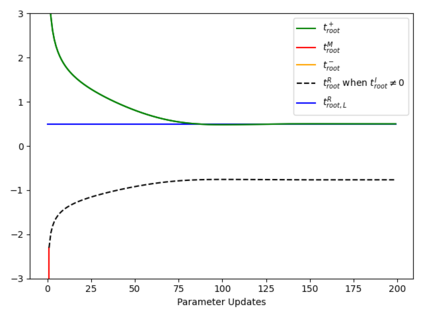
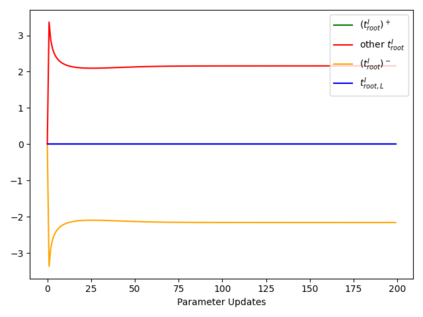
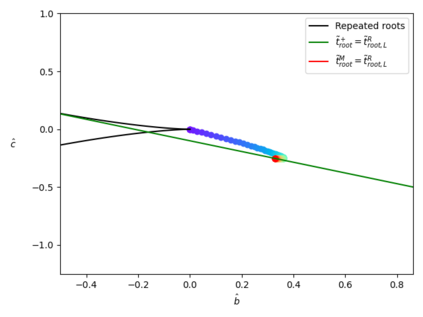
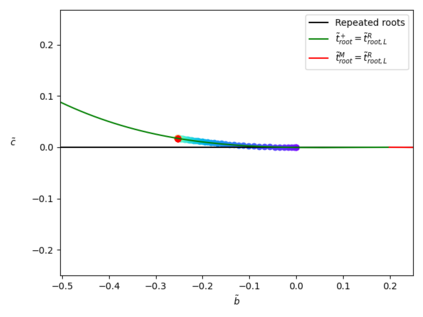
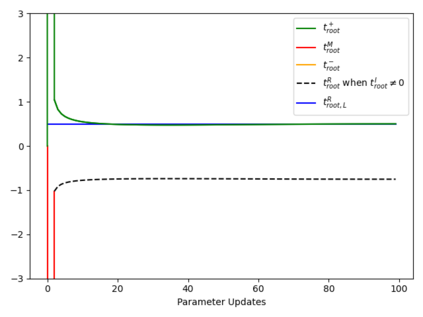
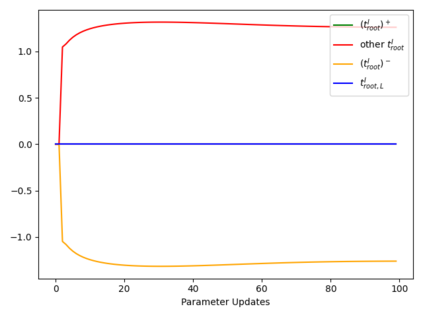
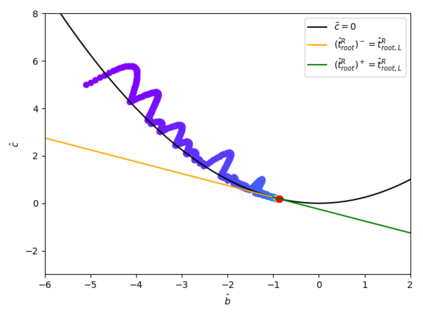
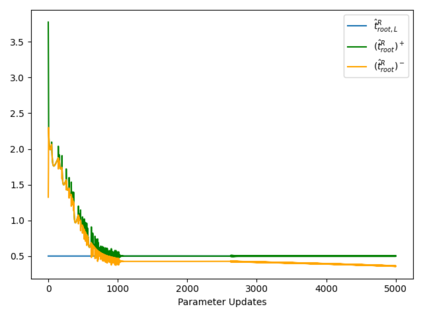
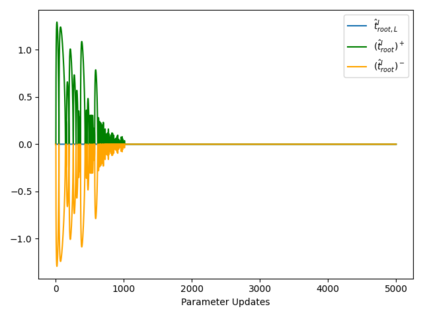
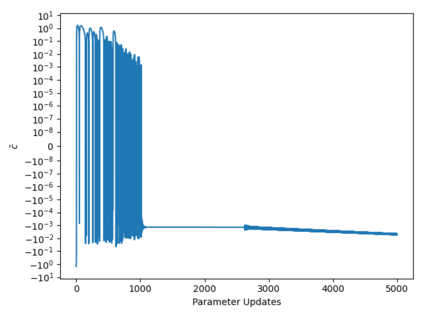
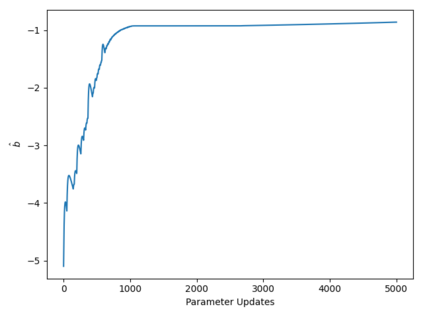
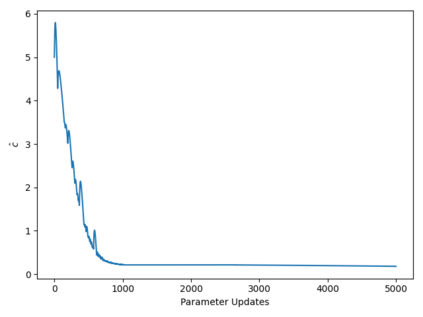
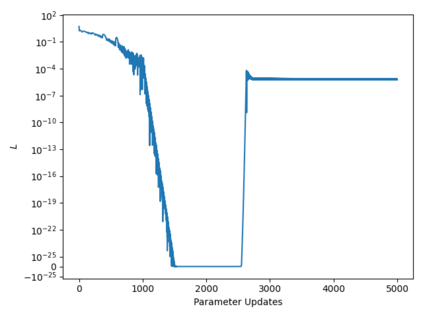
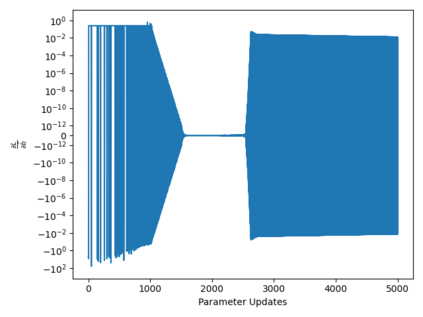
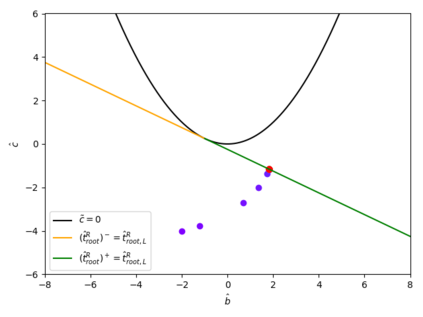
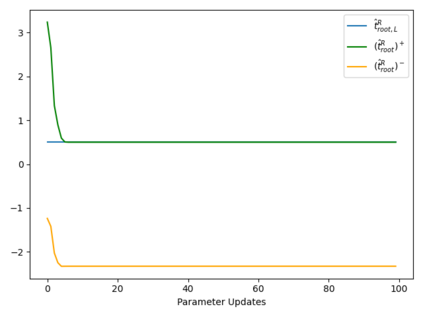
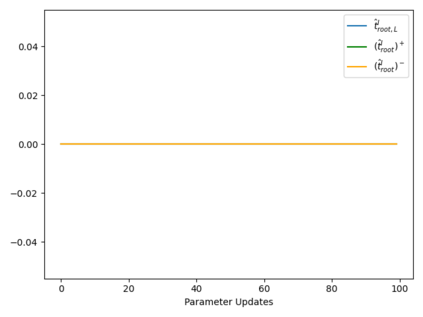
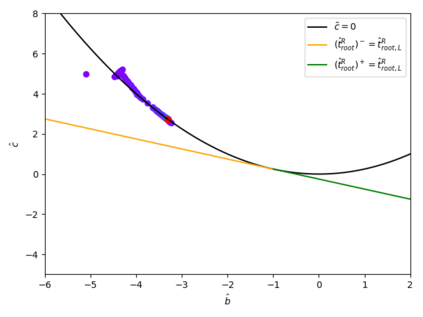
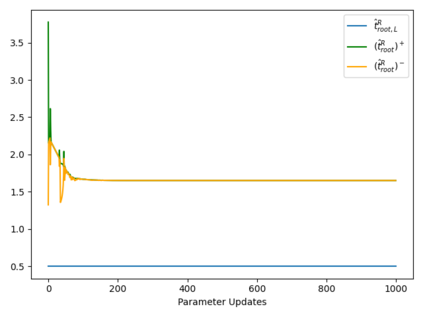
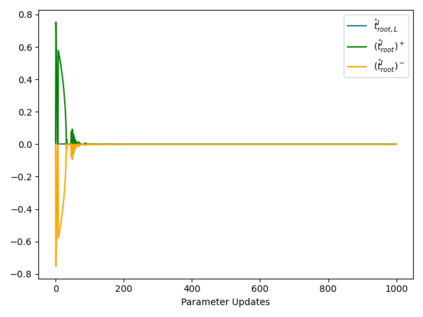
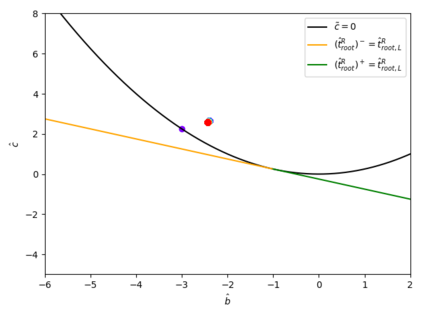
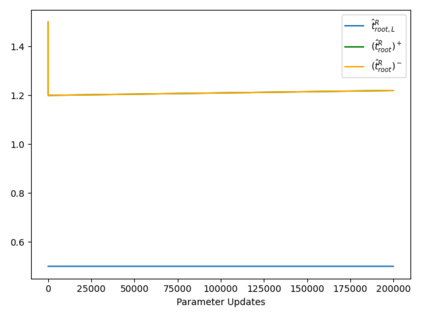
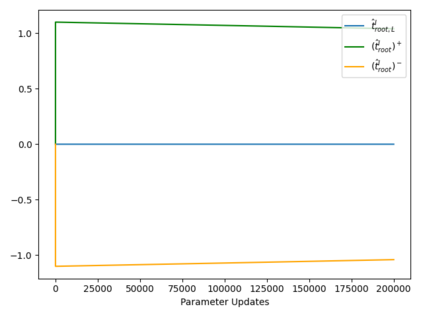
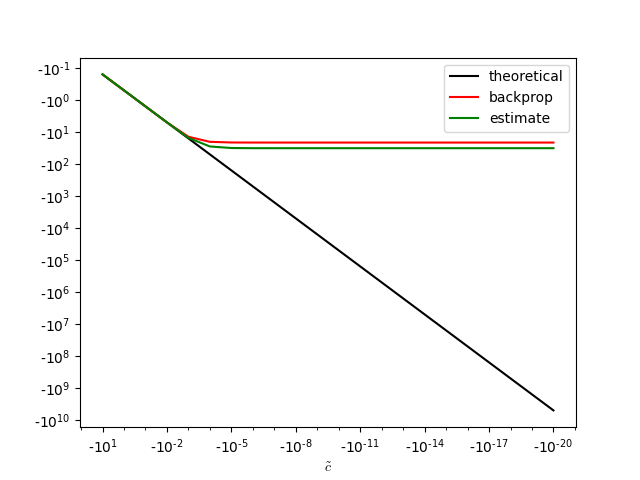
There are many physical processes that have inherent discontinuities in their mathematical formulations. This paper is motivated by the specific case of collisions between two rigid or deformable bodies and the intrinsic nature of that discontinuity. The impulse response to a collision is discontinuous with the lack of any response when no collision occurs, which causes difficulties for numerical approaches that require differentiability which are typical in machine learning, inverse problems, and control. We theoretically and numerically demonstrate that the derivative of the collision time with respect to the parameters becomes infinite as one approaches the barrier separating colliding from not colliding, and use lifting to complexify the solution space so that solutions on the other side of the barrier are directly attainable as precise values. Subsequently, we mollify the barrier posed by the unbounded derivatives, so that one can tunnel back and forth in a smooth and reliable fashion facilitating the use of standard numerical approaches. Moreover, we illustrate that standard approaches fail in numerous ways mostly due to a lack of understanding of the mathematical nature of the problem (e.g. typical backpropagation utilizes many rules of differentiation, but ignores L'Hopital's rule).
翻译:暂无翻译