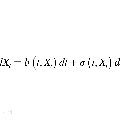基于非凸控制区域的倒向重随机控制系统最优控制必要条件的研究

项目名称： 基于非凸控制区域的倒向重随机控制系统最优控制必要条件的研究

项目编号： No.11526195

项目类型： 专项基金项目

立项/批准年度： 2016

项目学科： 数理科学和化学

项目作者： 王维峰

作者单位： 中南民族大学

项目金额： 2.5万元

中文摘要： 随机最优控制问题是目前比较活跃的一个学术领域。本项目在控制区域非凸且扩散系数含有控制变量的情形下研究倒向重随机控制系统的必要条件、充分条件以及最大值原理。众所周知，对于非凸控制区域情形下的控制问题我们通常将采用针状变分方法，利用伴随方程和对偶关系来得到最大值原理。然而由于本项目中扩散系数也含有控制变量，故仅仅依靠一阶伴随方程是不够的,还需要进行二阶估计。而构造一个对于倒向方程合适的二阶伴随方程是非常困难的。所以经典的方法在这里不能直接应用。我们拟利用倒向重随机微分方程和正-倒向(重)随机微分方程之间的关系，通过某些条件或者方法将我们所研究的内容转化为正-倒向随机微分方程的控制问题，然后再结合吴臻教授解决的带约束条件的控制问题的方法来完成本项目。我们的第二个想法是拟结合 S. Bahlali 教授在研究正-倒向随机控制系统时所采用的松控制方法来研究本项目。

中文关键词： 随机微分方程；最优控制；Pontryagin 最大值原理；线性结构；

英文摘要： Stochastic optimal control problem is a very hot subject today. This project studies the necessary conditions，sufficient conditions and maximum principle for backward doubly stochastic differential equation based on non-convex control domain. Moreover,the control variable is contained in the diffusion coefficient. It is well known that we usually use spike variation method to obtain the maximum principle by the adjoint equation and dual relation. Since the control variable is also contained in the diffusion coefficient,it is not enough to finish our project only by the first-order adjoint equation. We need to give the second-order estimates and the second-order adjoint equation. However, the backward equation is so complicated that it is difficult to construct such an equation. Thus the classical method can not be directly used here. We attempt to use some conditions or methods to transform our problem into the forward-backward stochastic control system by the relationship of backward doubly stochastic differential equation and forward-backward (doubly) stochastic differential equation. And then we may use the method introduced by professor Wu Zhen in a control problem with constraints to finish our project. And the second idea is to use the relaxed control method applied in the forward-backward stochasti

英文关键词： stochastic differential equations；optimal control；Pontryagin’s maximum principle；linear structure；