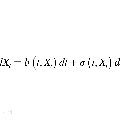随机微分方程守恒型数值方法研究

项目名称： 随机微分方程守恒型数值方法研究

项目编号： No.11201125

项目类型： 青年科学基金项目

立项/批准年度： 2013

项目学科： 数理科学和化学

项目作者： 张静静

作者单位： 河南理工大学

项目金额： 22万元

中文摘要： 本项目以Stratonovich积分意义下受维纳过程驱动且具有守恒量的随机微分方程为研究对象，拟利用包括离散梯度方法在内的数学方法系统研究守恒型数值方法的构造理论，分析所构造数值方法在强弱收敛意义下的收敛性、数值精度、相容性、稳定性等，进而设定合适的修正方程，发展所构造方法在强弱收敛意义下的向后误差分析理论，探讨守恒型数值方法在长时间数值计算中保持一个或多个守恒量的算法机理。在算法执行方面，对任意取值的离散维纳过程增量可能引起的算法不适定问题，拟在保证算法数值精度前提下，用有界的随机变量代替离散维纳过程增量，给出算法可靠数值计算的理论依据。本项目重视理论分析和数值实现相结合，基于算法的构造理论，拟提供守恒型算法数值实现的Matlab程序包。项目的预期成果可望为物理、生物等领域中具有守恒量的随机微分方程的高性能数值计算提供新方法和理论依据，进一步推进随机微分方程的保结构算法研究。

中文关键词： 随机微分方程；守恒量；离散梯度方法；精确离散；连续级方法

英文摘要： This proposal focuses on stochastic differential equations in Strotonovich sense driven by Wiener process with conserved quantities. We plan to do a systematic research on constructing conserved numerical methods of stochastic differential equations based on mathematical methods including discrete gradient approach; present theoretical analysis on convergence, numerical accuracy in strong and weak sense, compatibility and stability analysis for these methods; develop backward error analysis theory by assuming proper modified equations in strong and weak sense; explore conservative mechanism of our methods on preserving one or more conserved quantities in the long time numerical computation. To solve the possible improperly posed problem caused by discrete wiener process increment with arbitrary value, we will, based on the guaranteed numerical accuracy, replace discrete wiener process increment with bounded random variable, which lays a theoretical basis for the reliable numerical implementaion of our methods. We stress the combination of theoretical and numerical implementation studies, and expect to provied Matlab program package for numerical implementation based on the systematic construction theory of our methods. The results of this study are expected to provide new methods and theoretical foundations

英文关键词： stochastic differential equations；conserved quantity；discrete gradient approach；exact discretization；continuous stage method