Conditional Relative Risk: An association measure for longitudinal data analysis

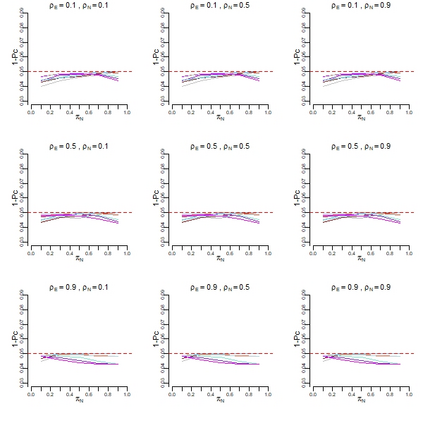
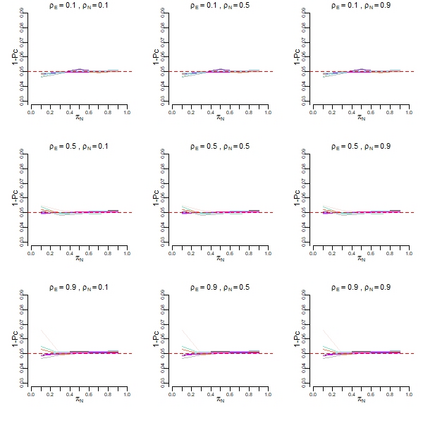
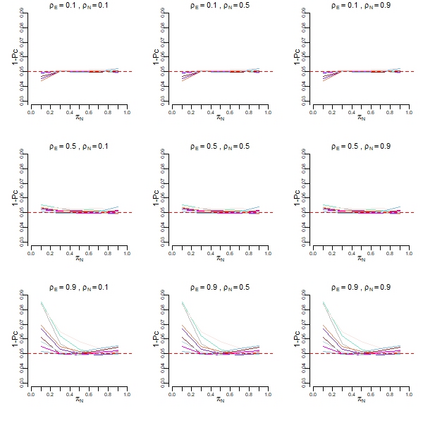
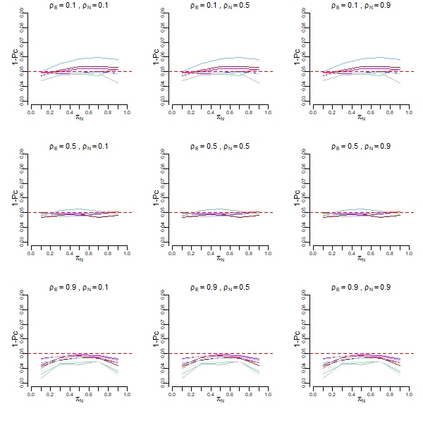
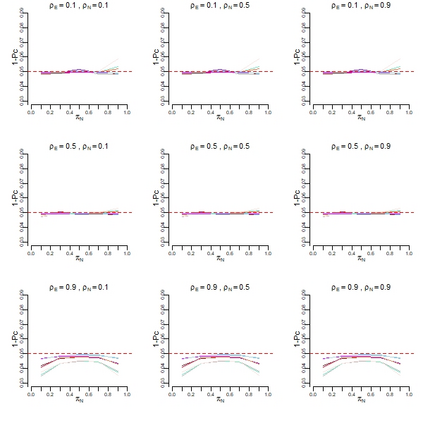
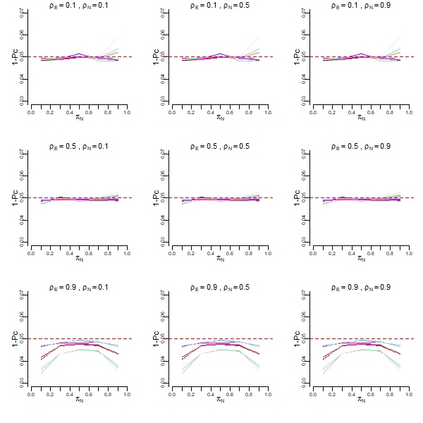
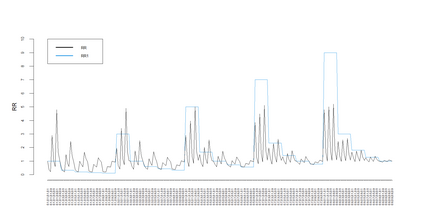
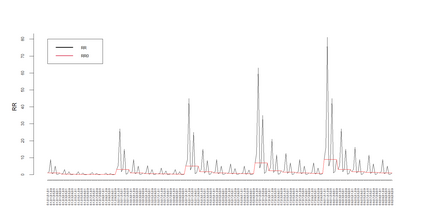
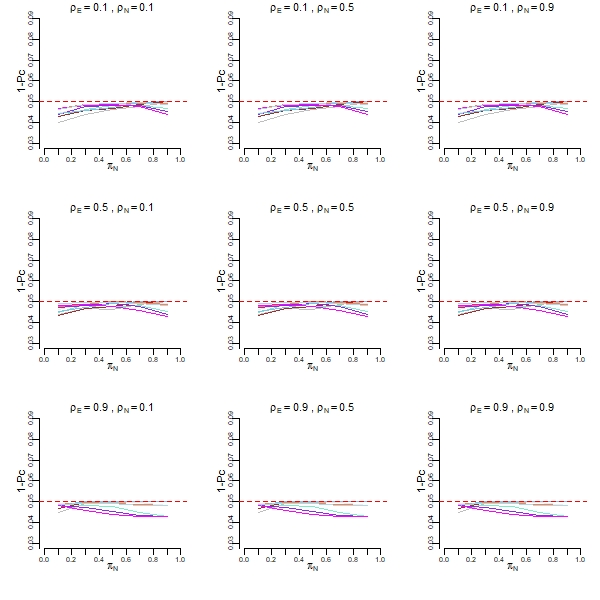
In this paper, we propose a novel association measure for longitudinal studies based on the traditional definition of relative risk. In a Markovian fashion, such a proposal takes into account the information content regarding the previous time. We derive its corresponding confidence interval by means of the Delta method having in mind the crude association between factor and event. Also, we study the properties of our uncertainty quantification scheme through an exhaustive simulation study. Our findings show that the coverage probability is quite close to the level of confidence. Finally, our proposal has a reasonable interpretation from the epidemiological as well as the statistical point of view.