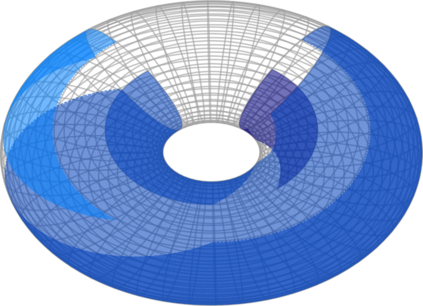
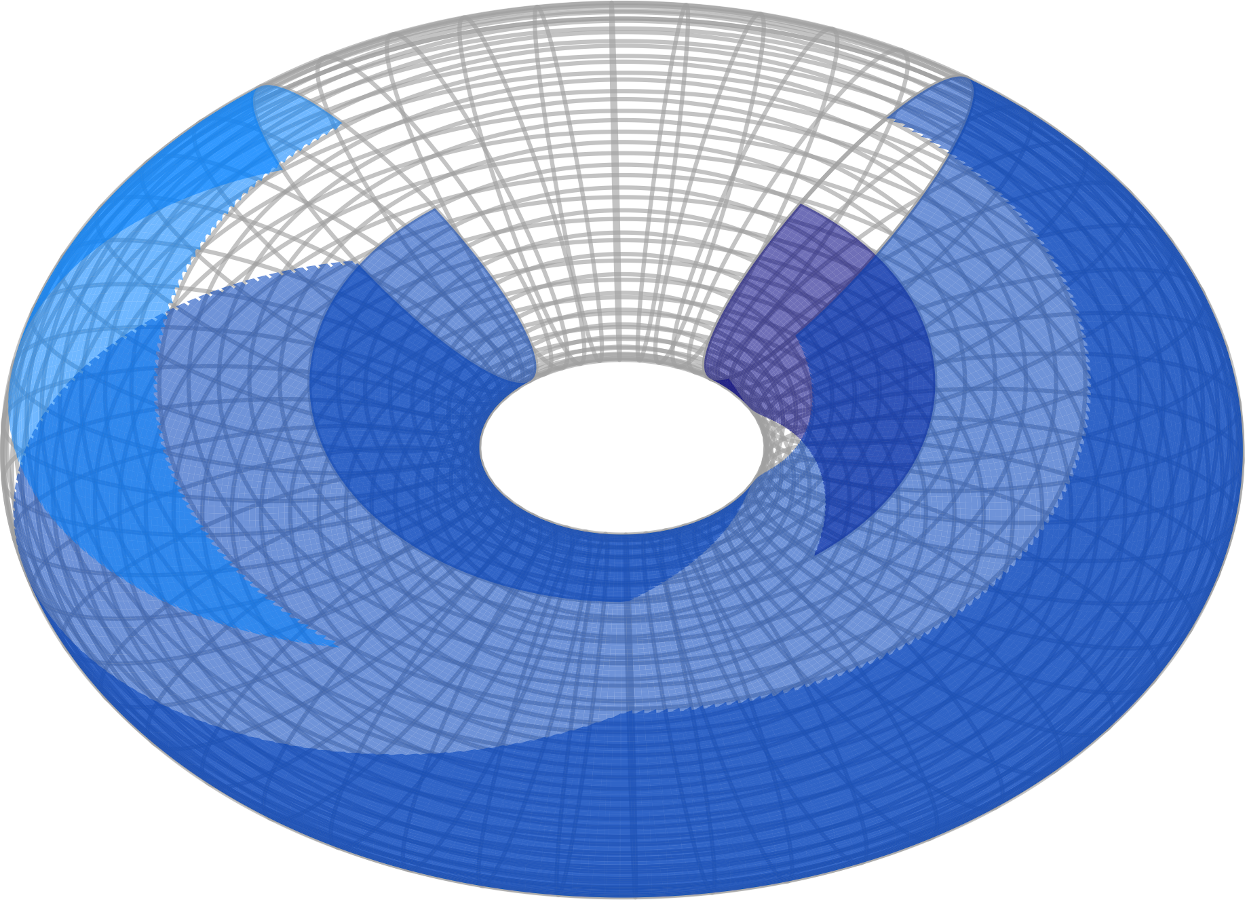
The Periodic Event Scheduling Problem (PESP) is the standard mathematical tool for optimizing periodic timetabling problems in public transport. A solution to PESP consists of three parts: a periodic timetable, a periodic tension, and integer periodic offset values. While the space of periodic tension has received much attention in the past, we explore geometric properties of the other two components, establishing novel connections between periodic timetabling and discrete geometry. Firstly, we study the space of feasible periodic timetables, and decompose it into polytropes, i.e., polytopes that are convex both classically and in the sense of tropical geometry. We then study this decomposition and use it to outline a new heuristic for PESP, based on the tropical neighbourhood of the polytropes. Secondly, we recognize that the space of fractional cycle offsets is in fact a zonotope. We relate its zonotopal tilings back to the hyperrectangle of fractional periodic tensions and to the tropical neighbourhood of the periodic timetable space. To conclude we also use this new understanding to give tight lower bounds on the minimum width of an integral cycle basis.
翻译:定期事件日程安排问题(PESP)是优化公共运输中定期计时问题的标准数学工具。 PESP 的解决方案由三部分组成: 定期时间表、 周期紧张和整数定期抵消值。 虽然周期紧张的空间在过去受到了很多关注, 我们探索了其他两个组成部分的几何特性, 在定期计时和离散几何学之间建立了新的联系。 首先, 我们研究可行的定期时间表空间, 并将其分解为多质间, 即古老和热带几何意义上的多面形。 我们然后研究这一分解, 并用它来根据多质间距的热带周边为 PESP 勾画出一种新的超低值。 第二, 我们认识到分量周期抵消的空间实际上是一个zonotoble。 我们将其骨质图图图图图与分解的高度相交错, 以及定期时间表空间的热带相邻系。 我们还利用这一新理解, 在最小整体周期的宽度基础上给予紧密的宽度。