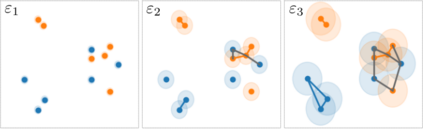
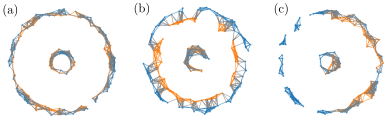
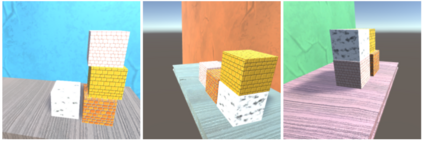
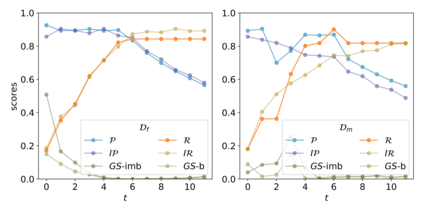
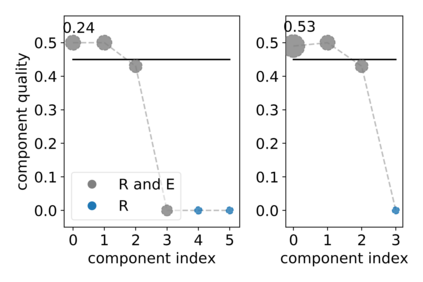
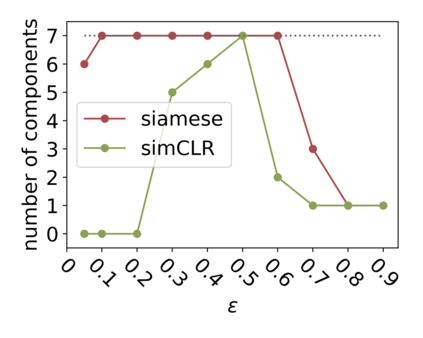
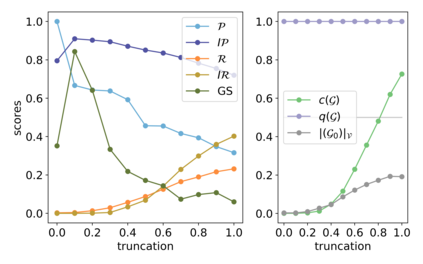
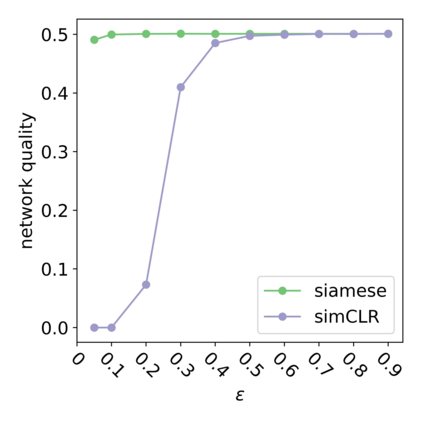
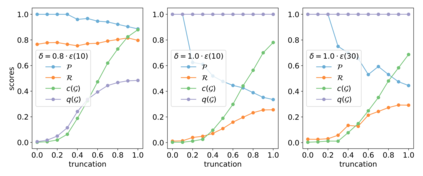
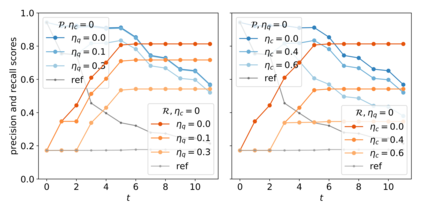
Evaluating the quality of learned representations without relying on a downstream task remains one of the challenges in representation learning. In this work, we present Geometric Component Analysis (GeomCA) algorithm that evaluates representation spaces based on their geometric and topological properties. GeomCA can be applied to representations of any dimension, independently of the model that generated them. We demonstrate its applicability by analyzing representations obtained from a variety of scenarios, such as contrastive learning models, generative models and supervised learning models.
翻译:在这项工作中,我们提出几何组成部分分析算法,根据代表空间的几何和地貌特性来评价其代表空间。 GeomCA可以适用于任何层面的表述,独立于产生这些表达法的模型。我们通过分析从不同情景(例如对比式学习模式、基因模型和受监督的学习模式)获得的表述来证明其适用性。
相关内容
ACM/IEEE第23届模型驱动工程语言和系统国际会议,是模型驱动软件和系统工程的首要会议系列,由ACM-SIGSOFT和IEEE-TCSE支持组织。自1998年以来,模型涵盖了建模的各个方面,从语言和方法到工具和应用程序。模特的参加者来自不同的背景,包括研究人员、学者、工程师和工业专业人士。MODELS 2019是一个论坛,参与者可以围绕建模和模型驱动的软件和系统交流前沿研究成果和创新实践经验。今年的版本将为建模社区提供进一步推进建模基础的机会,并在网络物理系统、嵌入式系统、社会技术系统、云计算、大数据、机器学习、安全、开源等新兴领域提出建模的创新应用以及可持续性。
官网链接:http://www.modelsconference.org/
专知会员服务
77+阅读 · 2020年6月14日
专知会员服务
65+阅读 · 2020年5月12日
专知会员服务
41+阅读 · 2020年4月11日
专知会员服务
51+阅读 · 2020年2月22日
专知会员服务
12+阅读 · 2019年11月10日
Arxiv
4+阅读 · 2018年5月18日