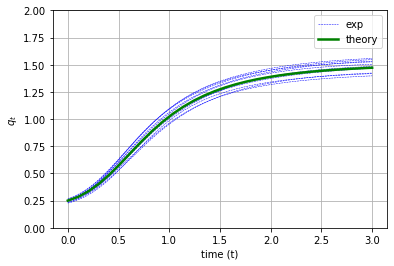
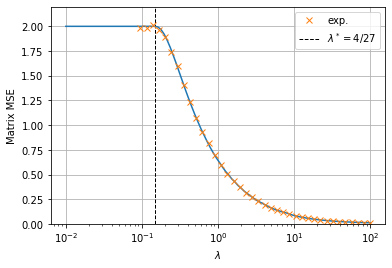
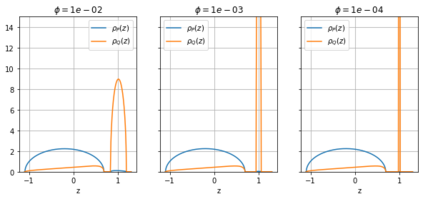
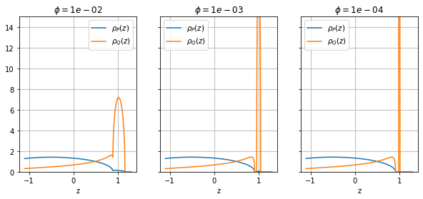
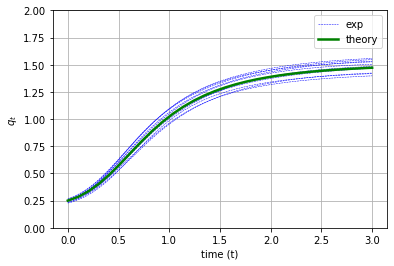
In this work, we present a new approach to analyze the gradient flow for a positive semi-definite matrix denoising problem in an extensive-rank and high-dimensional regime. We use recent linear pencil techniques of random matrix theory to derive fixed point equations which track the complete time evolution of the matrix-mean-square-error of the problem. The predictions of the resulting fixed point equations are validated by numerical experiments. In this short note we briefly illustrate a few predictions of our formalism by way of examples, and in particular we uncover continuous phase transitions in the extensive-rank and high-dimensional regime, which connect to the classical phase transitions of the low-rank problem in the appropriate limit. The formalism has much wider applicability than shown in this communication.
翻译:在这项工作中,我们提出一种新的方法来分析在广泛和高维系统中正半无限期矩阵分解问题的梯度流。我们利用最近随机矩阵理论的线性铅笔技术来得出固定点方程式,以跟踪问题的矩阵-平均比例-偏差的全时间演变情况。由此得出的固定点方程式的预测通过数字实验得到验证。在这份简短的说明中,我们通过实例简要地举例说明了对我们正式主义的一些预测,特别是我们发现了广泛和高维系统中的连续阶段转变,这些转变在适当限度内与低级问题的经典阶段过渡相连接。形式主义的可适用性比这一交流中所显示的要广泛得多。</s>