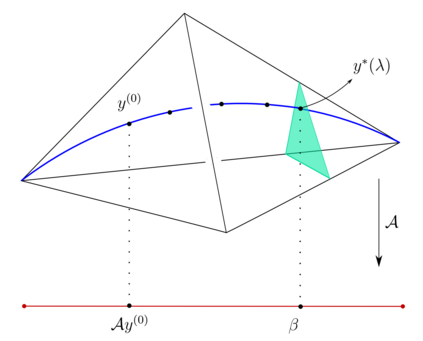
Entropic regularization is a method for large-scale linear programming. Geometrically, one traces intersections of the feasible polytope with scaled toric varieties, starting at the Birch point. We compare this to log-barrier methods, with reciprocal linear spaces, starting at the analytic center. We revisit entropic regularization for unbalanced optimal transport, and we develop the use of optimal conic couplings. We compute the degree of the associated toric variety, and we explore algorithms like iterative scaling.
翻译:成文法是大型线性编程的一种方法。 从 Birch 点开始, 从几何角度, 一个可操作的多面体与缩放多面体的交叉点 。 我们把它和对等的线性空间进行比较, 从分析中心开始 。 我们重新审视成文法对不平衡的最佳运输的成文法, 我们开发使用最佳的二次曲线组合。 我们计算相关成文体品种的程度, 我们探索迭代缩放等算法 。

相关内容
专知会员服务
428+阅读 · 2021年1月11日
专知会员服务
36+阅读 · 2019年10月17日
Arxiv
0+阅读 · 2022年4月19日
Arxiv
0+阅读 · 2022年4月19日
Arxiv
0+阅读 · 2022年4月18日
Arxiv
0+阅读 · 2022年4月15日