Bregman algorithms for a class of Mixed-Integer Generalized Nash Equilibrium Problems

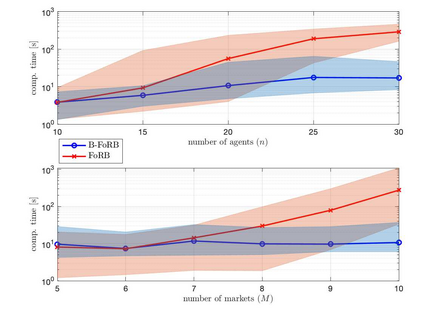
We consider the problem of computing a mixed-strategy generalized Nash equilibrium (MS-GNE) for a class of games where each agent has both continuous and integer decision variables. Specifically, we propose a novel Bregman forward-reflected-backward splitting and design distributed algorithms that exploit the problem structure. Technically, we prove convergence to a variational MS-GNE under monotonicity and Lipschitz continuity assumptions, which are typical of continuous GNE problems. Finally, we show the performance of our algorithms via numerical experiments.