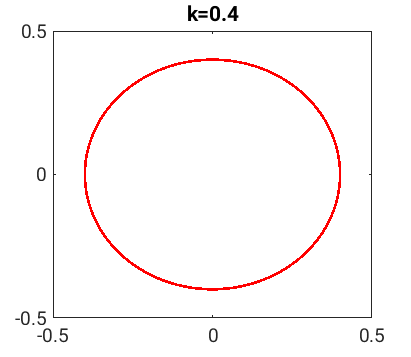
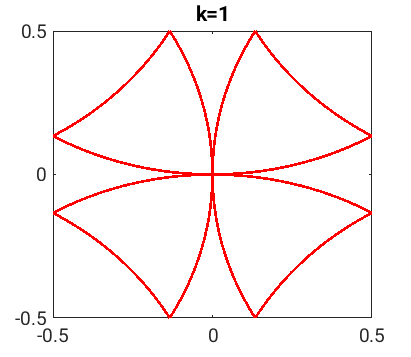
The perfectly matched layer (PML) is a very popular tool in the truncation of wave scattering in unbounded domains. In Chandler-Wilde & Monk et al. 2009, the author proposed a conjecture that for scattering problems with rough surfaces, the PML converges exponentially with respect to the PML parameter in any compact subset. In the author's previous paper (Zhang et al. 2022), this result has been proved for periodic surfaces in two dimensional spaces, when the wave number is not a half integer. In this paper, we prove that the method has a high order convergence rate in the 3D bi-periodic surface scattering problems. We extend the 2D results and prove that the exponential convergence still holds when the wavenumber is smaller than $0.5$. For lareger wavenumbers, although exponential convergence is no longer proved, we are able to prove that a higher order convergence for the PML method.
翻译:完全匹配的层( PML) 是一个非常流行的工具, 用于清除在无约束范围内散落的波浪。 在Chandler- Wilde & Monk 等人 2009 中, 作者提出了一个假设, 即对于粗表面的散射问题, PML 与任何紧凑子集的 PML 参数成指数。 在作者的上一份文件( 张等人 2022 ) 中, 这一结果被证明为两个维空间的周期表层的周期表层, 当波数不是半整数时 。 在本文中, 我们证明该方法在 3D 双周期地表散射问题中具有高度的顺序趋同率。 我们扩展了 2D 的结果, 并证明当波数小于 0. 5 美元时, 指数趋同值仍然维持着。 对于 lareger 浪数, 尽管指数趋同不再被证明, 我们能够证明 PML 方法有更高的顺序趋同 。