A linearly implicit structure-preserving scheme for the fractional sine-Gordon equation based on the IEQ approach

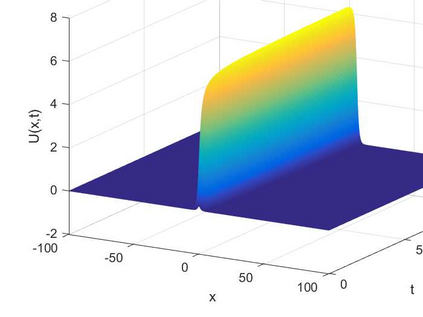
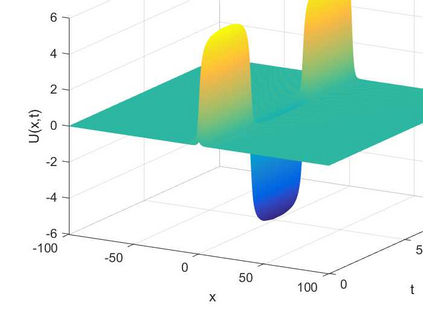
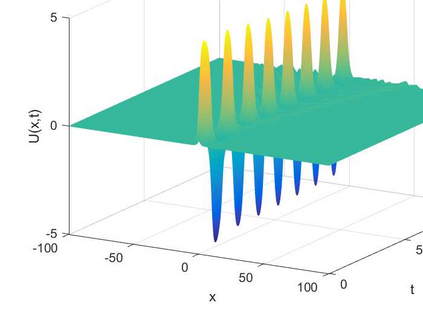
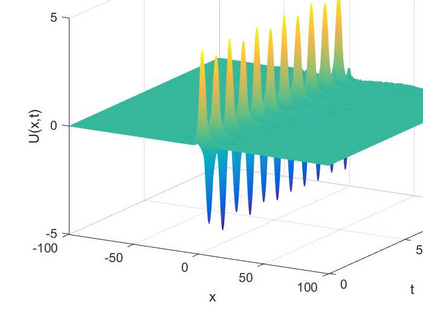
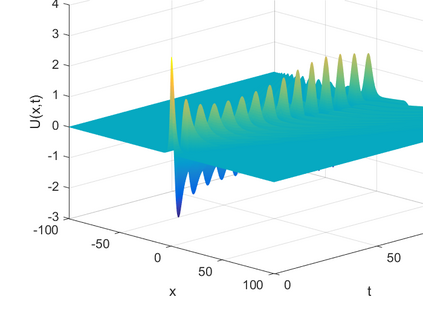
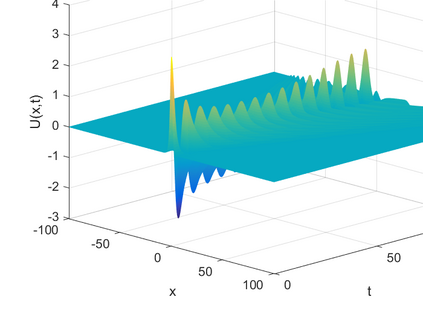
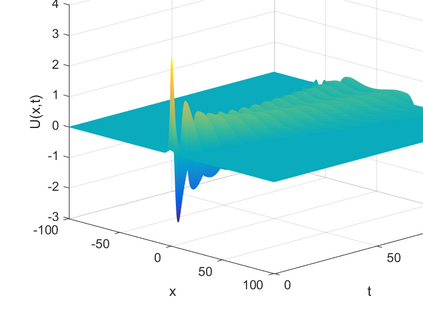
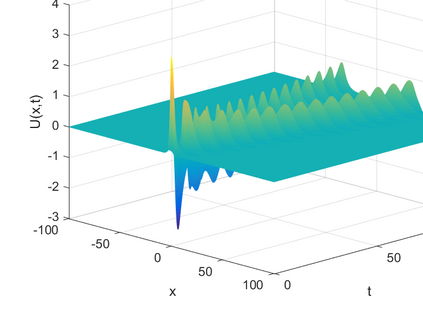
This paper aims to develop a linearly implicit structure-preserving numerical scheme for the space fractional sine-Gordon equation, which is based on the newly developed invariant energy quadratization method. First, we reformulate the equation as a canonical Hamiltonian system by virtue of the variational derivative of the functional with fractional Laplacian. Then, we utilize the fractional centered difference formula to discrete the equivalent system derived by the invariant energy quadratization method in space direction, and obtain a conservative semi-discrete scheme. Subsequently, the linearly implicit structure-preserving method is applied for the resulting semi-discrete system to arrive at a fully-discrete conservative scheme. The stability, solvability and convergence in the maximum norm of the numerical scheme are given. Furthermore, a fast algorithm based on the fast Fourier transformation technique is used to reduce the computational complexity in practical computation. Finally, numerical examples are provided to confirm our theoretical analysis results.