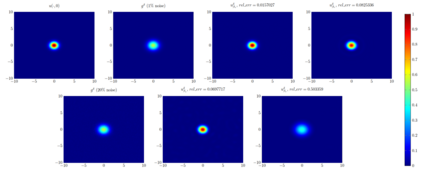
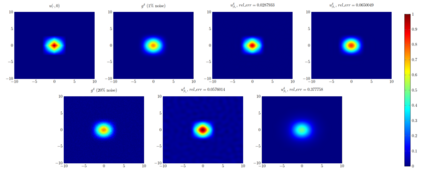
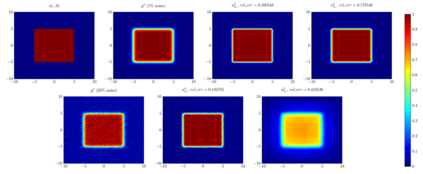
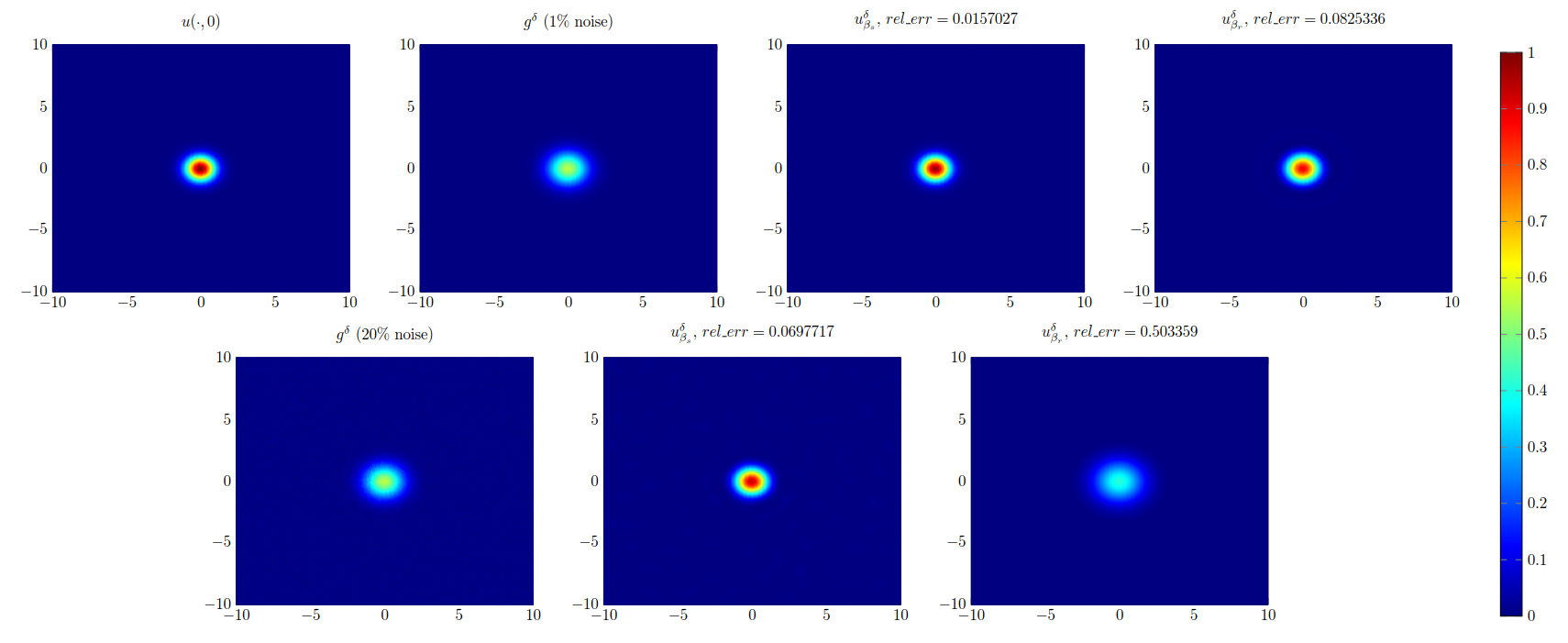
This paper addresses a backward heat conduction problem with fractional Laplacian and time-dependent coefficient in an unbounded domain. The problem models generalized diffusion processes and is well-known to be severely ill-posed. We investigate a simple and powerful variational regularization technique based on mollification. Under classical Sobolev smoothness conditions, we derive order-optimal convergence rates between the exact solution and regularized approximation in the practical case where both the data and the operator are noisy. Moreover, we propose an order-optimal a-posteriori parameter choice rule based on the Morozov principle. Finally, we illustrate the robustness and efficiency of the regularization technique by some numerical examples including image deblurring.
翻译:本文涉及一个在未约束域中与分数拉普拉西亚和时间依赖系数有关的后向热传导问题。 问题模型普及了扩散过程, 众所周知, 传播过程非常糟糕。 我们调查基于软体化的简单而有力的变异性规范化技术。 在传统的索博勒夫平滑条件下, 在数据和操作员都吵闹的实际案例中, 我们得出精确解决方案和常规近似之间的有序- 最佳趋同率。 此外, 我们根据莫罗佐夫原则, 提出一个命令- 最佳的超异性参数选择规则 。 最后, 我们用一些数字例子来说明正规化技术的稳健性和效率, 包括图像破碎。