An ASP approach for reasoning on neural networks under a finitely many-valued semantics for weighted conditional knowledge bases

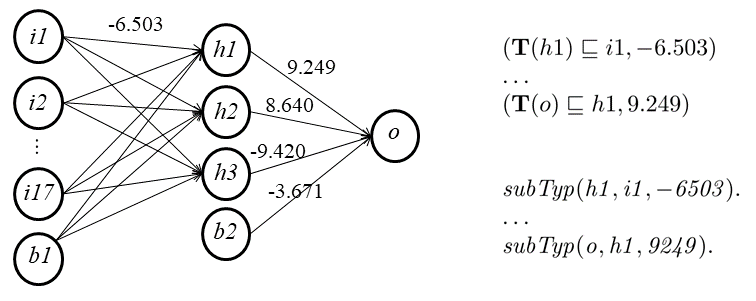
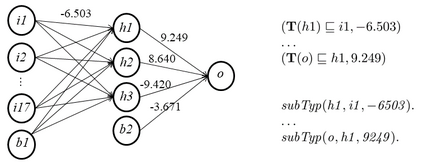
Weighted knowledge bases for description logics with typicality have been recently considered under a "concept-wise" multipreference semantics (in both the two-valued and fuzzy case), as the basis of a logical semantics of MultiLayer Perceptrons (MLPs). In this paper we consider weighted conditional ALC knowledge bases with typicality in the finitely many-valued case, through three different semantic constructions. For the boolean fragment LC of ALC we exploit ASP and "asprin" for reasoning with the concept-wise multipreference entailment under a phi-coherent semantics, suitable to characterize the stationary states of MLPs. As a proof of concept, we experiment the proposed approach for checking properties of trained MLPs.