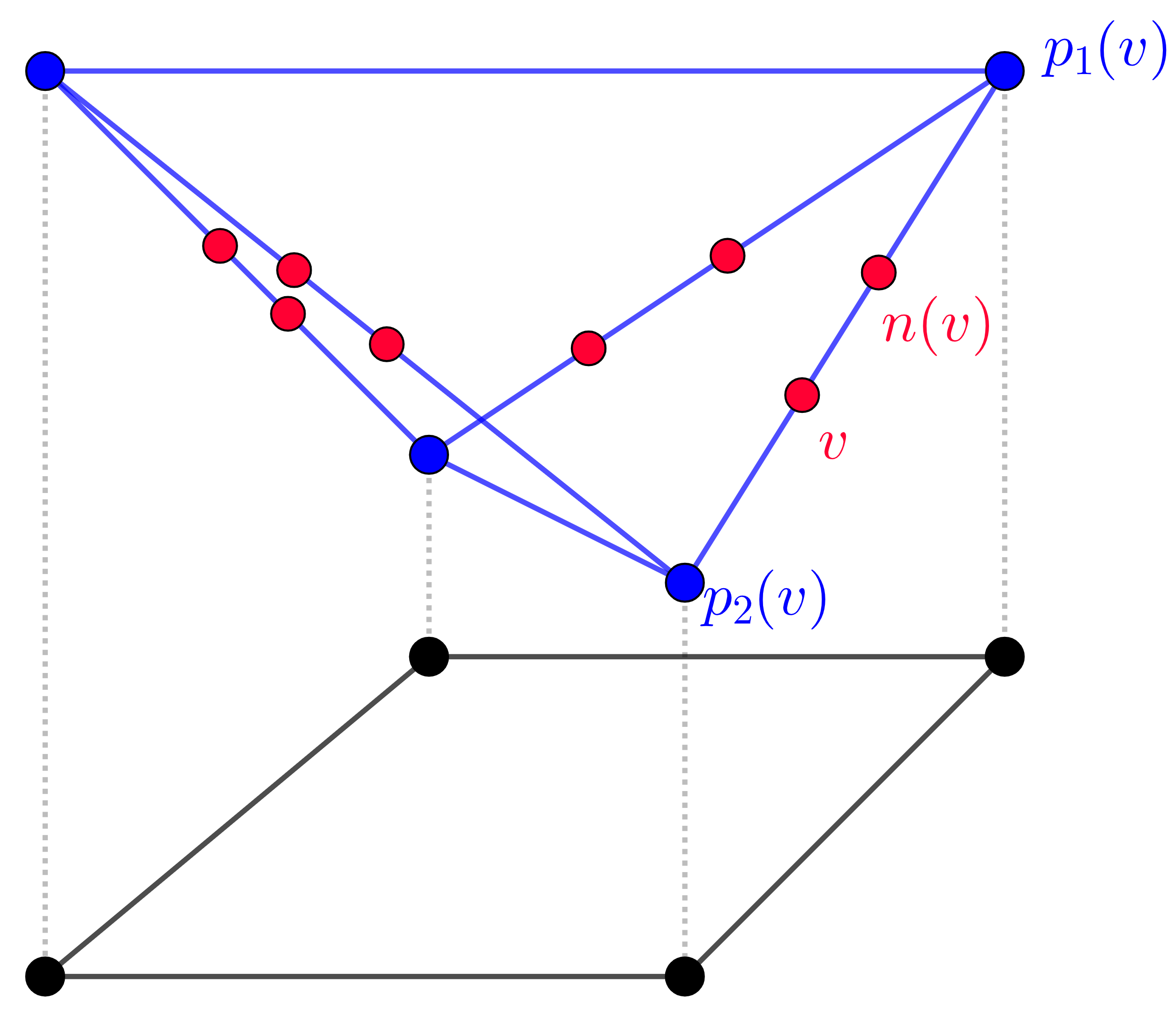
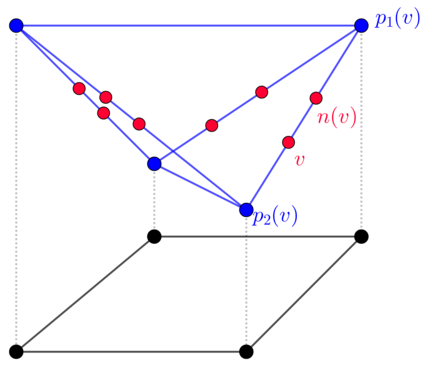
We study the maximum weight convex polytope problem, in which the goal is to find a convex polytope maximizing the total weight of enclosed points. Prior to this work, the only known result for this problem was an $O(n^3)$ algorithm for the case of $2$ dimensions due to Bautista et al. We show that the problem becomes $\mathcal{NP}$-hard to solve exactly in $3$ dimensions, and $\mathcal{NP}$-hard to approximate within $n^{1/2-\epsilon}$ for any $\epsilon > 0$ in $4$ or more dimensions. %\polyAPX-complete in $4$ dimensions even with binary weights. We also give a new algorithm for $2$ dimensions, albeit with the same $O(n^3)$ running time complexity as that of the algorithm of Bautsita et al.
翻译:我们研究的是最大重量Convex 聚苯乙烯问题, 目标是找到一个能最大限度地增加封闭点总重量的复合聚苯乙烯问题。 在这项工作之前, 这一问题的唯一已知结果是, Bautista 等公司对2美元维值的计算法。 我们发现, 问题变得很困难, 很难完全用3美元维值来解决, 而 美元( mathcal{NP} $- hard) 很难在4美元或4美元以上维值中接近0.0美元之内。 ⁇ polyAPX 以4美元维值完成, 即使带有二元重量。 我们还为2美元维值提供了一个新的算法, 尽管与 Bautsita 等公司算法一样, 运行时间复杂。