A Theory of Quantum Subspace Diagonalization

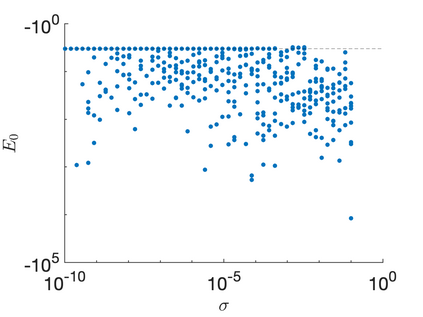
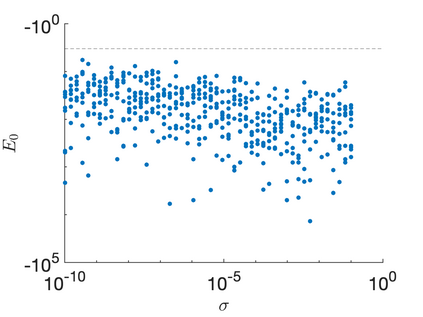
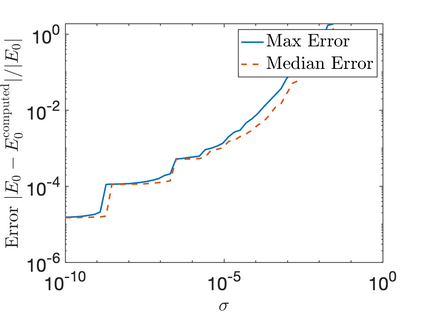
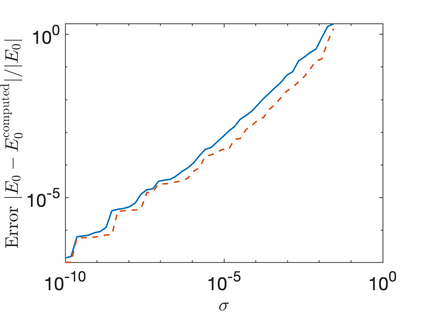
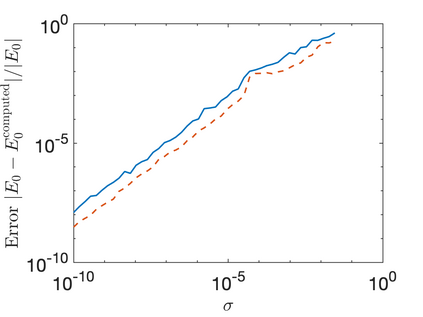
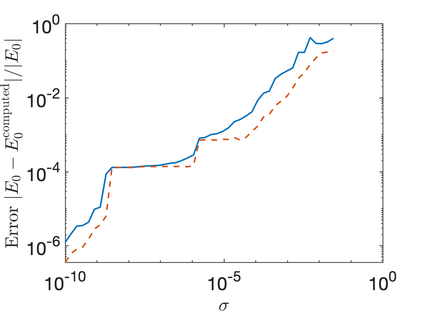
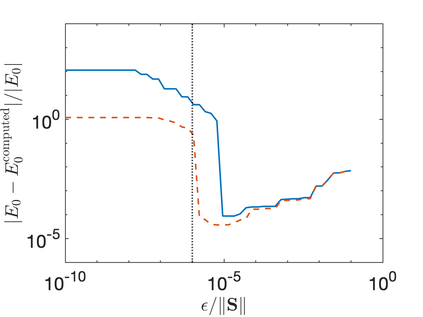
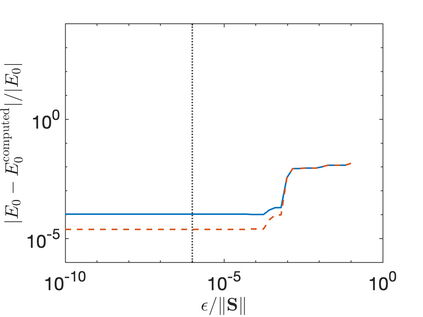
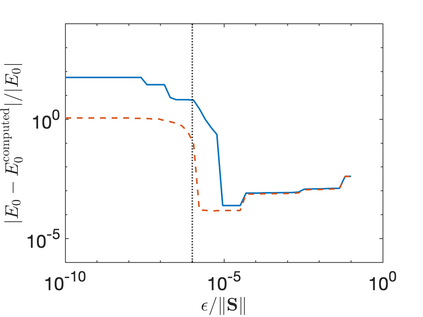
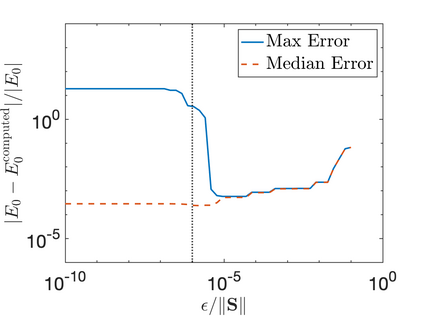
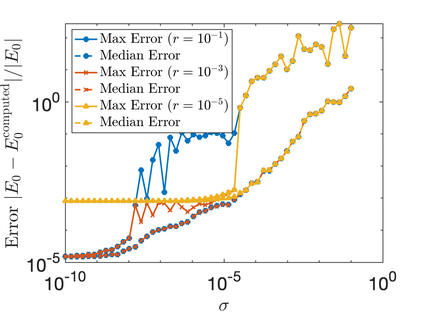
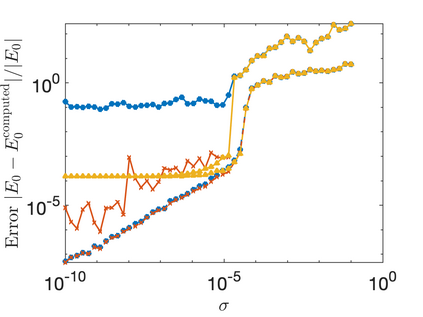
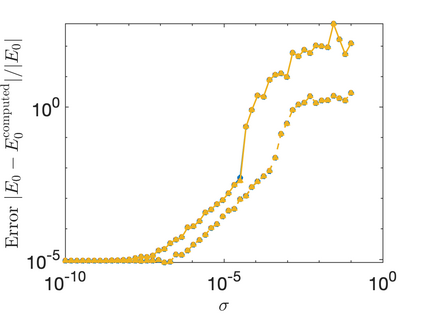
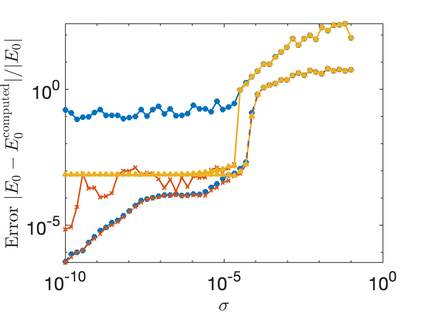
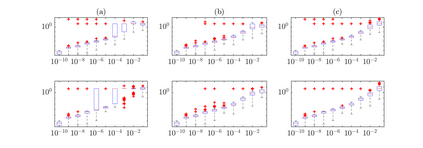
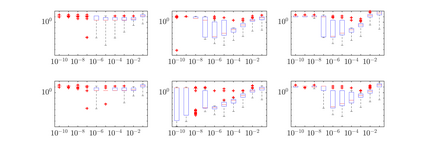
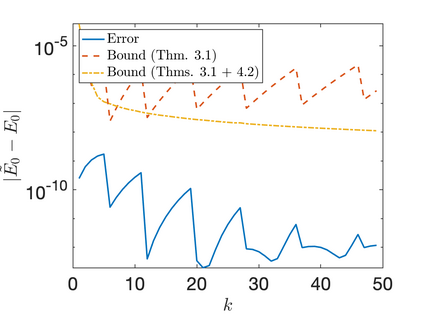
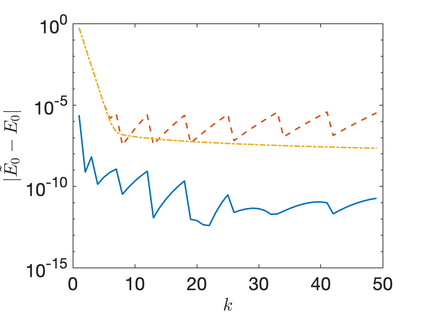
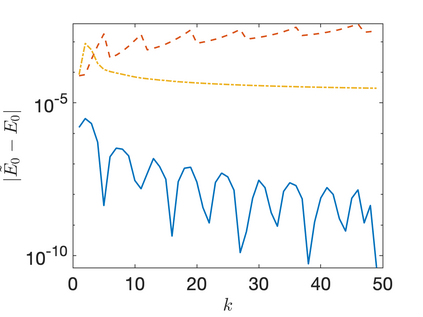
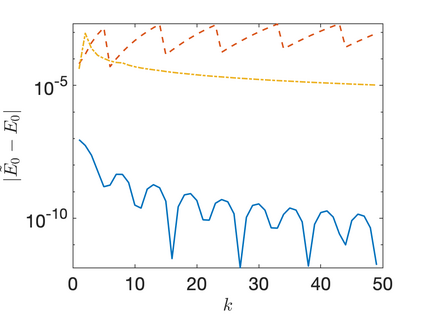
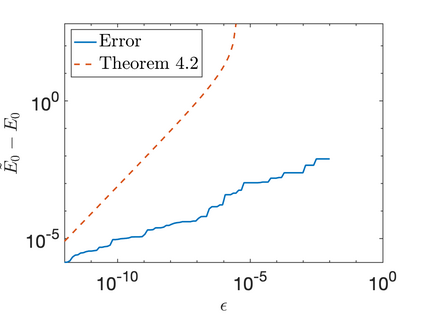
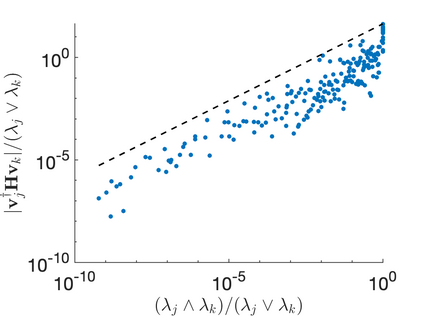
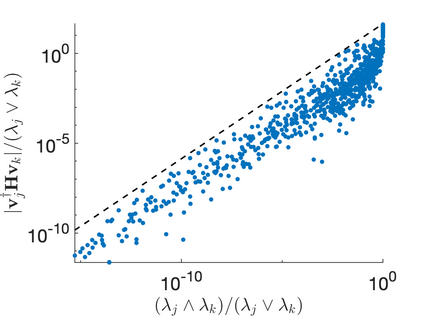
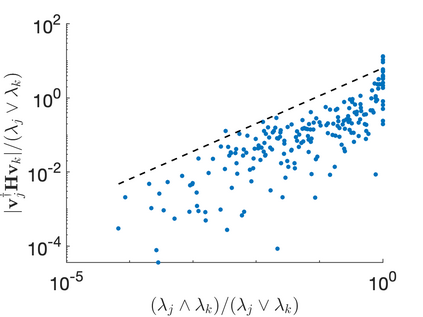
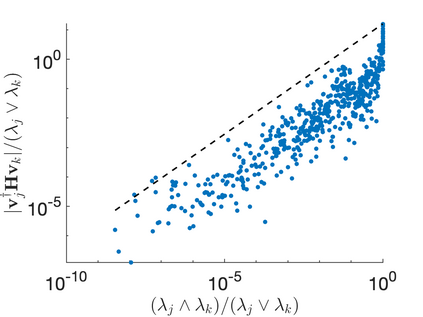
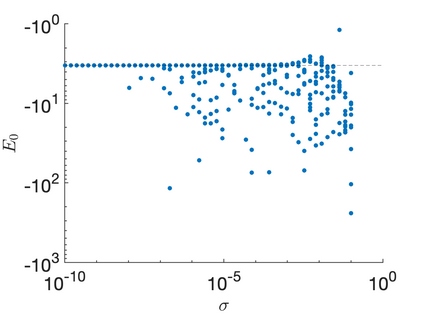
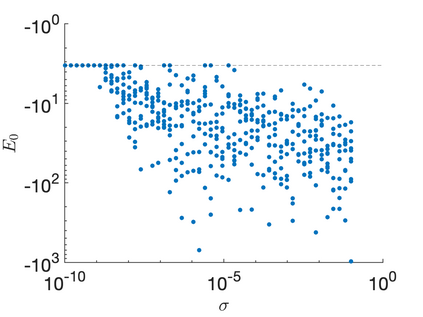
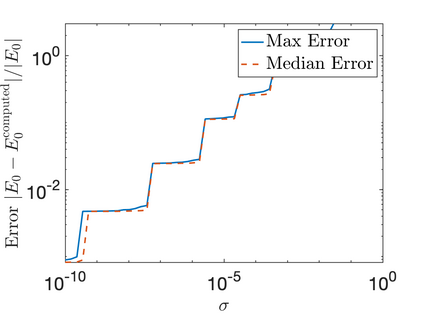
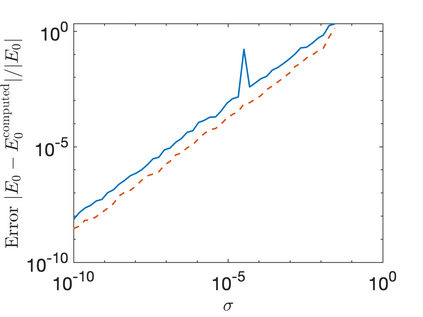
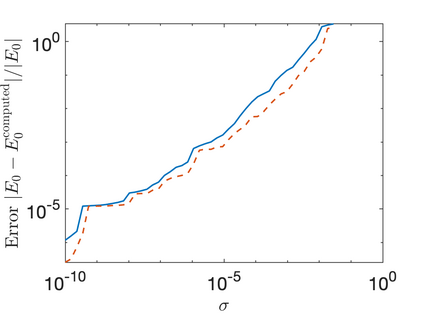
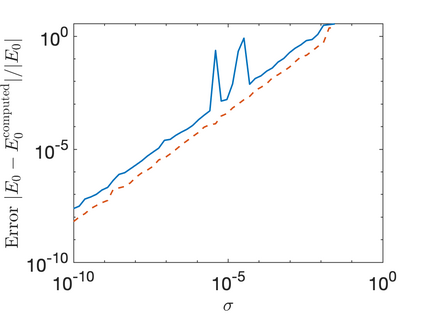
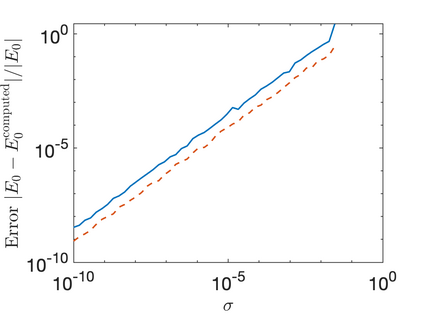
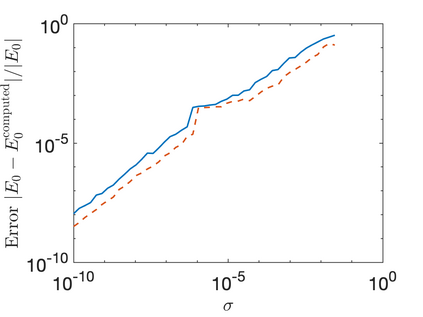
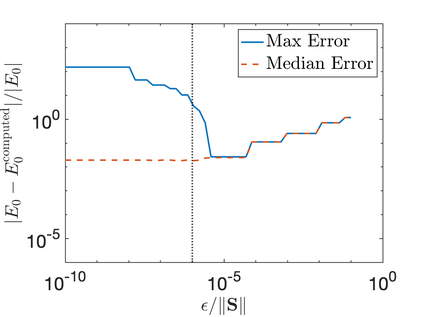
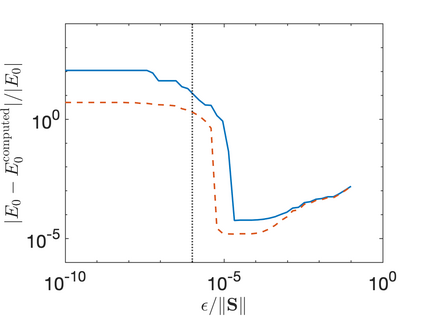
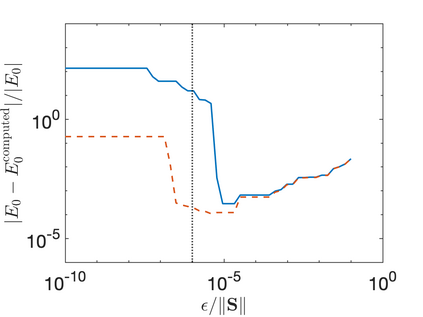
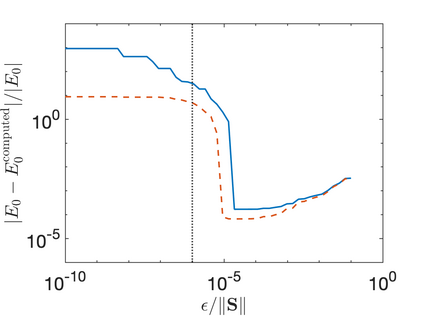
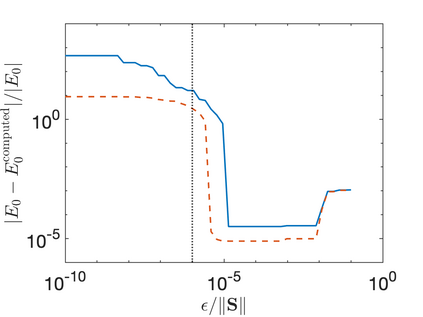
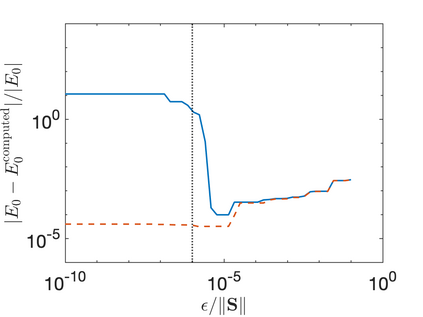
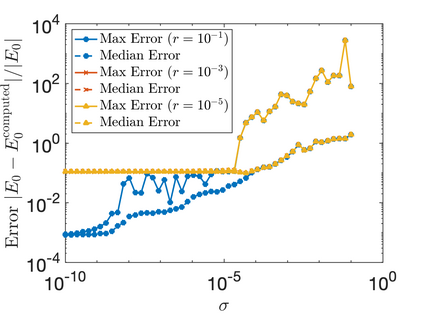
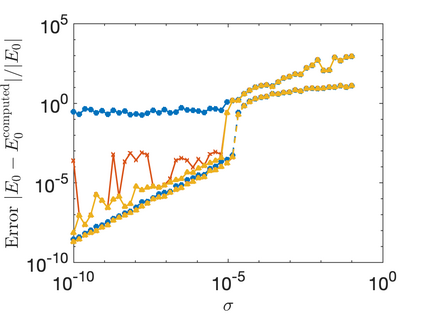
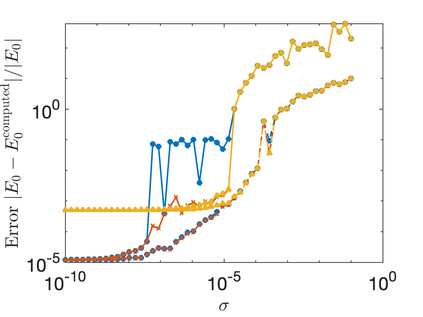
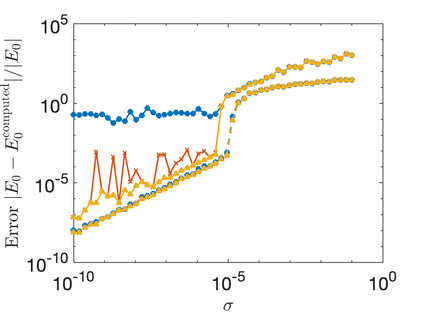
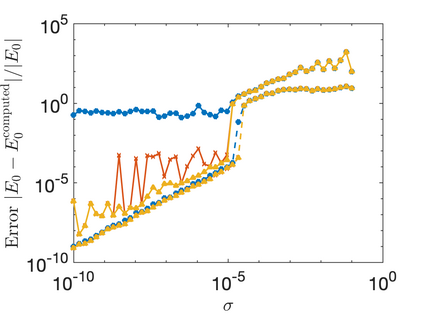
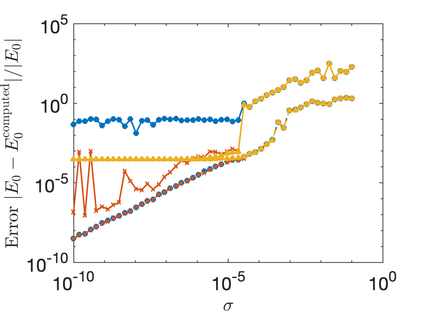
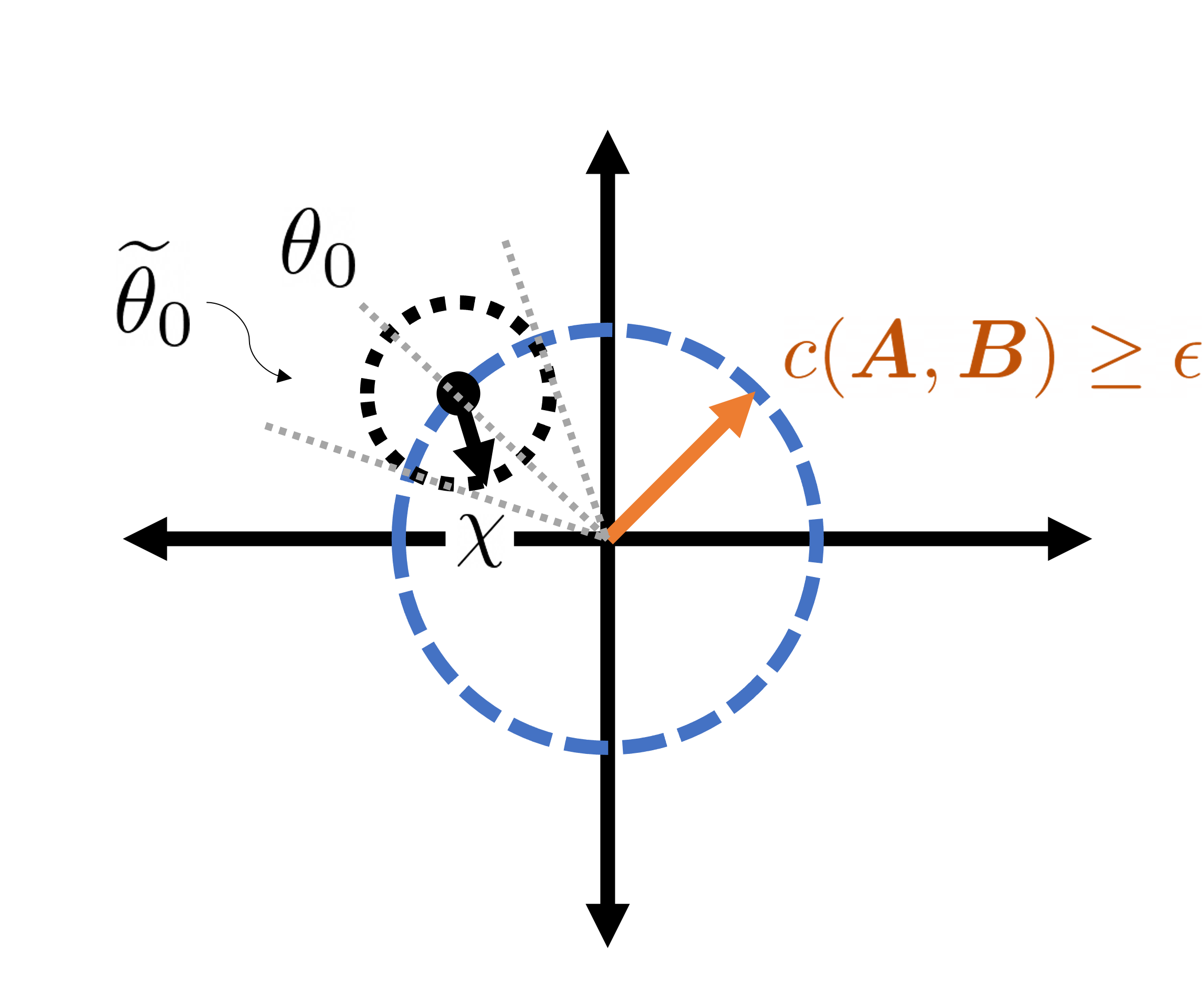
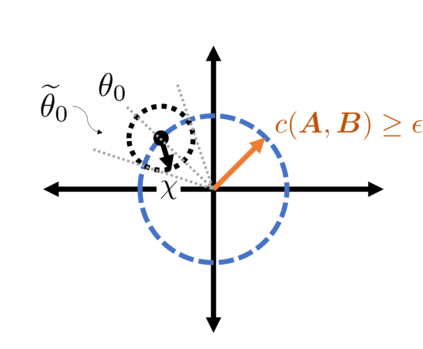Quantum subspace diagonalization methods are an exciting new class of algorithms for solving large scale eigenvalue problems using quantum computers. Unfortunately, these methods require the solution of an ill-conditioned generalized eigenvalue problem, with a matrix pencil corrupted by a non-negligible amount of noise that is far above the machine precision. Despite pessimistic predictions from classical perturbation theories, these methods can perform reliably well if the generalized eigenvalue problem is solved using a standard truncation strategy. We provide a theoretical analysis of this surprising phenomenon, proving that under certain natural conditions, a quantum subspace diagonalization algorithm can accurately compute the smallest eigenvalue of a large Hermitian matrix. We give numerical experiments demonstrating the effectiveness of the theory and providing practical guidance for the choice of truncation level.