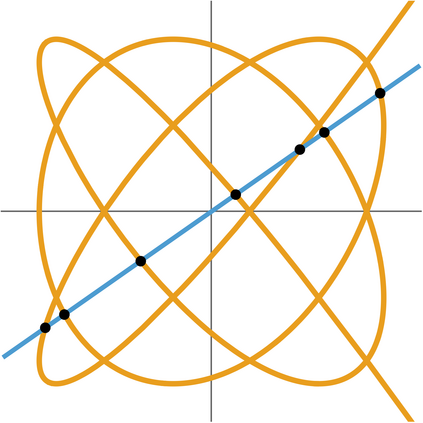
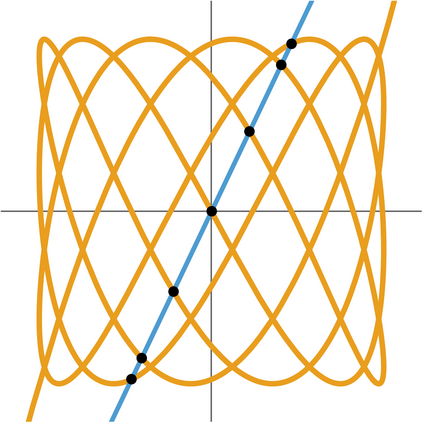
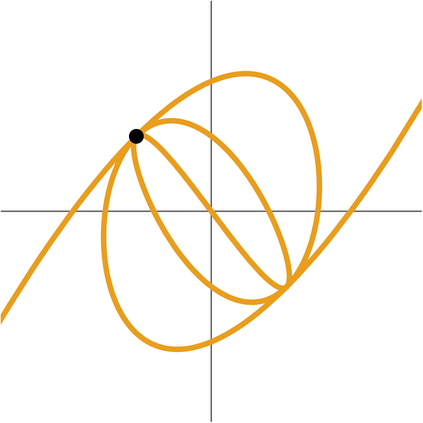
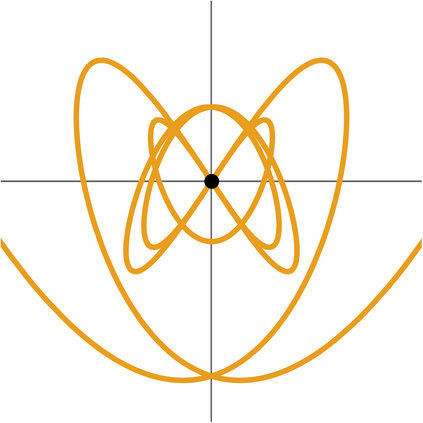
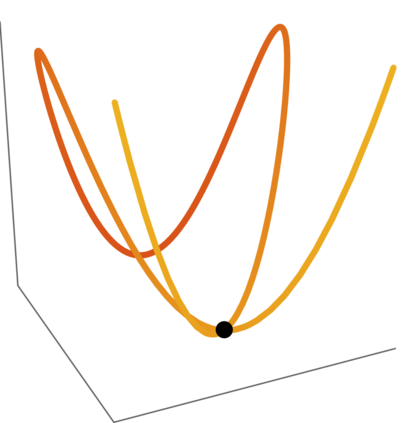
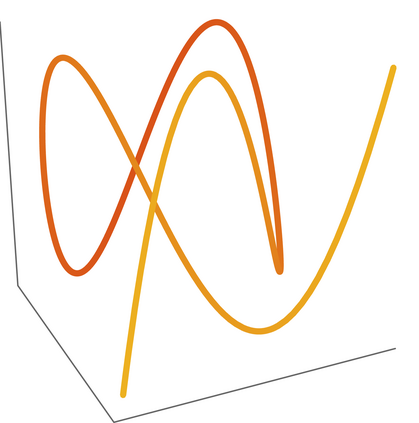
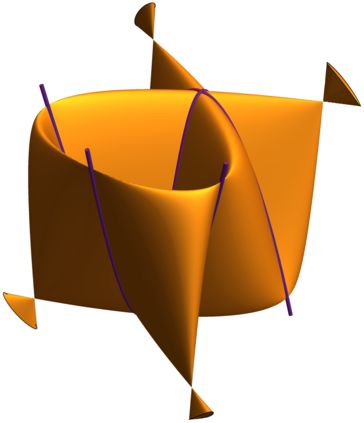
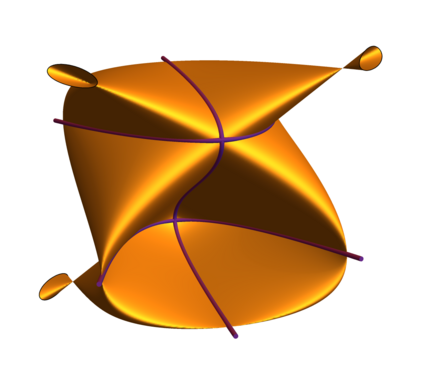
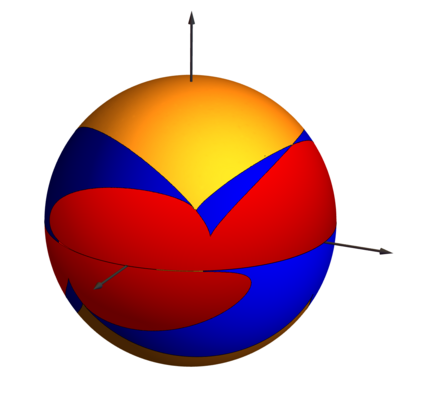
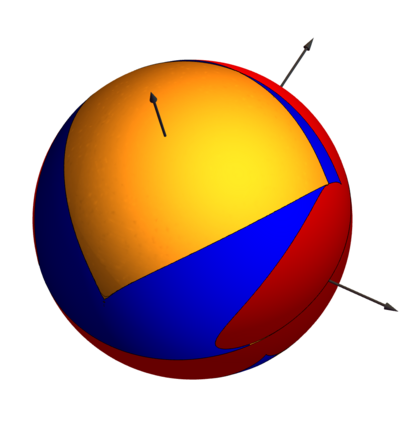
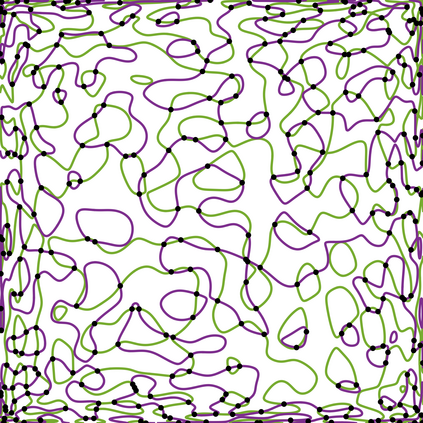
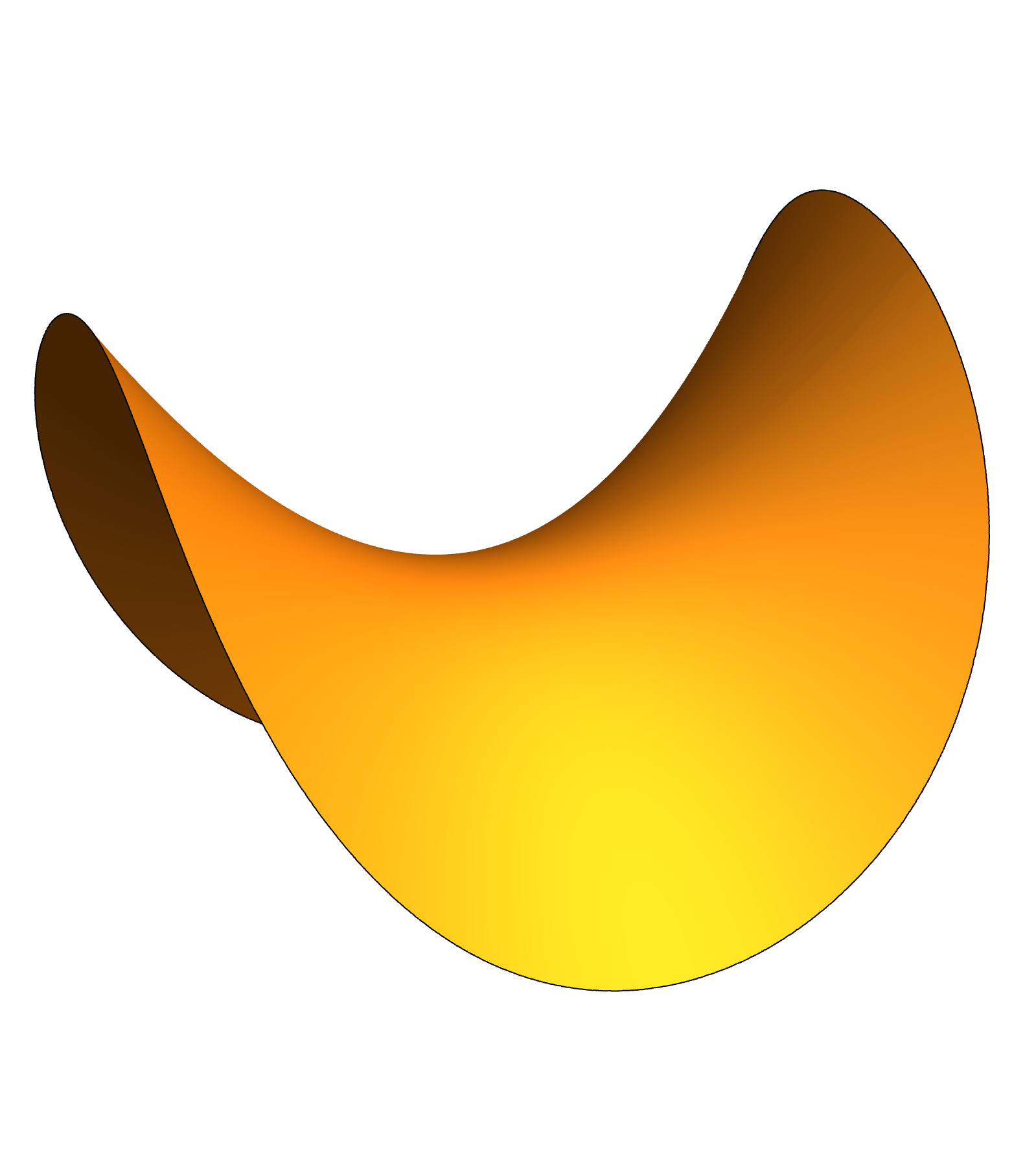
Chebyshev Varieties

Chebyshev varieties are algebraic varieties parametrized by Chebyshev polynomials or their multivariate generalizations. We determine the dimension, degree, singular locus and defining equations of these varieties. We explain how they play the role of toric varieties in sparse polynomial root finding, when monomials are replaced by Chebyshev polynomials. We present numerical root finding algorithms that exploit our results.