A Generalization of the Stratonovich's Value of Information and Application to Privacy-Utility Trade-off

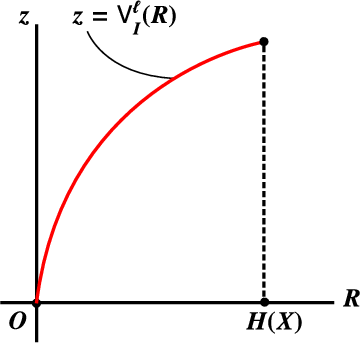
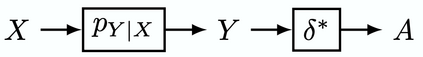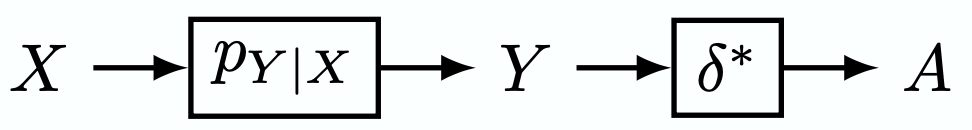The Stratonovich's value of information (VoI) is quantity that measure how much inferential gain is obtained from a perturbed sample under information leakage constraint. In this paper, we introduce a generalized VoI for a general loss function and general information leakage. Then we derive an upper bound of the generalized VoI. Moreover, for a classical loss function, we provide a achievable condition of the upper bound which is weaker than that of in previous studies. Since VoI can be viewed as a formulation of a privacy-utility trade-off (PUT) problem, we provide an interpretation of the achievable condition in the PUT context.