Exchangeable Bernoulli distributions: high dimensional simulation, estimate and testing

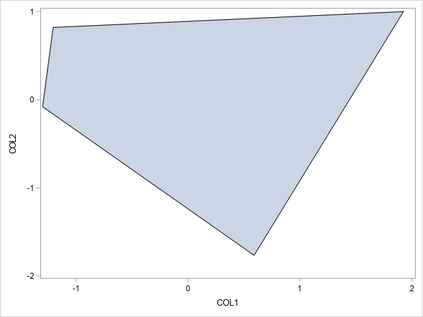
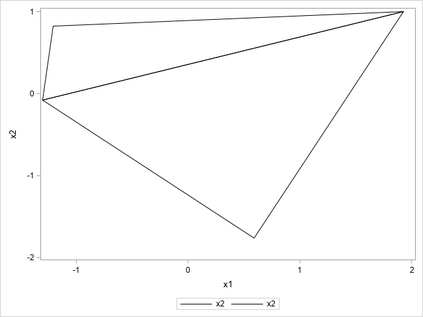
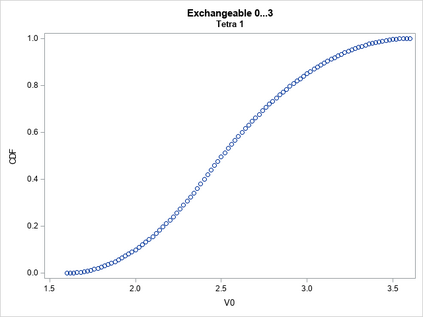
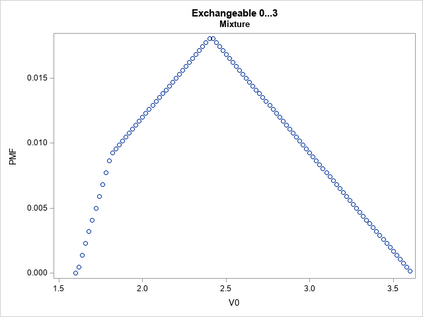
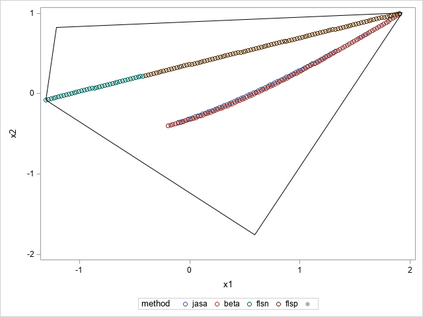
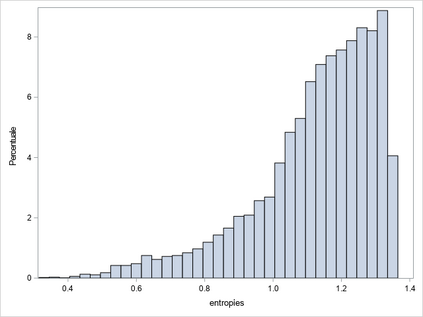
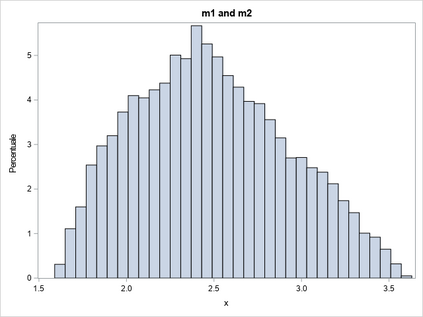
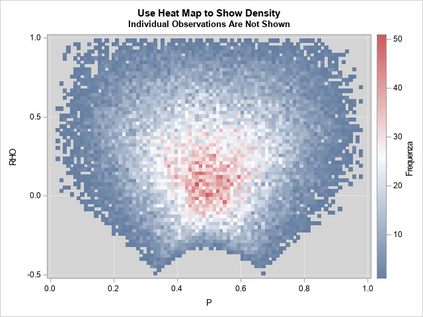
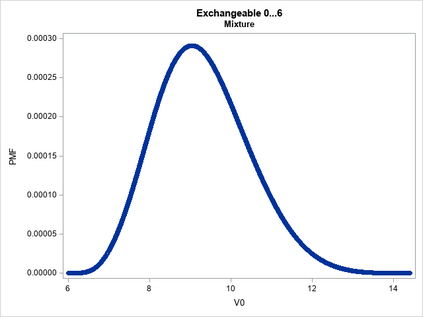
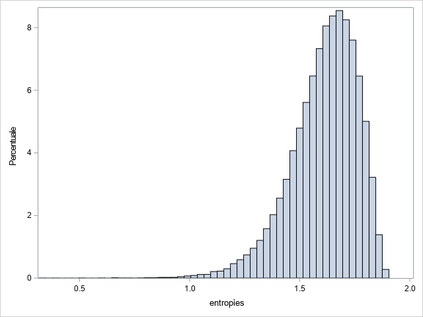
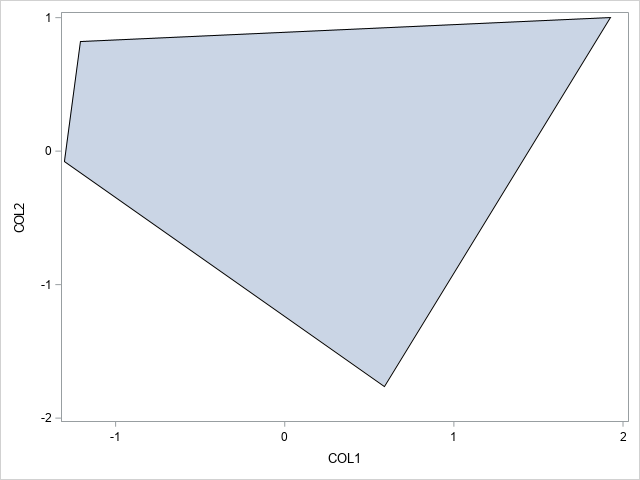
We explore the class of exchangeable Bernoulli distributions building on their geometrical structure. Exchangeable Bernoulli probability mass functions are points in a convex polytope and we have found analytical expressions for their extremal generators. The geometrical structure turns out to be crucial to simulate high dimensional and negatively correlated binary data. Furthermore, for a wide class of statistical indices and measures of a probability mass function we are able to find not only their sharp bounds in the class, but also their distribution across the class. Estimate and testing are also addressed.