PyDTS: A Python Package for Discrete-Time Survival (Regularized) Regression with Competing Risks

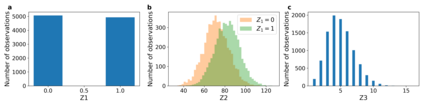
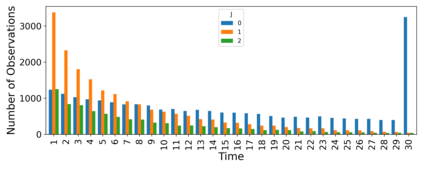
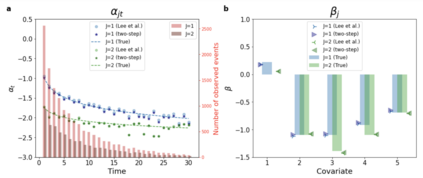
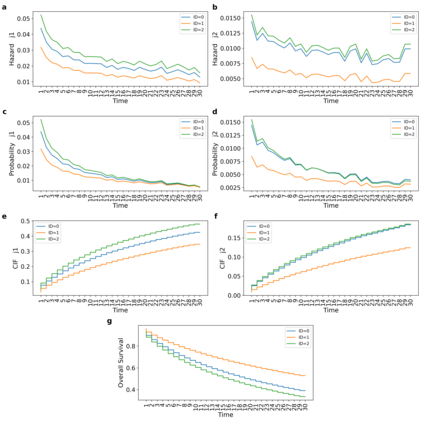
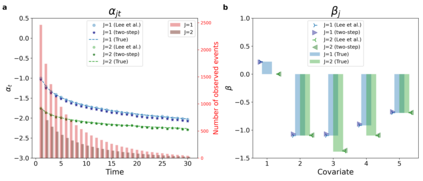
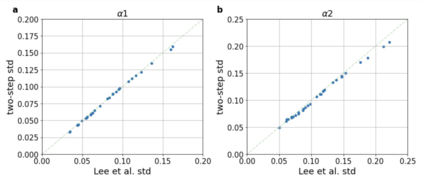
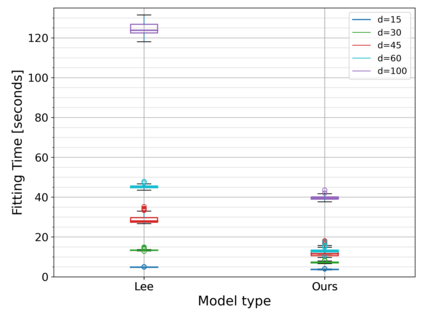
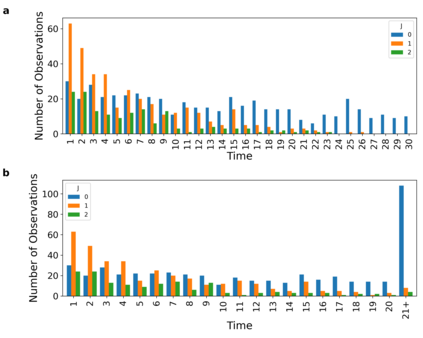
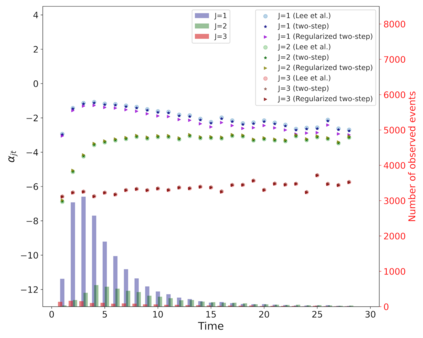
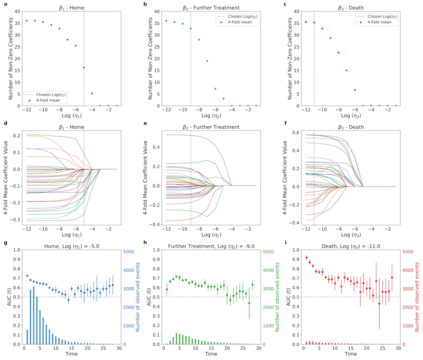
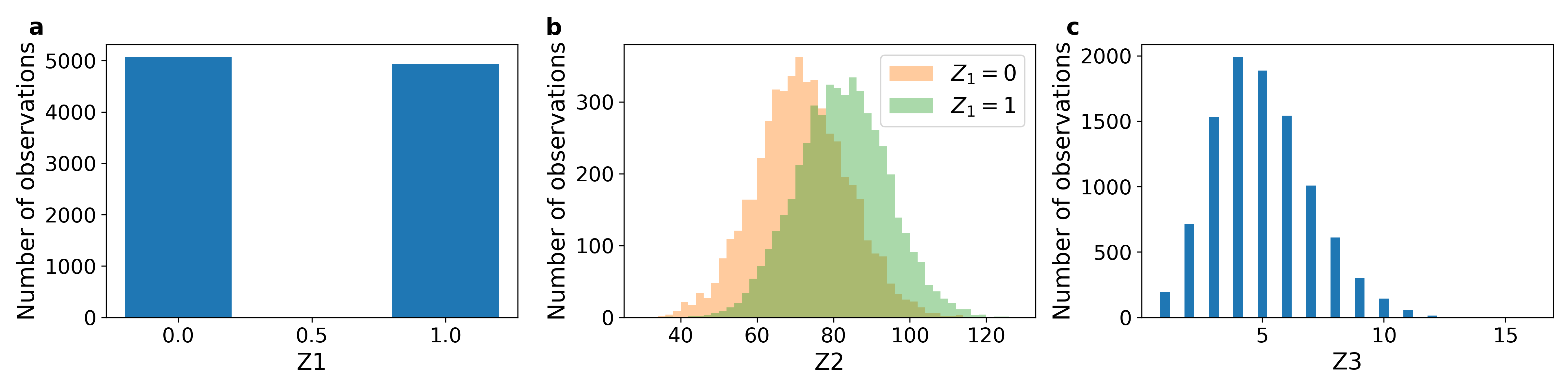
Time-to-event analysis (survival analysis) is used when the response of interest is the time until a pre-specified event occurs. Time-to-event data are sometimes discrete either because time itself is discrete or due to grouping of failure times into intervals or rounding off measurements. In addition, the failure of an individual could be one of several distinct failure types, known as competing risks (events). Most methods and software packages for survival regression analysis assume that time is measured on a continuous scale. It is well-known that naively applying standard continuous-time models with discrete-time data may result in biased estimators of the discrete-time models. The Python package PyDTS, for simulating, estimating and evaluating semi-parametric competing-risks models for discrete-time survival data, is introduced. The package implements a fast procedure that enables including regularized regression methods, such as LASSO and elastic net, among others. A simulation study showcases flexibility and accuracy of the package. The utility of the package is demonstrated by analysing the Medical Information Mart for Intensive Care (MIMIC) - IV dataset for prediction of hospitalization length of stay.