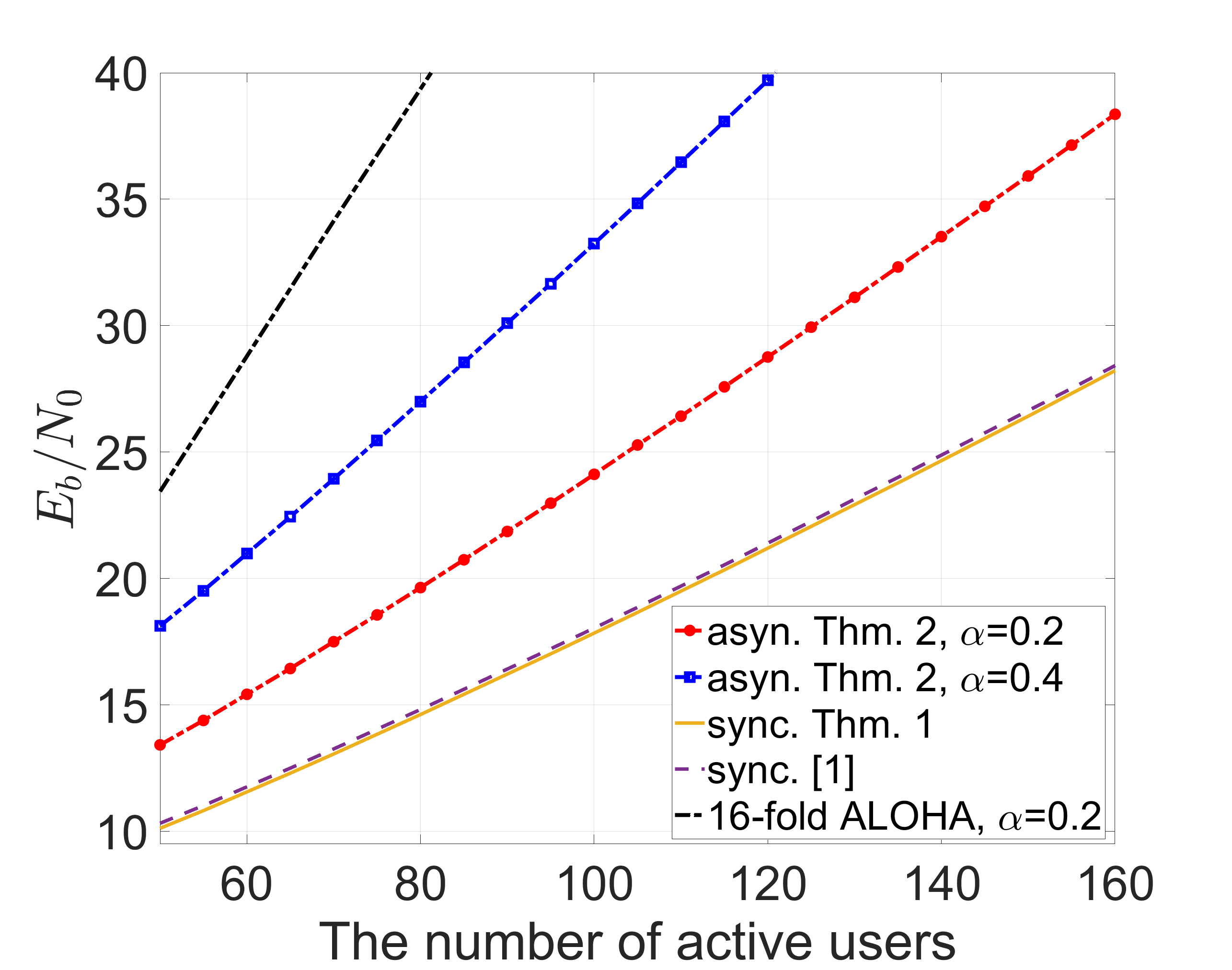
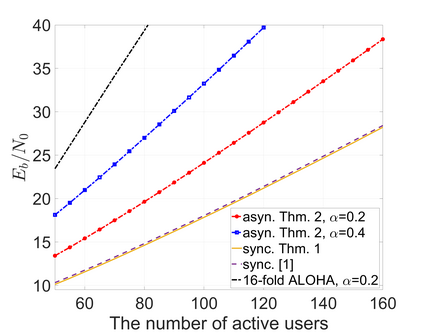
This work considers an asynchronous $\textsf{K}_\text{a}$-active-user unsourced multiple access channel (AUMAC) with the worst-case asynchronicity. The transmitted messages must be decoded within $n$ channel uses, while some codewords are not completely received due to asynchronicities. We consider a constraint of the largest allowed delay of the transmission. The AUMAC lacks the permutation-invariant property of the synchronous UMAC since different permutations of the same codewords with a fixed asynchronicity are distinguishable. Hence, the analyses require calculating all $2^{\textsf{K}_\text{a}}-1$ combinations of erroneously decoded messages. Moreover, transmitters cannot adapt the corresponding codebooks according to asynchronicity due to a lack of information on asynchronicities. To overcome this challenge, a uniform bound of the per-user probability of error (PUPE) is derived by investigating the worst-case of the asynchronous patterns with the delay constraint. Numerical results show the trade-off between the energy-per-bit and the number of active users for different delay constraints. In addition, although the asynchronous transmission reduces interference, the required energy-per-bit increases as the receiver decodes with incompletely received codewords, compared to the synchronous case.
翻译:暂无翻译