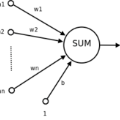
In this paper we show the possibility of creating and identifying the features of an artificial neural network (ANN) which consists of mathematical models of biological neurons. The FitzHugh--Nagumo (FHN) system is used as an example of model demonstrating simplified neuron activity. First, in order to reveal how biological neurons can be embedded within an ANN, we train the ANN with nonlinear neurons to solve a a basic image recognition problem with MNIST database; and next, we describe how FHN systems can be introduced into this trained ANN. After all, we show that an ANN with FHN systems inside can be successfully trained and its accuracy becomes larger. What has been done above opens up great opportunities in terms of the direction of analog neural networks, in which artificial neurons can be replaced by biological ones. \end{abstract}
翻译:在本文中,我们展示了创建和确定由生物神经数学模型组成的人工神经网络(ANN)特征的可能性。FitzHugh-Nagumo(FHN)系统被用作示范简化神经活动模型的范例。首先,为了揭示如何将生物神经元嵌入ANN,我们用非线性神经元对ANN进行了培训,以解决MNIST数据库中基本图像识别问题;然后,我们描述了如何将FHN系统引入这个经过培训的ANN。毕竟,我们证明一个带有FHN系统的ANN可以成功培训,其准确性会更大。以上已经做的工作为模拟神经网络的方向提供了巨大的机会,在这种网络中,人造神经元可以被生物神经取代。\ end{amptraty}