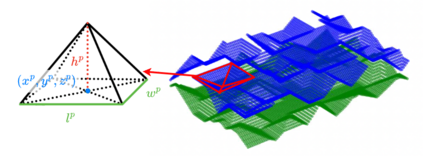
Recent advances of locomotion controllers utilizing deep reinforcement learning (RL) have yielded impressive results in terms of achieving rapid and robust locomotion across challenging terrain, such as rugged rocks, non-rigid ground, and slippery surfaces. However, while these controllers primarily address challenges underneath the robot, relatively little research has investigated legged mobility through confined 3D spaces, such as narrow tunnels or irregular voids, which impose all-around constraints. The cyclic gait patterns resulted from existing RL-based methods to learn parameterized locomotion skills characterized by motion parameters, such as velocity and body height, may not be adequate to navigate robots through challenging confined 3D spaces, requiring both agile 3D obstacle avoidance and robust legged locomotion. Instead, we propose to learn locomotion skills end-to-end from goal-oriented navigation in confined 3D spaces. To address the inefficiency of tracking distant navigation goals, we introduce a hierarchical locomotion controller that combines a classical planner tasked with planning waypoints to reach a faraway global goal location, and an RL-based policy trained to follow these waypoints by generating low-level motion commands. This approach allows the policy to explore its own locomotion skills within the entire solution space and facilitates smooth transitions between local goals, enabling long-term navigation towards distant goals. In simulation, our hierarchical approach succeeds at navigating through demanding confined 3D environments, outperforming both pure end-to-end learning approaches and parameterized locomotion skills. We further demonstrate the successful real-world deployment of our simulation-trained controller on a real robot.
翻译:暂无翻译