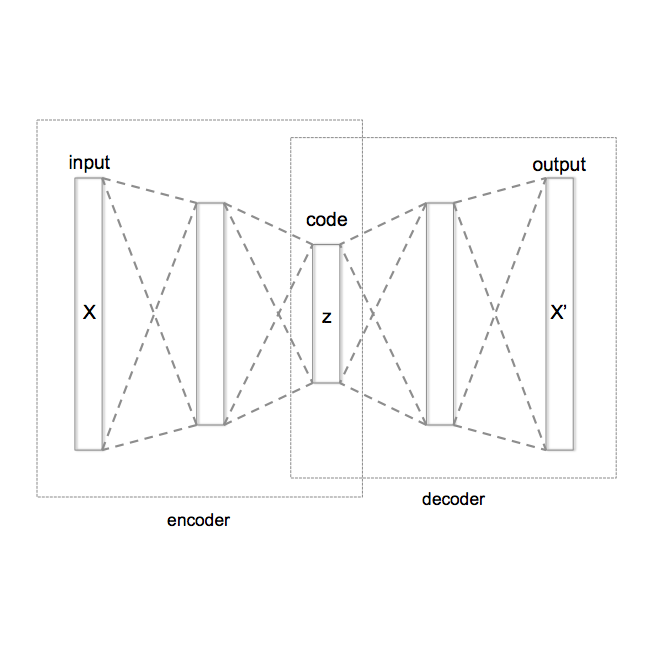
Variational auto-encoders are powerful probabilistic models in generative tasks but suffer from generating low-quality samples which are caused by the holes in the prior. We propose the Coupled Variational Auto-Encoder (C-VAE), which formulates the VAE problem as one of Optimal Transport (OT) between the prior and data distributions. The C-VAE allows greater flexibility in priors and natural resolution of the prior hole problem by enforcing coupling between the prior and the data distribution and enables flexible optimization through the primal, dual, and semi-dual formulations of entropic OT. Simulations on synthetic and real data show that the C-VAE outperforms alternatives including VAE, WAE, and InfoVAE in fidelity to the data, quality of the latent representation, and in quality of generated samples.
翻译:暂无翻译