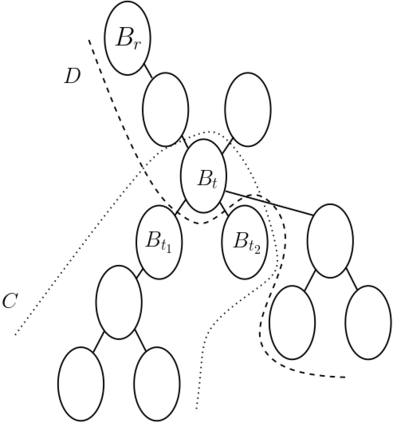
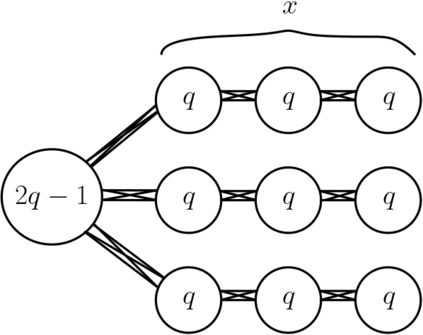
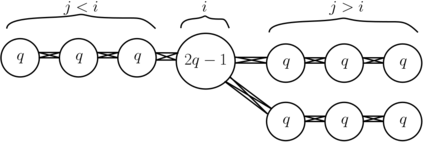
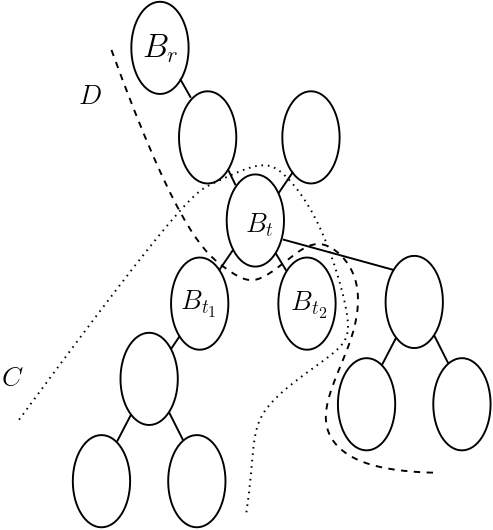
In a reduction sequence of a graph, vertices are successively identified until the graph has one vertex. At each step, when identifying $u$ and $v$, each edge incident to exactly one of $u$ and $v$ is coloured red. Bonnet, Kim, Thomass\'e and Watrigant [J. ACM 2022] defined the twin-width of a graph $G$ to be the minimum integer $k$ such that there is a reduction sequence of $G$ in which every red graph has maximum degree at most $k$. For any graph parameter $f$, we define the reduced $f$ of a graph $G$ to be the minimum integer $k$ such that there is a reduction sequence of $G$ in which every red graph has $f$ at most $k$. Our focus is on graph classes with bounded reduced bandwidth, which implies and is stronger than bounded twin-width (reduced maximum degree). We show that every proper minor-closed class has bounded reduced bandwidth, which is qualitatively stronger than an analogous result of Bonnet et al.\ for bounded twin-width. In many instances, we also make quantitative improvements. For example, all previous upper bounds on the twin-width of planar graphs were at least $2^{1000}$. We show that planar graphs have reduced bandwidth at most $466$ and twin-width at most $583$. Our bounds for graphs of Euler genus $\gamma$ are $O(\gamma)$. Lastly, we show that fixed powers of graphs in a proper minor-closed class have bounded reduced bandwidth (irrespective of the degree of the vertices). In particular, we show that map graphs of Euler genus $\gamma$ have reduced bandwidth $O(\gamma^4)$. Lastly, we separate twin-width and reduced bandwidth by showing that any infinite class of expanders excluding a fixed complete bipartite subgraph has unbounded reduced bandwidth, while there are bounded-degree expanders with twin-width at most 6.
翻译:在图形的递减序列中,顶点会连续被识别,直到图形有一个顶点。每一步,在确定美元和美元时,每个边点事件都会被确定为1美元和1美元,美元是彩色红色。Bonnet、Kim、Thomas\'e和Watrigant[J.ACM 2022]定义了图形的双维(G$是最小整数的整数,也就是说每个红色平面的平面有最高水平的G$。对于任何图形参数,我们确定一个G$的减价是最小整数美元,美元是最低整数。Bonet 和alvilate $。我们的平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面平面,我们平面平面平面平面平面平面平面平面平面平面平面平面平面,我们平面平面平面平面平面平面平面平面平面平面平面平面。