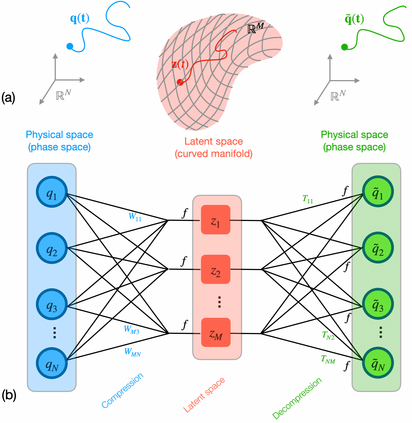
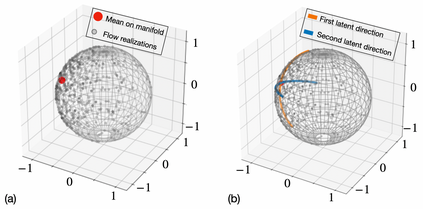
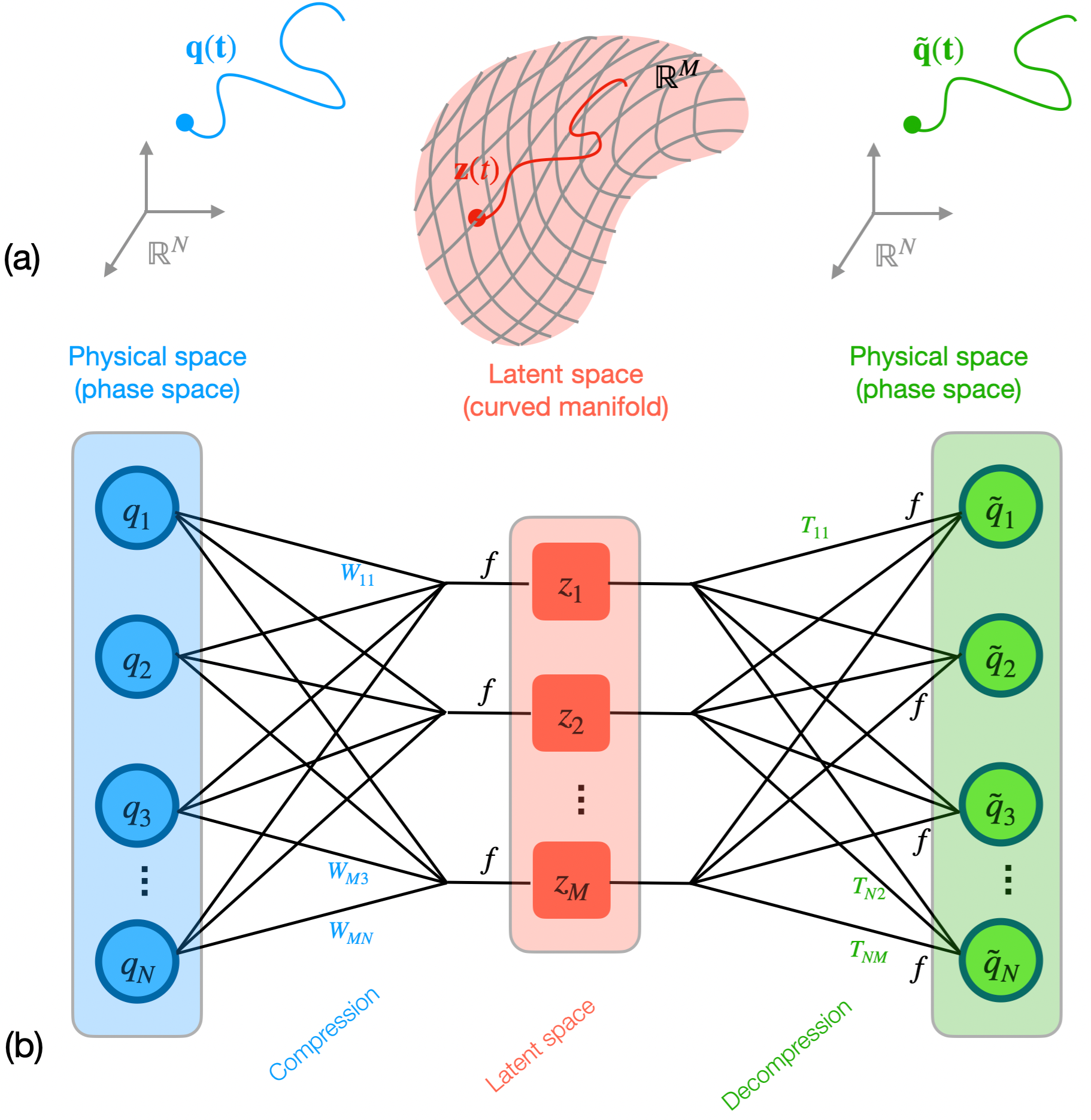
The dynamics of a turbulent flow tend to occupy only a portion of the phase space at a statistically stationary regime. From a dynamical systems point of view, this portion is the attractor. The knowledge of the turbulent attractor is useful for two purposes, at least: (i) We can gain physical insight into turbulence (what is the shape and geometry of the attractor?), and (ii) it provides the minimal number of degrees of freedom to accurately describe the turbulent dynamics. Autoencoders enable the computation of an optimal latent space, which is a low-order representation of the dynamics. If properly trained and correctly designed, autoencoders can learn an approximation of the turbulent attractor, as shown by Doan, Racca and Magri (2022). In this paper, we theoretically interpret the transformations of an autoencoder. First, we remark that the latent space is a curved manifold with curvilinear coordinates, which can be analyzed with simple tools from Riemann geometry. Second, we characterize the geometrical properties of the latent space. We mathematically derive the metric tensor, which provides a mathematical description of the manifold. Third, we propose a method -- proper latent decomposition (PLD) -- that generalizes proper orthogonal decomposition of turbulent flows on the autoencoder latent space. This decomposition finds the dominant directions in the curved latent space. This theoretical work opens up computational opportunities for interpreting autoencoders and creating reduced-order models of turbulent flows.
翻译:动荡流的动态在统计性固定状态下只能占据部分阶段空间。 从动态系统的观点看, 这部分是吸引器。 动荡吸引器的知识对以下两个目的至少有用:(一) 我们可以从物理上洞察动荡( 吸引器的形状和几何? ), (二) 它为准确描述动荡动态提供了最小的自由度。 自动进化器能够计算出一个最佳的潜潜伏空间, 这是一种动态的低顺序代表器。 如果经过适当培训和正确设计, 自动进化器可以学习动荡吸引器的近似, 如 Doan、 Racca 和 Magri (2022年) 所示 。 在本文中, 我们可以从理论上解释一个自动变形变形器的变形。 首先, 我们指出, 潜伏空间是一个曲线的曲线, 可以使用来自 Riemann 几何测量的简单工具加以分析。 其次, 我们用数学来描述暗层空间的地基值变值特性。 我们从数学角度推导出调调的直径阵列空间流,, 也就是地变变变形法, 。