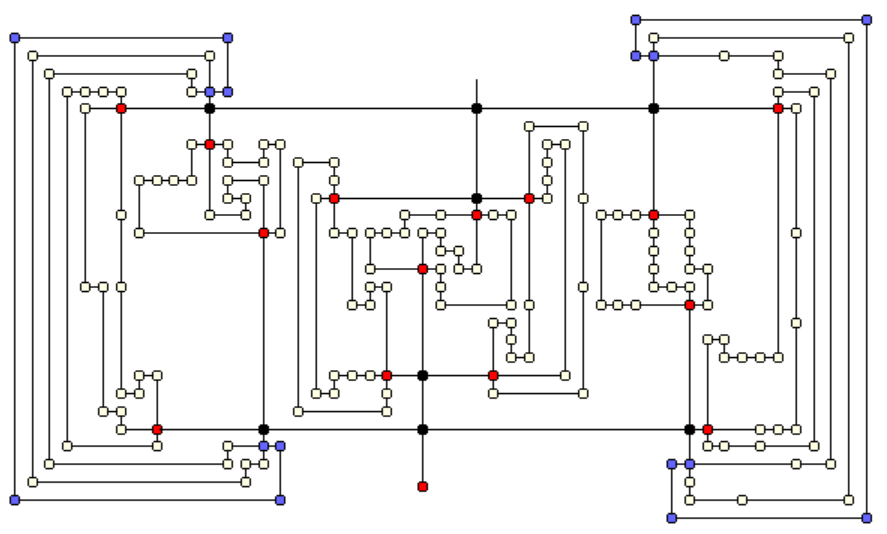
We study the long-standing open problem of efficiently testing rectilinear planarity of series-parallel graphs (SP-graphs) in the variable embedding setting. A key ingredient behind the design of a linear-time testing algorithm for SP-graphs of vertex-degree at most three is that one can restrict the attention to a constant number of ``rectilinear shapes'' for each series or parallel component. To formally describe these shapes the notion of spirality can be used. This key ingredient no longer holds for SP-graphs with vertices of degree four, as we prove a logarithmic lower bound on the spirality of their components. The bound holds even for the independent-parallel SP-graphs, in which no two parallel components share a pole. Nonetheless, by studying the spirality properties of the independent-parallel SP-graphs, we are able to design a linear-time rectilinear planarity testing algorithm for this graph family.
翻译:我们研究了在变量嵌入设置中有效测试系列单线图(SP-graphs)的直线性平面图(SP-graphs)长期存在的开放问题。设计SP顶部分层图的线性时间测试算法背后的一个关键要素是,人们可以将注意力限制在每个序列或平行组件的“正线形形状”的恒定数上。要正式描述这些形状,可以使用螺旋性概念。这个关键成分不再用于具有四度顶部的 SP-graphs(SP-graphs),因为我们证明其组件的螺旋性对数约束较低。即使对于独立的单线性SP-graphs, 也没有两个平行组件共享一个极。然而,通过研究独立的单线SP-graphs的螺旋性特性,我们可以为这个图形家族设计一个线性直线性对线性平线性平线性测算算算法。