Exponentially Fitted Finite Difference Approximation for Singularly Perturbed Fredholm Integro-Differential Equation

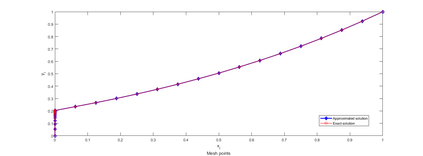
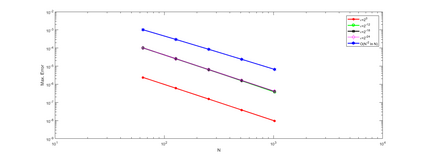
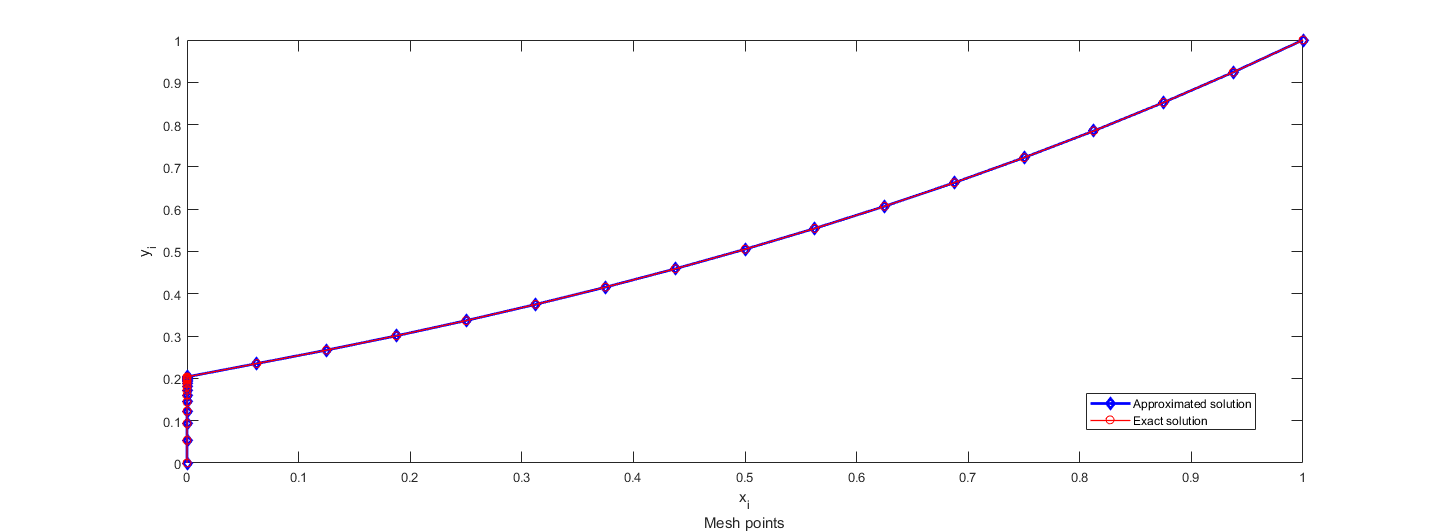
In this paper, we concentrate on solving second-order singularly perturbed Fredholm integro-differential equations (SPFIDEs). It is well known that solving these equations analytically is a challenging endeavor because of the presence of boundary and interior layers within the domain. To overcome these challenges, we develop a fitted second-order difference scheme that can capture the layer behavior of the solution accurately and efficiently, which is again, based on the integral identities with exponential basis functions, the composite trapezoidal rule, and an appropriate interpolating quadrature rules with the remainder terms in the integral form on a piecewise uniform mesh. Hence, our numerical method acts as a superior alternative to the existing methods in the literature. Further, using appropriate techniques in error analysis the scheme's convergence and stability have been studied in the discrete max norm. We have provided necessary experimental evidence that corroborates the theoretical results with a high degree of accuracy.