A tensor bidiagonalization method for higher-order singular value decomposition with applications

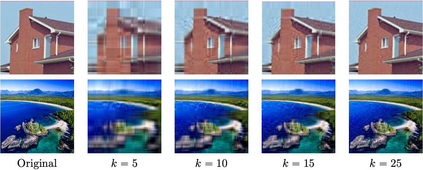
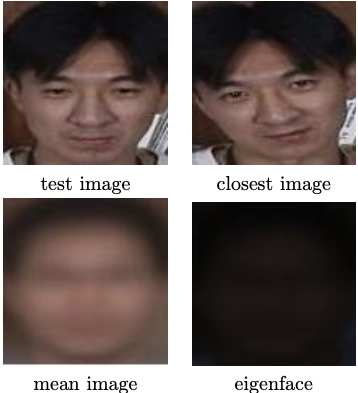
The need to know a few singular triplets associated with the largest singular values of third-order tensors arises in data compression and extraction. This paper describes a new method for their computation using the t-product. Methods for determining a couple of singular triplets associated with the smallest singular values also are presented. The proposed methods generalize available restarted Lanczos bidiagonalization methods for computing a few of the largest or smallest singular triplets of a matrix. The methods of this paper use Ritz and harmonic Ritz lateral slices to determine accurate approximations of the largest and smallest singular triplets, respectively. Computed examples show applications to data compression and face recognition.