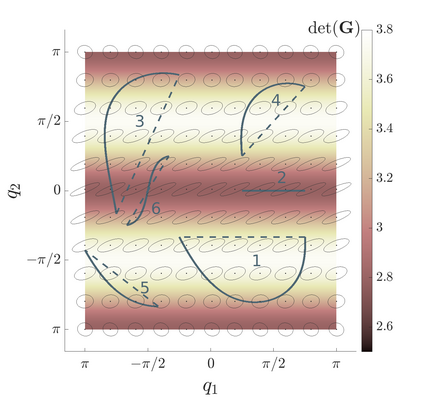
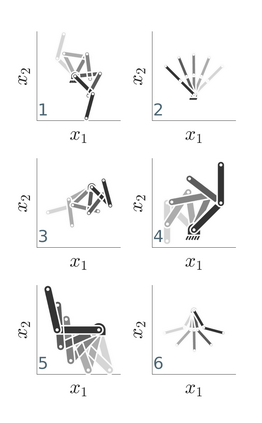
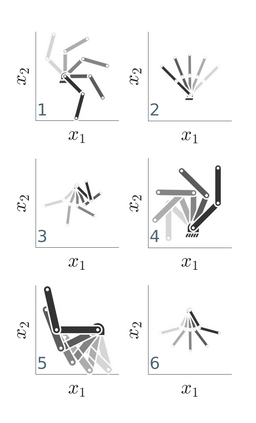
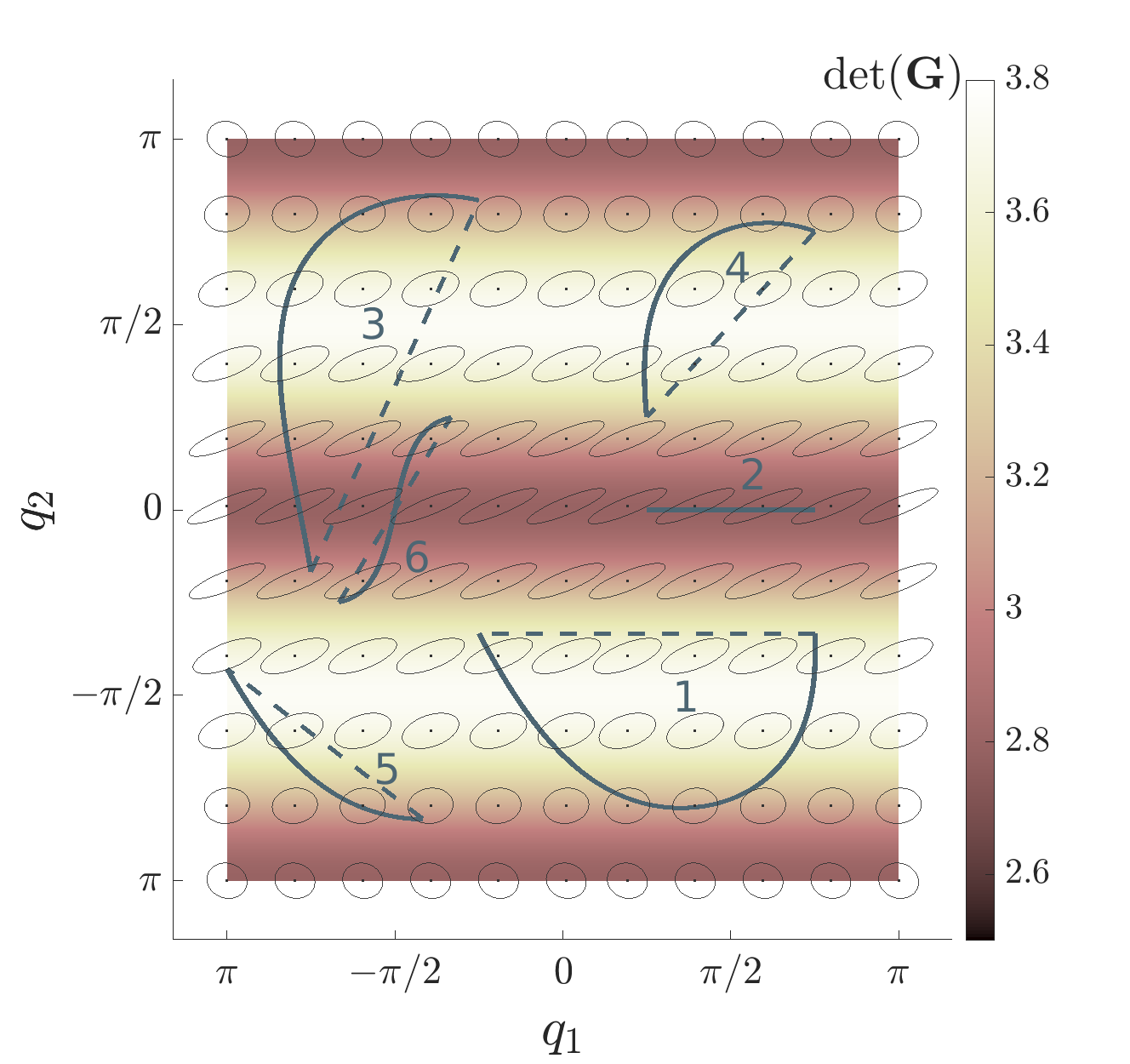
Riemannian geometry is a mathematical field which has been the cornerstone of revolutionary scientific discoveries such as the theory of general relativity. Despite early uses in robot design and recent applications for exploiting data with specific geometries, it mostly remains overlooked in robotics. With this blue sky paper, we argue that Riemannian geometry provides the most suitable tools to analyze and generate well-coordinated, energy-efficient motions of robots with many degrees of freedom. Via preliminary solutions and novel research directions, we discuss how Riemannian geometry may be leveraged to design and combine physically-meaningful synergies for robotics, and how this theory also opens the door to coupling motion synergies with perceptual inputs.
翻译:Riemannian 几何学是一个数学领域,它一直是革命性科学发现的基石,例如一般相对论理论。尽管机器人设计早期使用和最近应用机器人设计及利用特定几何数据的应用,但在机器人中仍然大都被忽视。我们争辩说,Riemannian 几何学提供了最合适的工具,可以分析和产生协调良好、节能且自由程度多的机器人运动。我们讨论了初步解决方案和新研究方向,我们讨论了如何利用Riemann 几何学来设计和结合机器人的物理利益协同作用,以及这一理论如何打开了将运动协同作用与概念投入结合起来的大门。