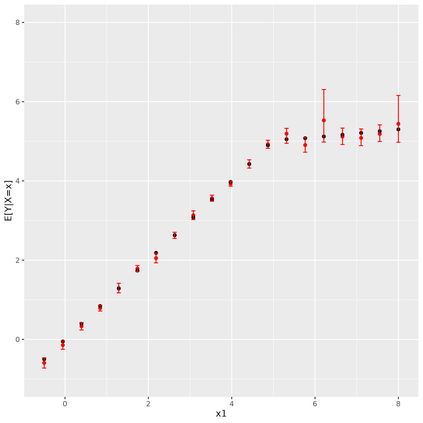
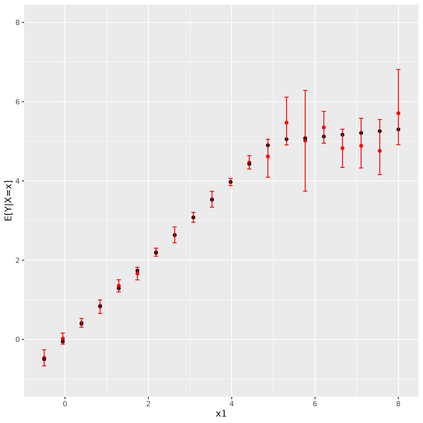
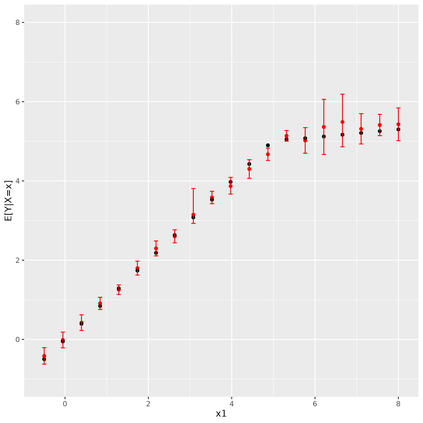
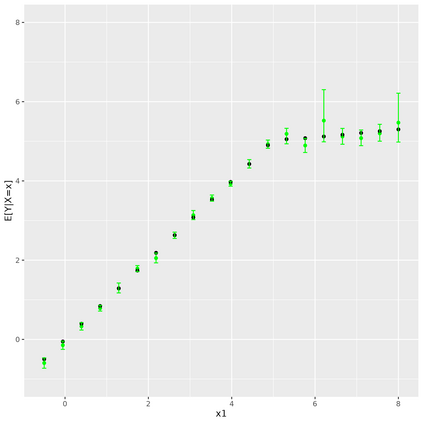
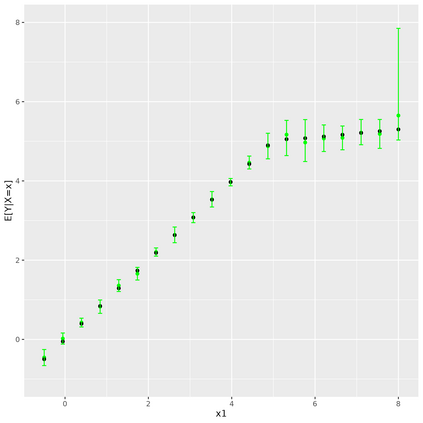
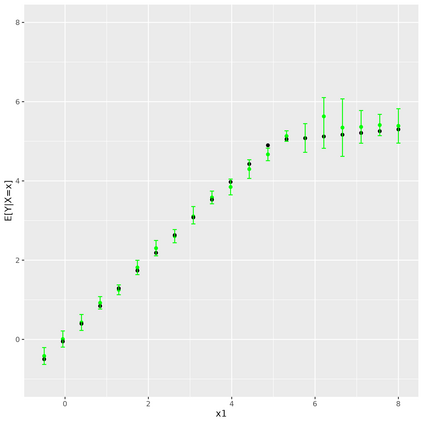
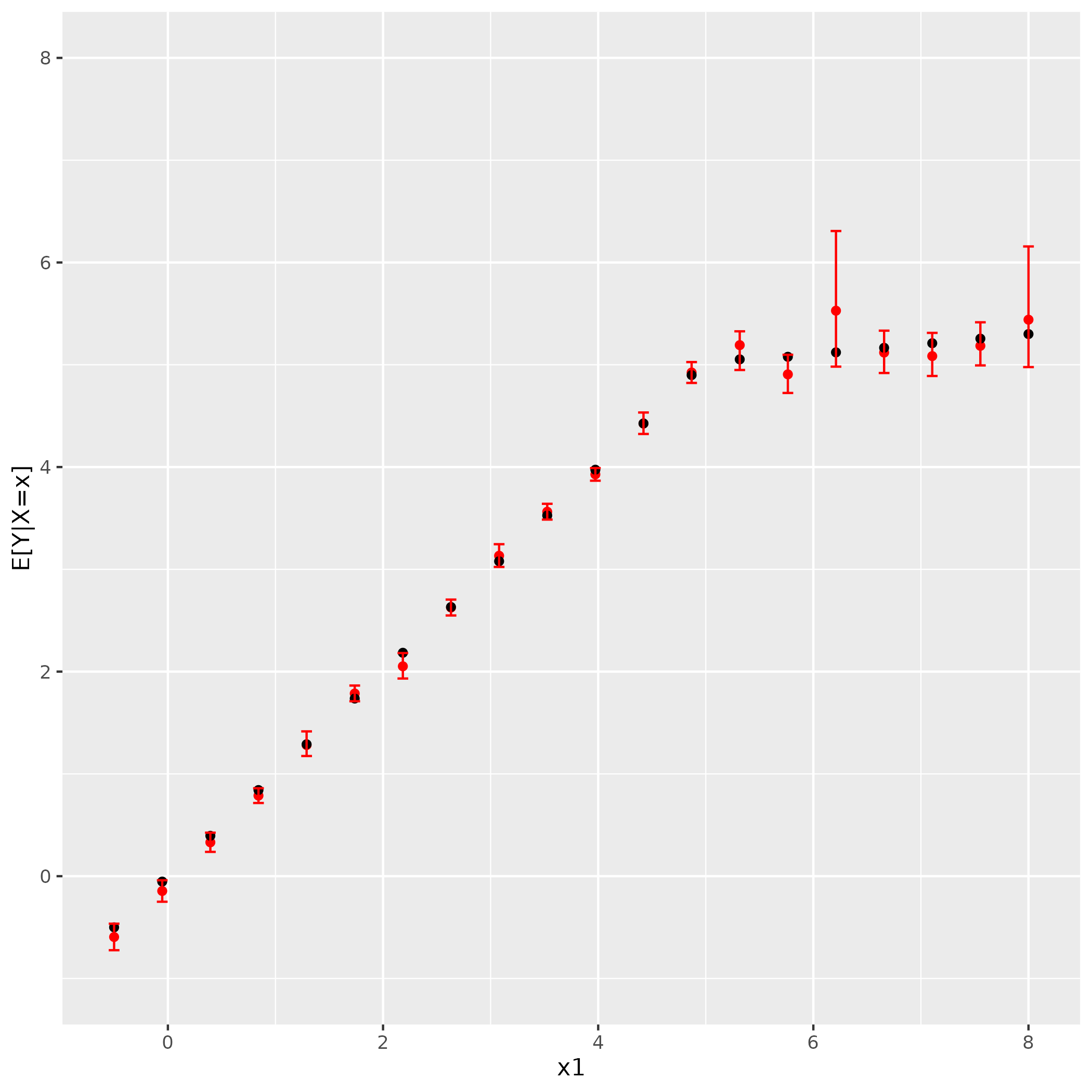
Enriched Dirichlet process mixture (EDPM) models are Bayesian nonparametric models which can be used for nonparametric regression and conditional density estimation and which overcome a key disadvantage of jointly modeling the response and predictors as a Dirichlet process mixture (DPM) model: when there is a large number of predictors, the clusters induced by the DPM will be overwhelmingly determined by the predictors rather than the response. A truncation approximation to a DPM allows a blocked Gibbs sampling algorithm to be used rather than a Polya urn sampling algorithm. The blocked Gibbs sampler offers potential improvement in mixing. The truncation approximation also allows for implementation in standard software ($\textit{rjags}$ and $\textit{rstan}$). In this paper we introduce an analogous truncation approximation for an EDPM. We show that with sufficiently large truncation values in the approximation of the EDP prior, a precise approximation to the EDP is available. We verify that the truncation approximation and blocked Gibbs sampler with minimum truncation values that obtain adequate error bounds achieve similar accuracy to the truncation approximation and blocked Gibbs sampler with large truncation values using a simulated example. Further, we use the simulated example to show that the blocked Gibbs sampler improves upon the mixing in the Polya urn sampler, especially as the number of covariates increases.
翻译:暂无翻译