A Region-based Randers Geodesic Approach for Image Segmentation

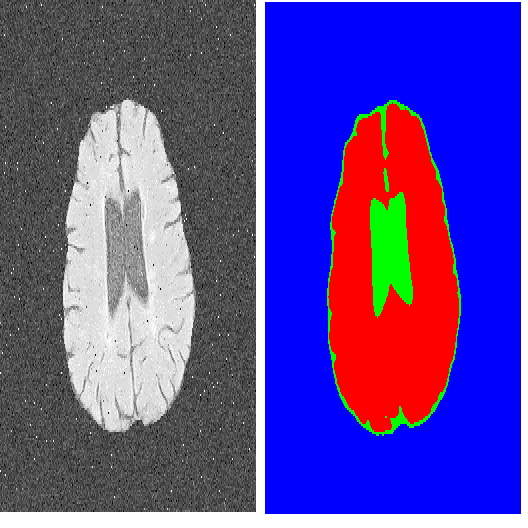
The geodesic model based on the eikonal partial differential equation (PDE) has served as a fundamental tool for the applications of image segmentation and boundary detection in the past two decades. However, the existing approaches commonly only exploit the image edge-based features for computing minimal geodesic paths, potentially limiting their performance in complicated segmentation situations. In this paper, we introduce a new variational image segmentation model based on the minimal geodesic path framework and the eikonal PDE, where the region-based appearance term that defines then regional homogeneity features can be taken into account for estimating the associated minimal geodesic paths. This is done by constructing a Randers geodesic metric interpretation of the region-based active contour energy functional. As a result, the minimization of the active contour energy functional is transformed into finding the solution to the Randers eikonal PDE. We also suggest a practical interactive image segmentation strategy, where the target boundary can be delineated by the concatenation of several piecewise geodesic paths. We invoke the Finsler variant of the fast marching method to estimate the geodesic distance map, yielding an efficient implementation of the proposed region-based Randers geodesic model for image segmentation. Experimental results on both synthetic and real images exhibit that our model indeed achieves encouraging segmentation performance.