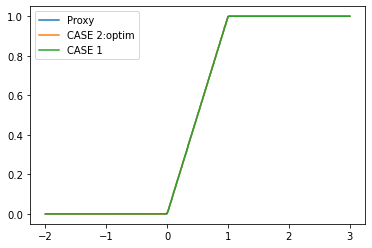
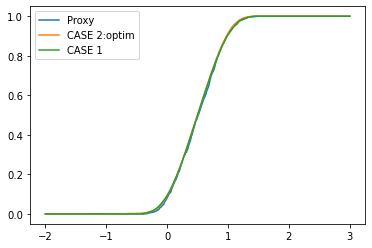
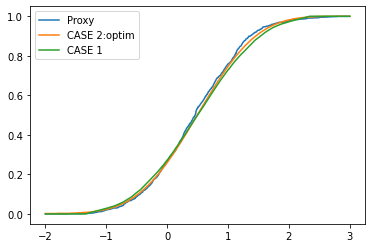
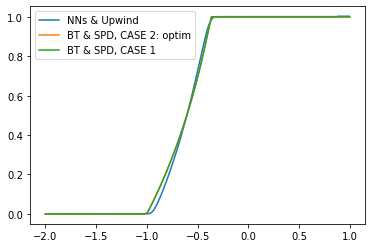
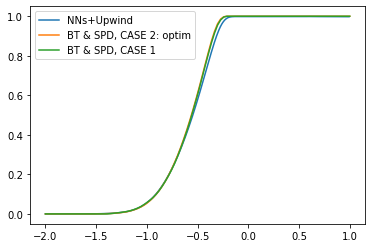
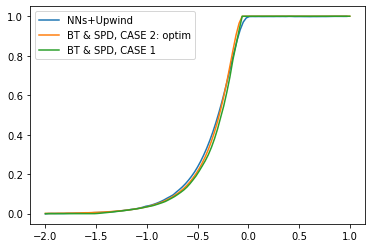
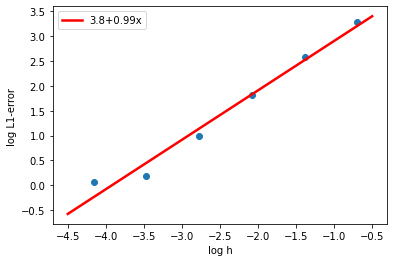
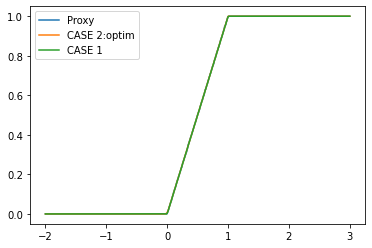
We study an implementation of the theoretical splitting scheme introduced in [Chassagneux and Yang, 2022] for singular FBSDEs [Carmona and Delarue 2013] and their associated quasi-linear degenerate PDEs. The fully implementable algorithm is based on particles approximation of the transport operator and tree like approximation of the diffusion operator appearing in the theoretical splitting. We prove the convergence with a rate of our numerical method under some reasonable conditions on the coefficients functions. This validates a posteriori some numerical results obtained in [Chassagneux and Yang, 2022]. We conclude the paper with a numerical section presenting various implementations of the algorithm and discussing their efficiency in practice.
翻译:我们研究了在[Chassagneux和Yang,2022年]对单一的FBSDEs[Carmona和Delarue 2013]及其相关的准线性退化的PDEs实行的理论分解计划的实施情况。完全可实施的算法基于运输运营商的粒子近似值和树像理论分解中出现的扩散运营商的近似值。我们证明,在系数函数的某些合理条件下,我们的数字方法的速率与我们的数字方法的速率趋同。这证实了在[Chassagneux和Yang,2022年]中得出的一些事后数字结果。我们用一个数字部分来结束该文件,介绍算法的各种实施情况并讨论其实际效率。