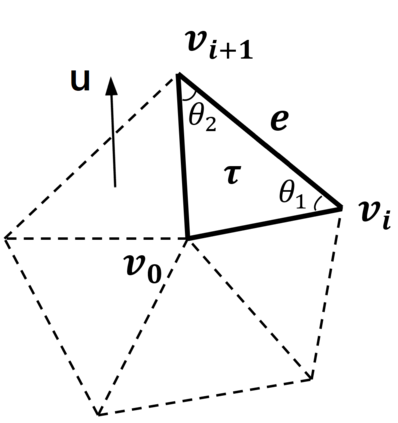
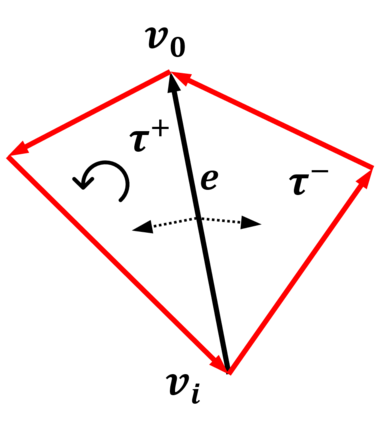
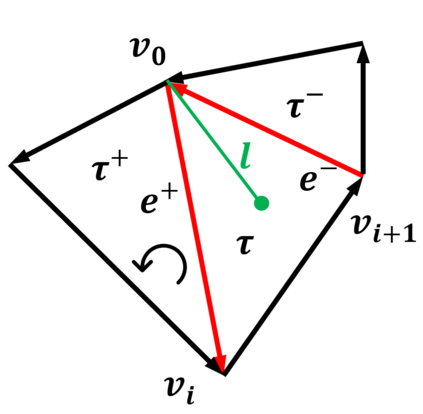
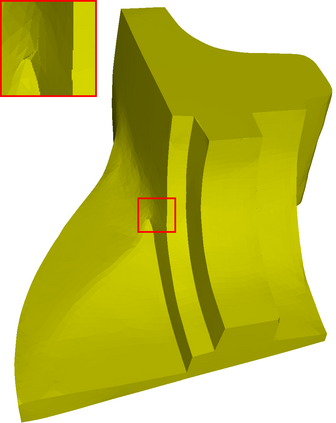
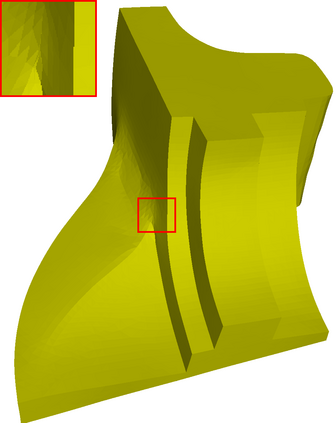
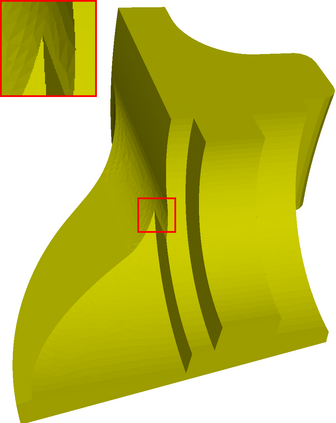
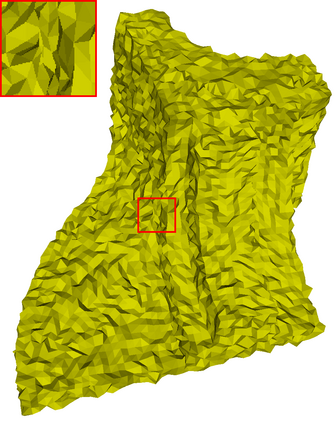
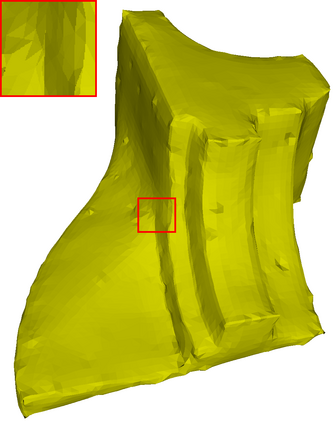
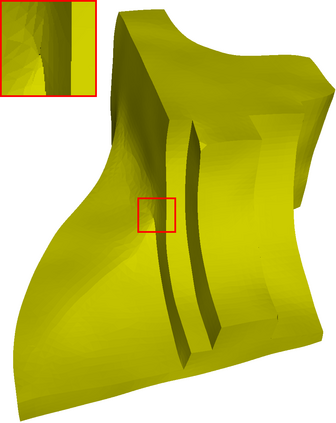
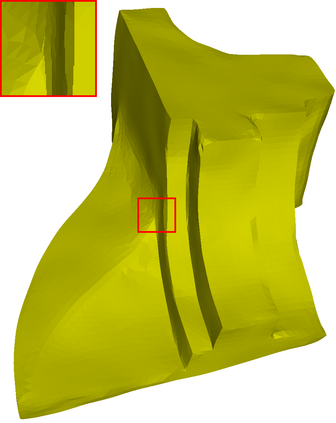
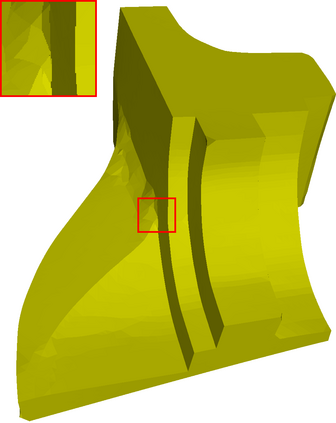
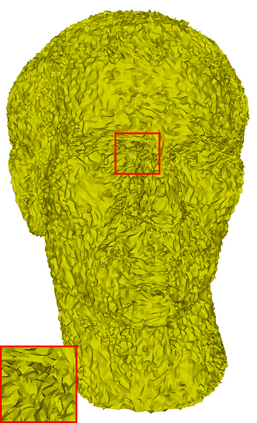
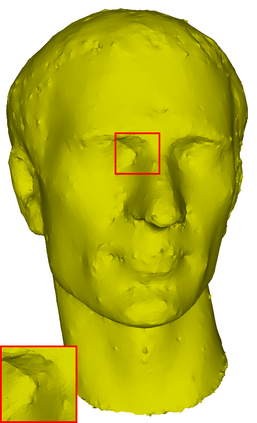
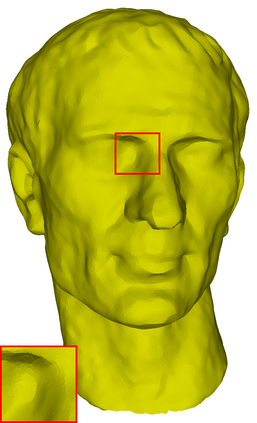
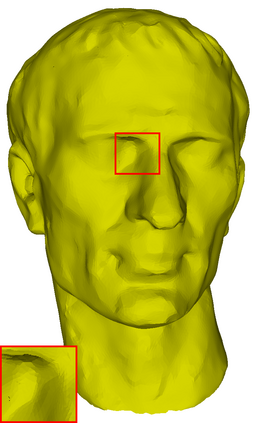
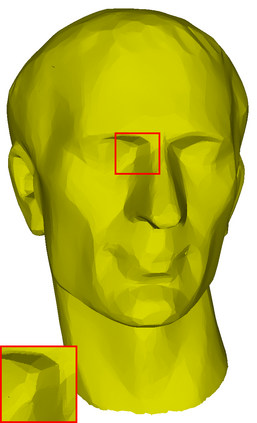
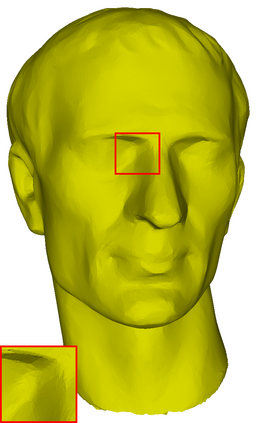
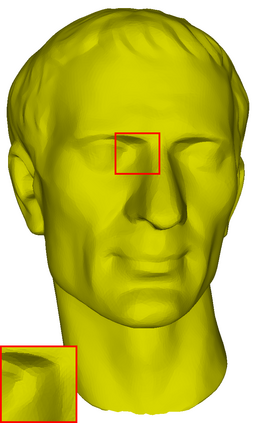
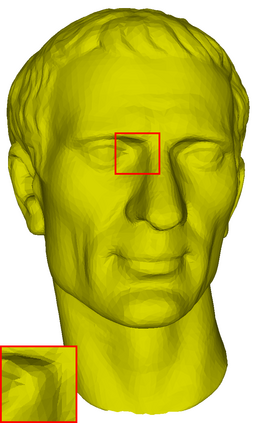
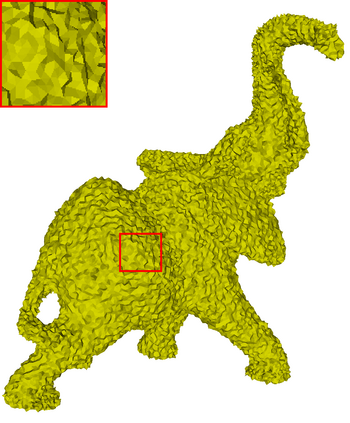
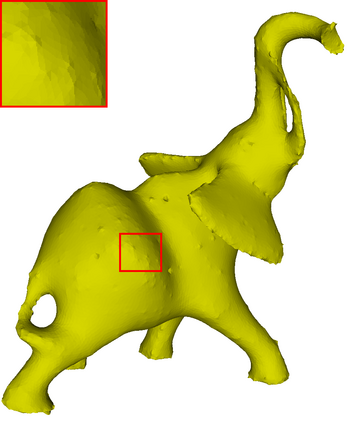
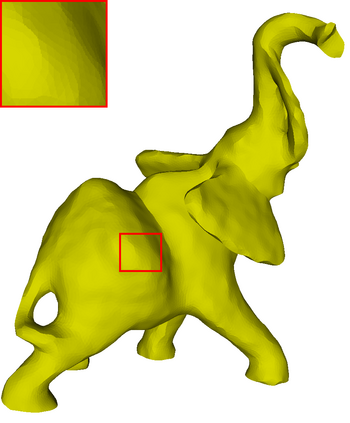
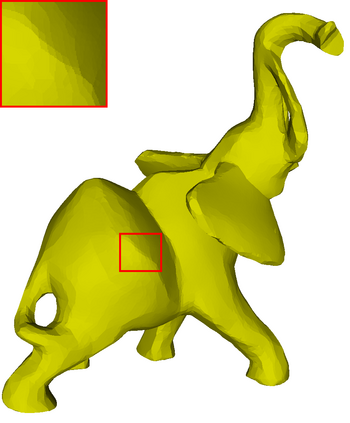
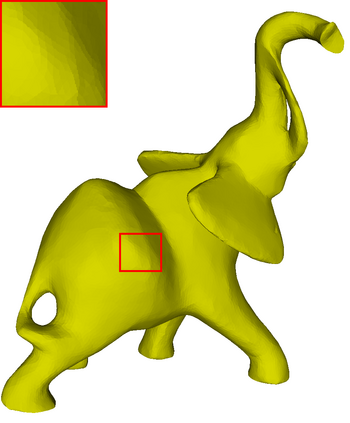
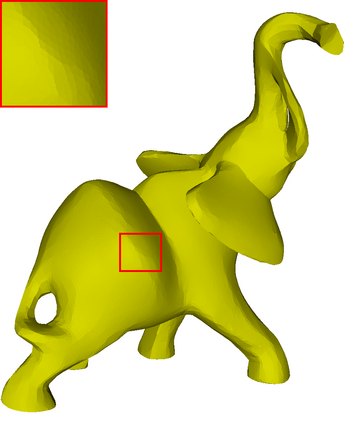
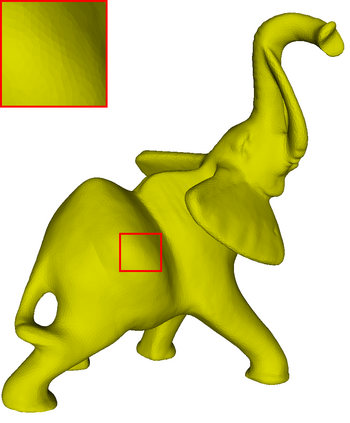
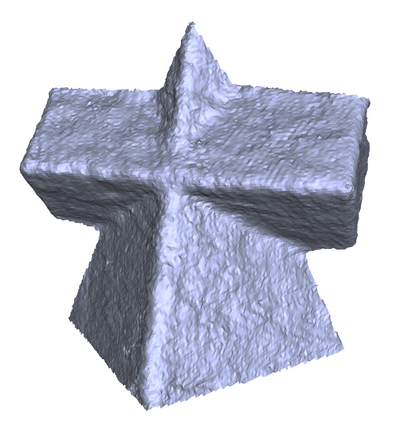
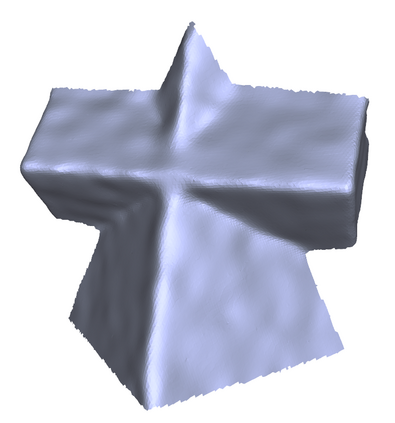
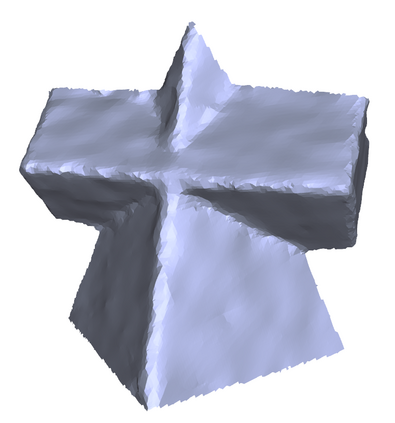
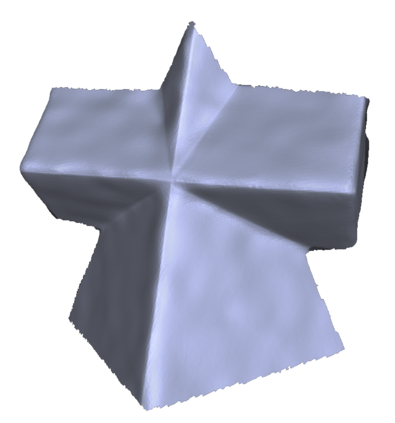
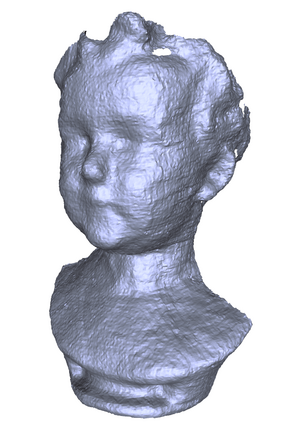
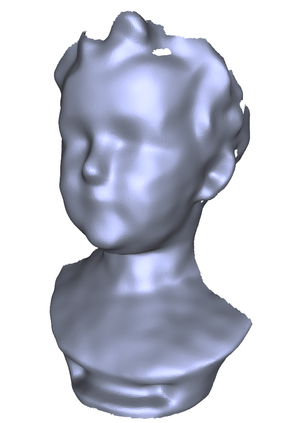
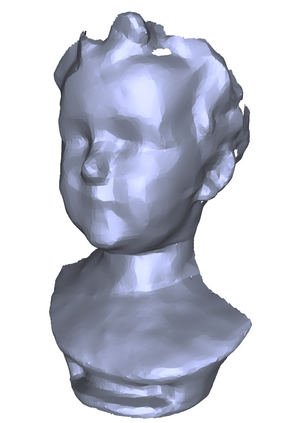
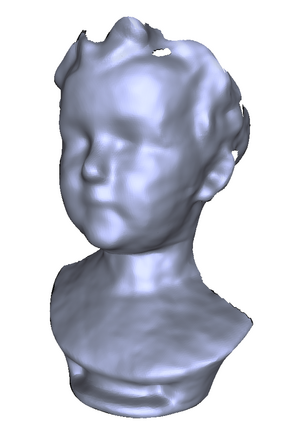
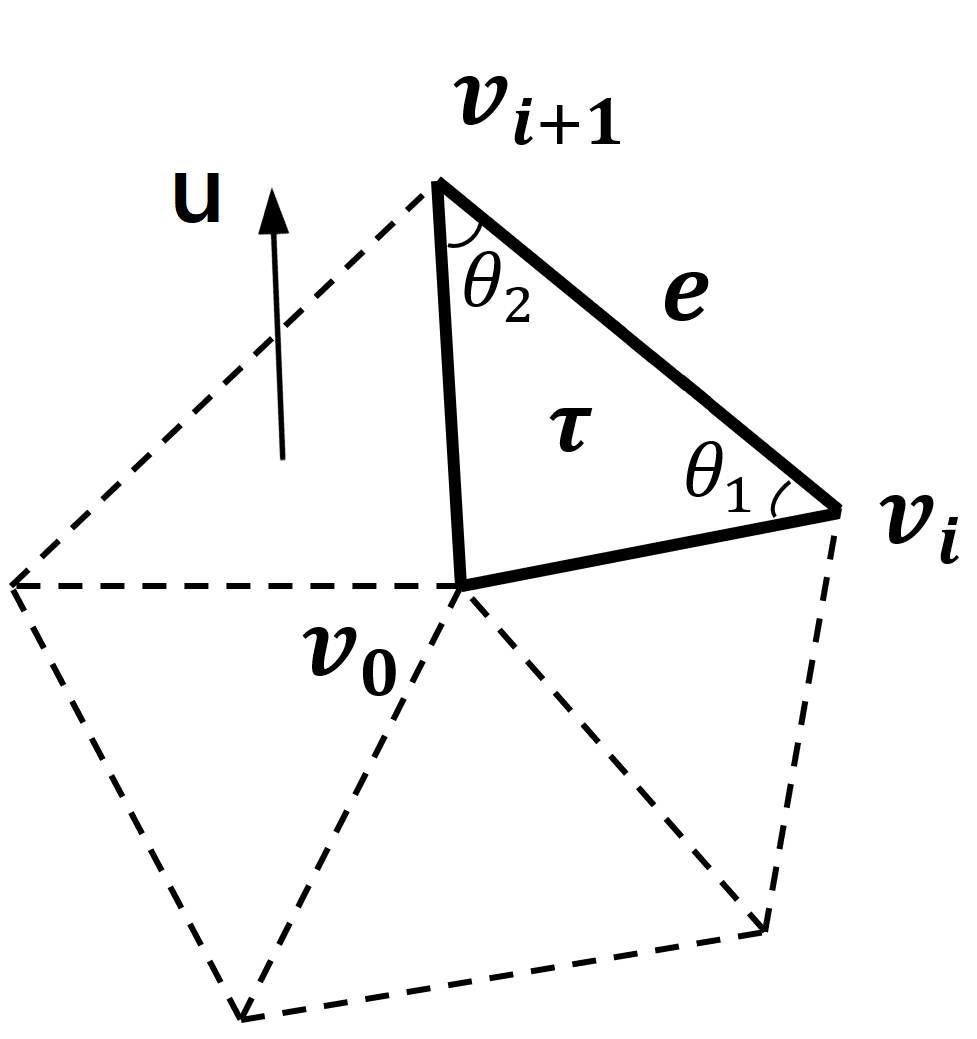
We present a semi-sparsity model for 3D triangular mesh denoising, which is motivated by the success of semi-sparsity regularization in image processing applications. We demonstrate that such a regularization model can be also applied for graphic processing and gives rise to similar simultaneous-fitting results in preserving sharp features and piece-wise smoothing surfaces. Specifically, we first describe the piecewise constant function spaces associated with the differential operators on triangular meshes and then show how to extend the semi-sparsity model to meshes denoising. To verify its effectiveness, we present an efficient iterative algorithm based on the alternating direction method of multipliers (ADMM) technique and show the experimental results on synthetic and real scanning data against the state-of-the-arts both visually and quantitatively.
翻译:暂无翻译