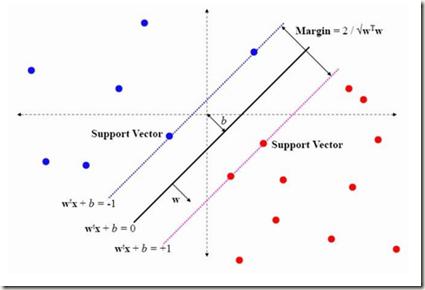
In this paper, we study the splitting method based on alternating direction method of multipliers for support vector machine in reproducing kernel Hilbert space with lower semi-continuous loss function. If the loss function is lower semi-continuous and subanalytic, we use the Kurdyka-Lojasiewicz inequality to show that the iterative sequence induced by the splitting method globally converges to a stationary point. The numerical experiments also demonstrate the effectiveness of the splitting method.
翻译:在本文中,我们研究基于支持矢量机器的乘数交替方向法的分解法,以生成内核的半连续性损失功能来复制内核的Hilbert空间。如果损失功能是较低的半连续性和亚分析性,我们使用Kurdyka-Lojasiewicz的不平等性来显示分解法在全球诱发的迭接序列会与固定点相融合。数字实验还证明了分解方法的有效性。
相关内容
专知会员服务
36+阅读 · 2019年10月17日
Arxiv
0+阅读 · 2022年10月4日