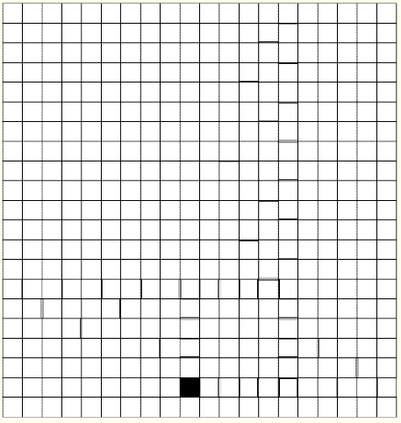
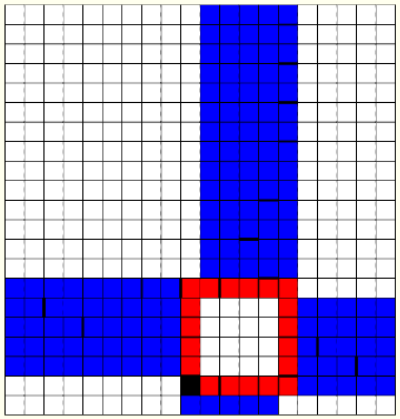
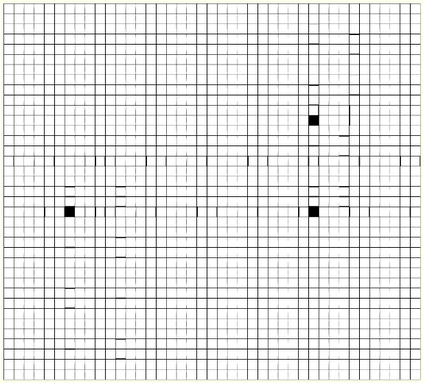
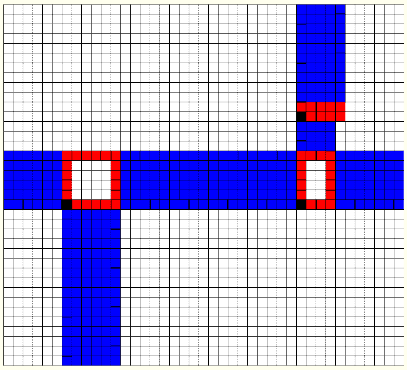
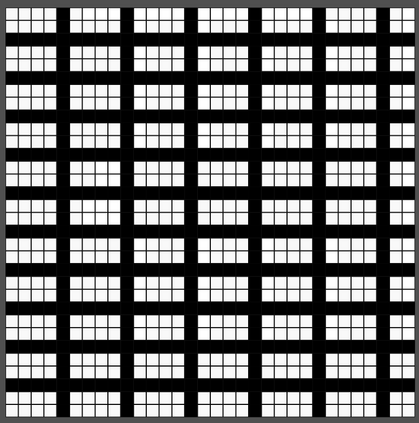
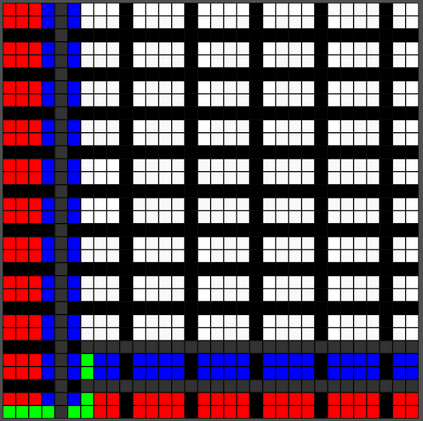
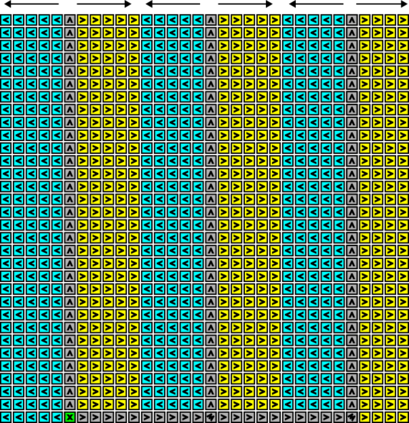
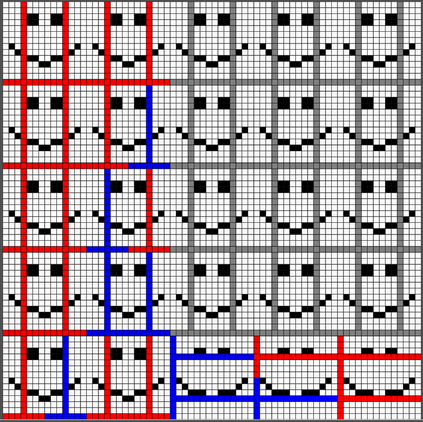
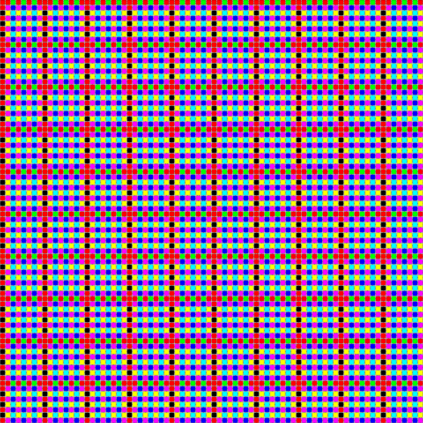
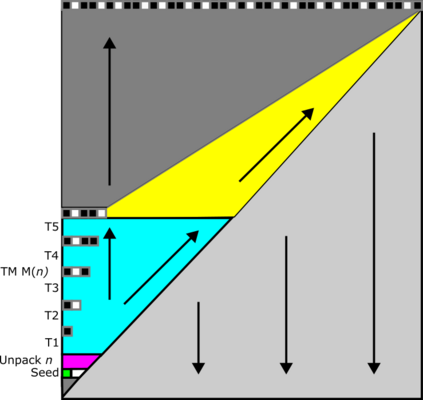
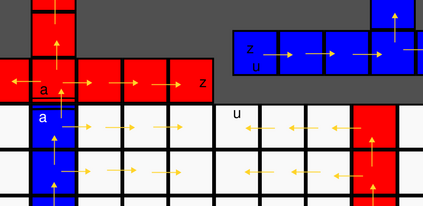
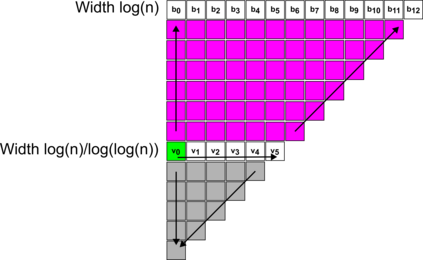
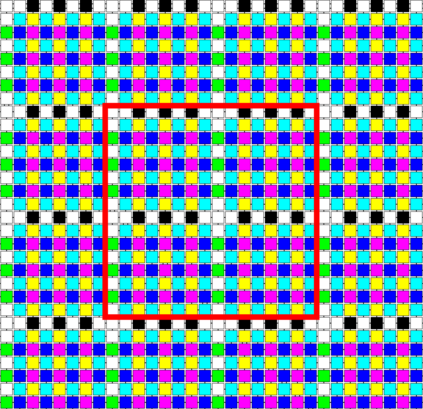
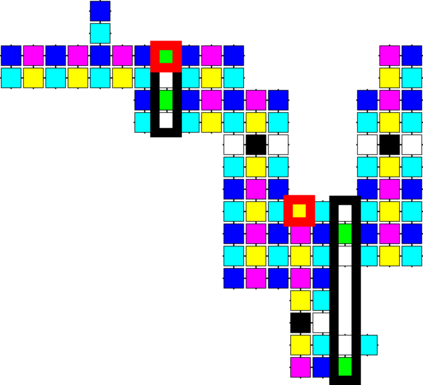
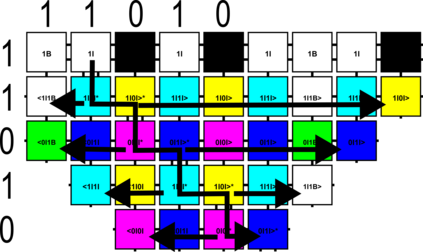
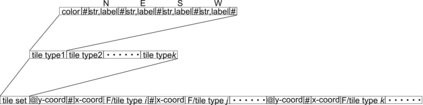
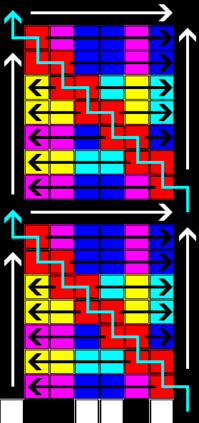
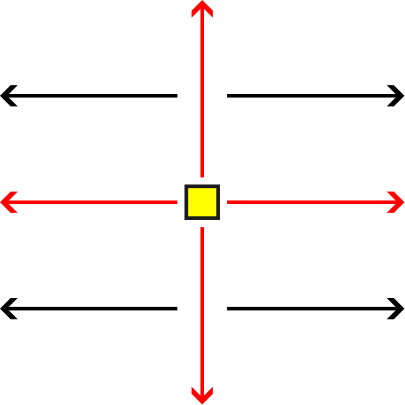
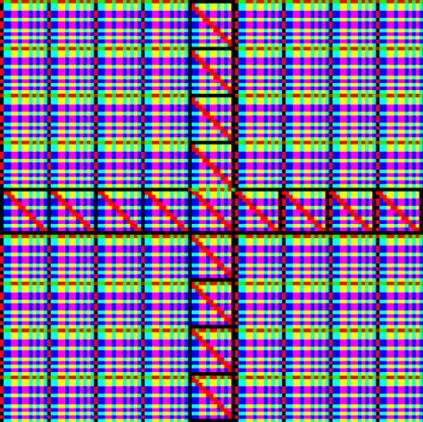
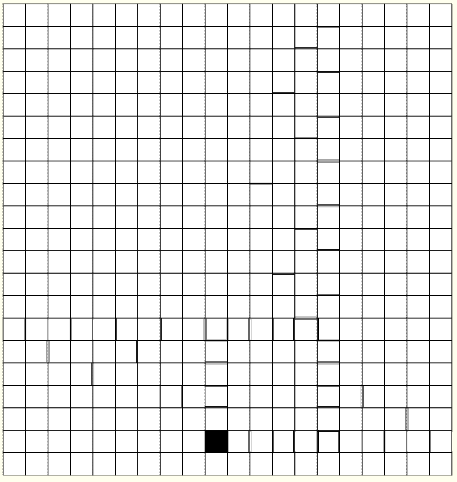
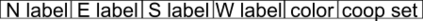In the abstract Tile Assembly Model, self-assembling systems consisting of tiles of different colors can form structures on which colored patterns are ``painted.'' We explore the complexity, in terms of the numbers of unique tile types required, of assembling various patterns. We first demonstrate how to efficiently self-assemble a set of simple patterns, then show tight bounds on the tile type complexity of self-assembling 2-colored patterns on the surfaces of square assemblies. Finally, we demonstrate an exponential gap in tile type complexity of self-assembling an infinite series of patterns between systems restricted to one plane versus those allowed two planes.
翻译:暂无翻译
相关内容
ACM/IEEE第23届模型驱动工程语言和系统国际会议,是模型驱动软件和系统工程的首要会议系列,由ACM-SIGSOFT和IEEE-TCSE支持组织。自1998年以来,模型涵盖了建模的各个方面,从语言和方法到工具和应用程序。模特的参加者来自不同的背景,包括研究人员、学者、工程师和工业专业人士。MODELS 2019是一个论坛,参与者可以围绕建模和模型驱动的软件和系统交流前沿研究成果和创新实践经验。今年的版本将为建模社区提供进一步推进建模基础的机会,并在网络物理系统、嵌入式系统、社会技术系统、云计算、大数据、机器学习、安全、开源等新兴领域提出建模的创新应用以及可持续性。
官网链接:http://www.modelsconference.org/
专知会员服务
54+阅读 · 2020年1月30日
专知会员服务
34+阅读 · 2019年10月18日
专知会员服务
36+阅读 · 2019年10月17日
Arxiv
13+阅读 · 2022年11月10日
Arxiv
11+阅读 · 2021年9月3日
Arxiv
17+阅读 · 2021年6月18日
Arxiv
10+阅读 · 2018年5月10日