Contamination Bias in Linear Regressions

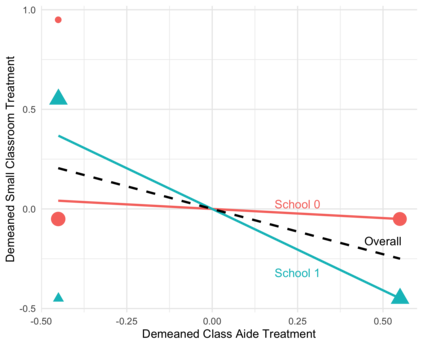
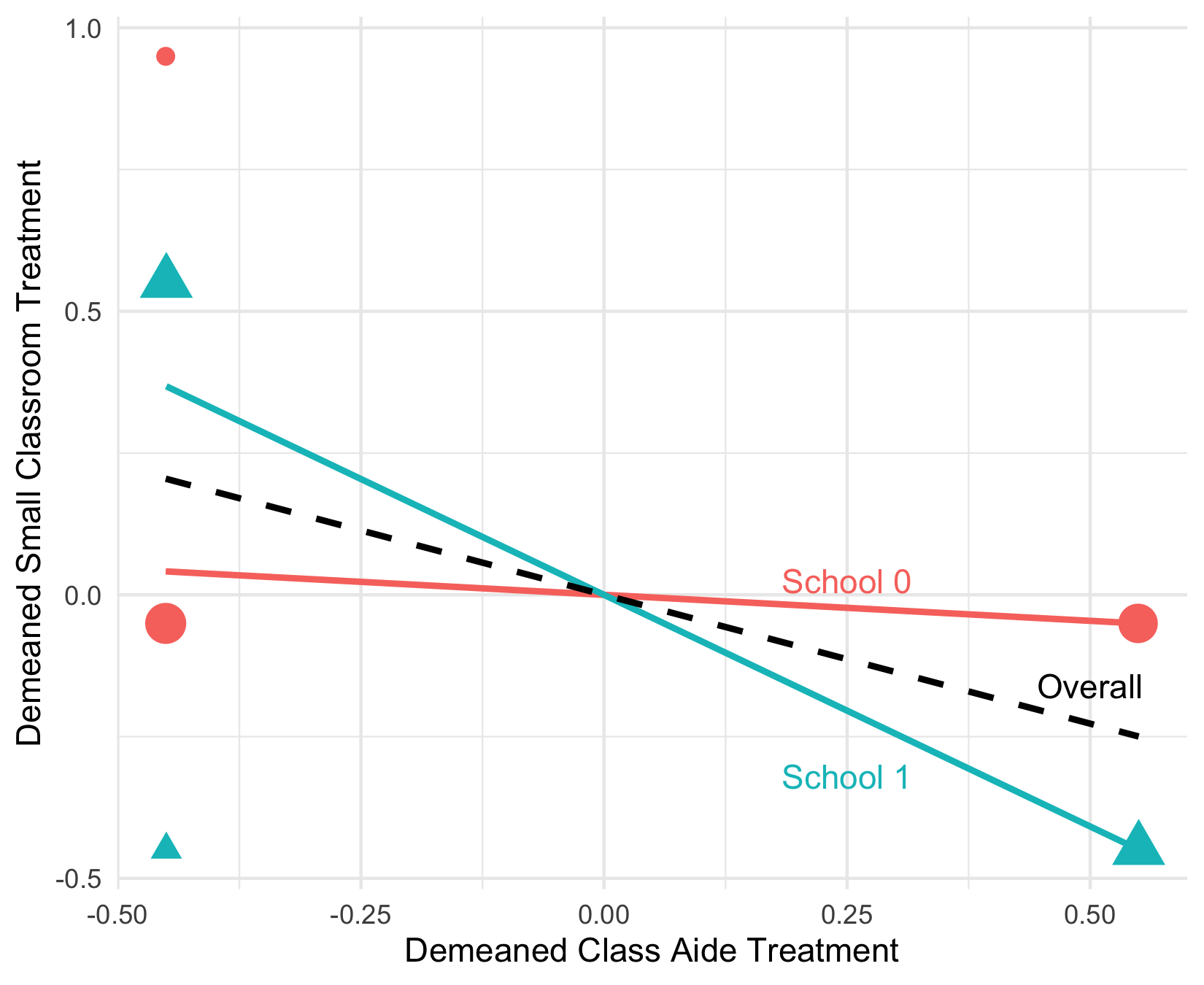
We study regressions with multiple treatments and a set of controls that is flexible enough to purge omitted variable bias. We show these regressions generally fail to estimate convex averages of heterogeneous treatment effects; instead, estimates of each treatment's effect are contaminated by non-convex averages of the effects of other treatments. We discuss three estimation approaches that avoid such contamination bias, including a new estimator of efficiently weighted average effects. We find minimal bias in a re-analysis of Project STAR, due to idiosyncratic effect heterogeneity. But sizeable contamination bias arises when effect heterogeneity becomes correlated with treatment propensity scores.