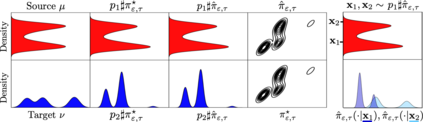
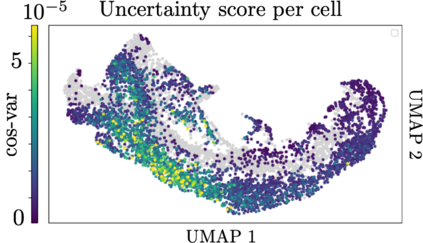
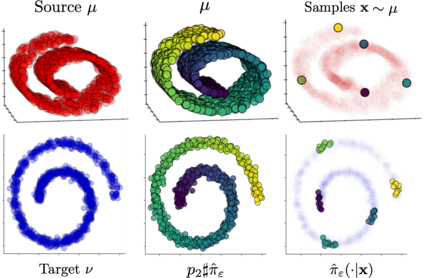
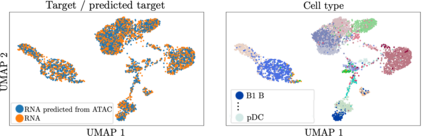
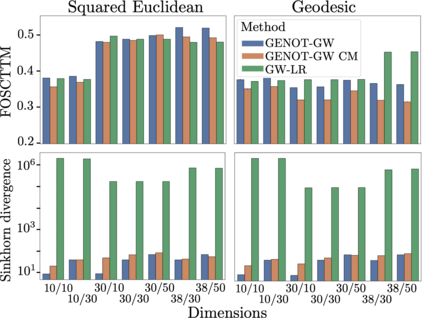
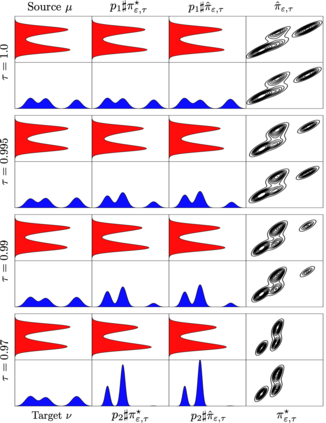
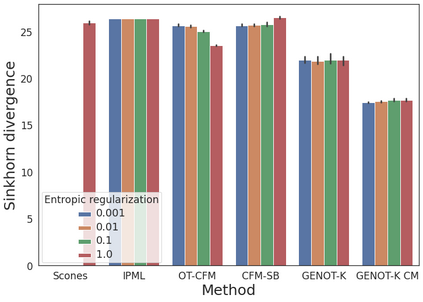
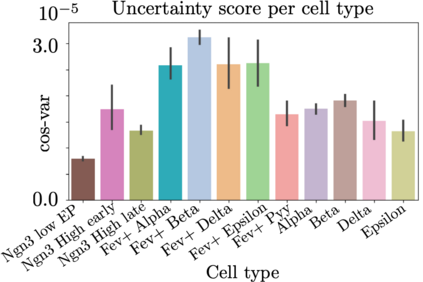
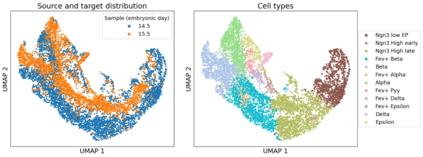
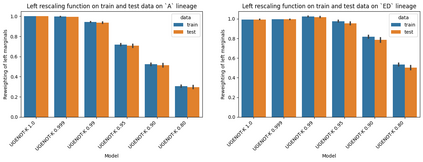
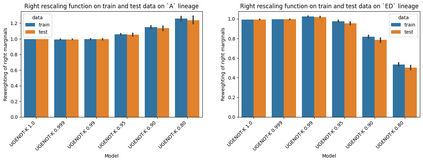
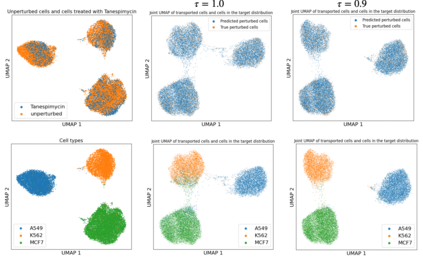
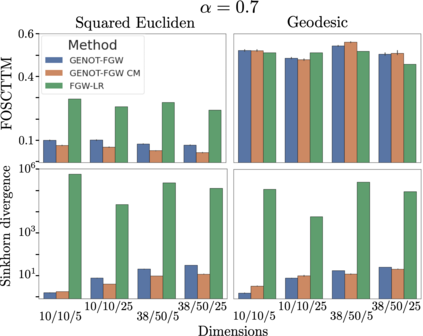
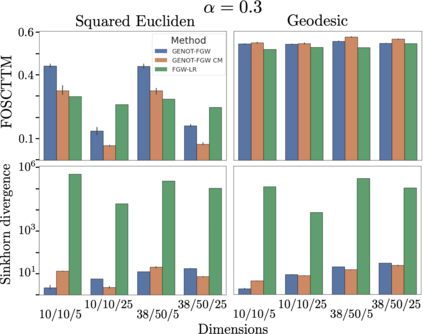
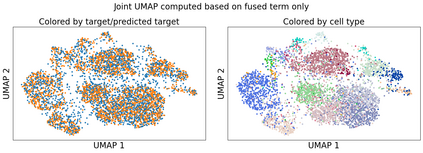
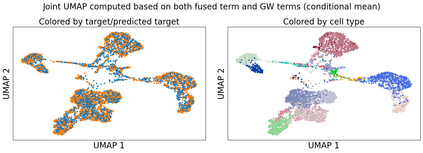
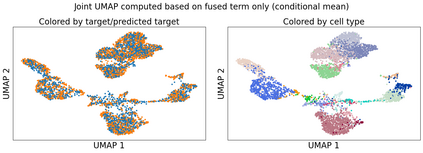
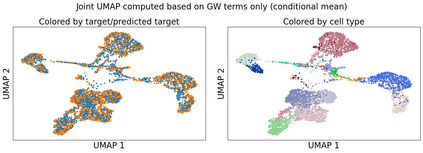
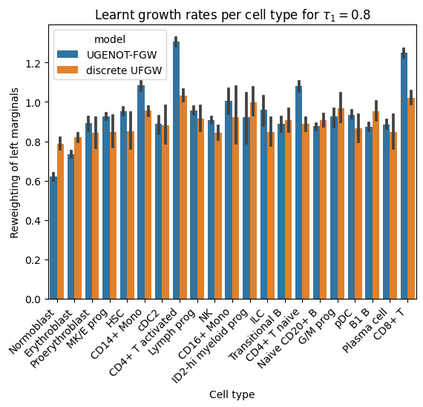
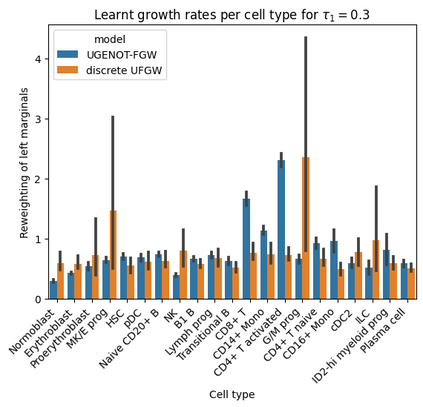
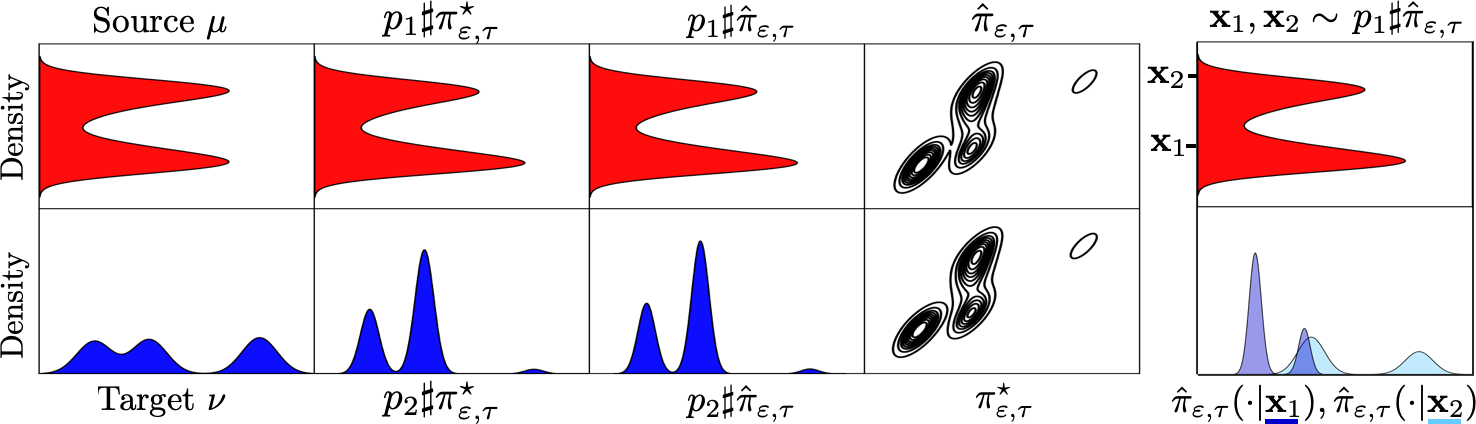
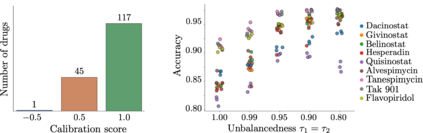Learning measure-to-measure mappings is a crucial task in machine learning, featured prominently in generative modeling. Recent years have witnessed a surge of techniques that draw inspiration from optimal transport (OT) theory. Combined with neural network models, these methods collectively known as \textit{Neural OT} use optimal transport as an inductive bias: such mappings should be optimal w.r.t. a given cost function, in the sense that they are able to move points in a thrifty way, within (by minimizing displacements) or across spaces (by being isometric). This principle, while intuitive, is often confronted with several practical challenges that require adapting the OT toolbox: cost functions other than the squared-Euclidean cost can be challenging to handle, the deterministic formulation of Monge maps leaves little flexibility, mapping across incomparable spaces raises multiple challenges, while the mass conservation constraint inherent to OT can provide too much credit to outliers. While each of these mismatches between practice and theory has been addressed independently in various works, we propose in this work an elegant framework to unify them, called \textit{generative entropic neural optimal transport} (GENOT). GENOT can accommodate any cost function; handles randomness using conditional generative models; can map points across incomparable spaces, and can be used as an \textit{unbalanced} solver. We evaluate our approach through experiments conducted on various synthetic datasets and demonstrate its practicality in single-cell biology. In this domain, GENOT proves to be valuable for tasks such as modeling cell development, predicting cellular responses to drugs, and translating between different data modalities of cells.
翻译:暂无翻译