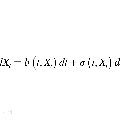脉冲延迟微分方程数值分析

项目名称： 脉冲延迟微分方程数值分析

项目编号： No.11271101

项目类型： 面上项目

立项/批准年度： 2013

项目学科： 数理科学和化学

项目作者： 丁效华

作者单位： 哈尔滨工业大学

项目金额： 50万元

中文摘要： 目前，脉冲微分方程- - 作为瞬动型系统的一种数学模型，是科学研究领域中的一个热门问题，它被广泛地应用于生物技术、药物动力学、经济学、种群动力学、流行病学、通讯工程、控制工程等领域。本项目的研究目的是：构造适用于求解脉冲延迟微分方程的高精度的数值方法，如Runge-Kutta方法，研究相应数值离散系统如何保持原系统的某些动力学性质，如稳定性、可控性及鲁棒性等，并利用这些数值方法来研究由脉冲延迟微分方程所描述的数学模型的相应动力特性。本项目涉及到一些新的课题，项目的研究不但在理论上能丰富微分方程数值分析的内涵，同时在实践中具有很好的应用前景。

中文关键词： 脉冲延迟微分方程；随机微分方程；数值方法；收敛性；保结构

英文摘要： Nowadays, impulsive differential equations, as a kind of model of instantaneous systems, have become a hot problem in the scientific research field. They have been applied widely in biotechnology, pharmacokinetics, economics, population dynamics, epidemiology, communication engineering, control engineering, etc. The main purpose of this project is that constuct some highly accurate numerical methods, e.g. Runge-Kutta methods, to solve impulsive delay differential equations. We shall study how the numerical discrete systems preserve some intrinsic dynamical properties, such as stability, controllability, robustness, of the original systems. Furthermore, we shall apply these numerical methods to research the dynamics of some mathematical models, which are described by impulsive delay differential equations. This project concerns on some new subjects, and the study not only riches the connotation of the numerical analysis of differential equations in theory, but also has a good application prospect in practice.

英文关键词： Impulsive delay differential equations；Stochastic differential equations；Numerical methods；Convergence；Structure-preserving