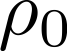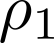Variational Quantum Algorithms (VQAs) may be a path to quantum advantage on Noisy Intermediate-Scale Quantum (NISQ) computers. A natural question is whether noise on NISQ devices places fundamental limitations on VQA performance. We rigorously prove a serious limitation for noisy VQAs, in that the noise causes the training landscape to have a barren plateau (i.e., vanishing gradient). Specifically, for the local Pauli noise considered, we prove that the gradient vanishes exponentially in the number of qubits $n$ if the depth of the ansatz grows linearly with $n$. These noise-induced barren plateaus (NIBPs) are conceptually different from noise-free barren plateaus, which are linked to random parameter initialization. Our result is formulated for a generic ansatz that includes as special cases the Quantum Alternating Operator Ansatz and the Unitary Coupled Cluster Ansatz, among others. For the former, our numerical heuristics demonstrate the NIBP phenomenon for a realistic hardware noise model.
翻译:自然的问题是,新谢克尔装置上的噪音是否对VQA的性能造成根本限制。我们严格证明对吵闹的VQA的严格限制,因为噪音导致培训场景有一个贫瘠的高原(即,渐变的梯度)。具体地说,对于考虑的当地保利噪音来说,我们证明,如果 anaz 的深度以美元线性增长,则梯度会指数性地消失在qubits $n。这些噪音诱发的贫瘠高原在概念上是否与无噪音的高原不同,而这些高原与随机设定参数有关。我们的结果是针对一种通用的atz,其中特别包括Qantum Altherated Ansatz和Unitey Coupd Ansatz。对于前者,我们的数字超音量表明NIBP是一种现实的硬件噪音模型现象。