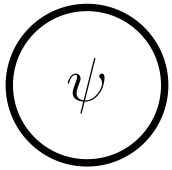
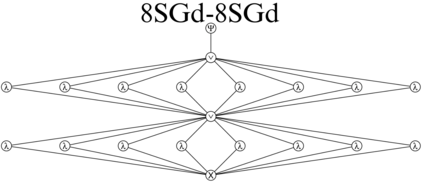
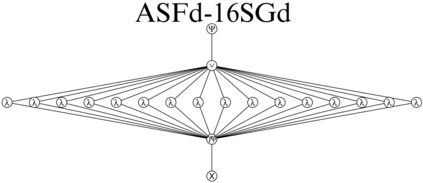
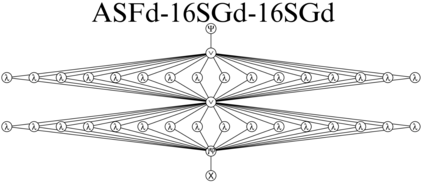
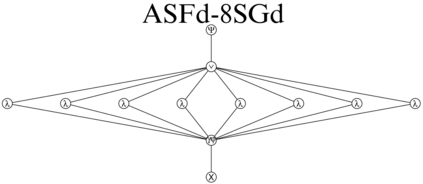
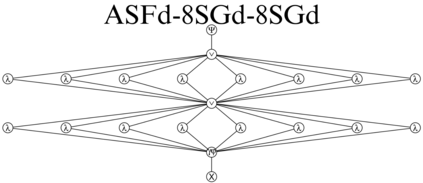
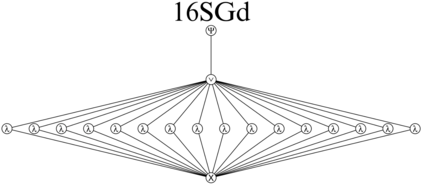
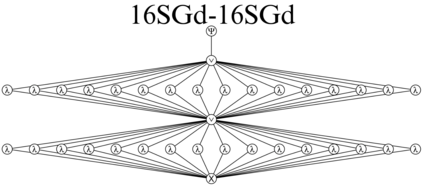
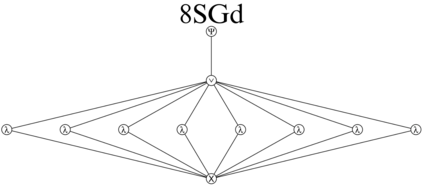
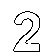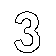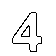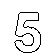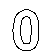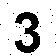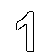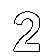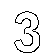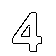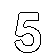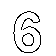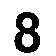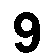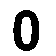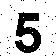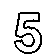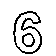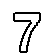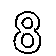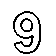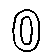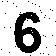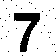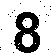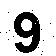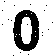A classical approach to designing binary image operators is Mathematical Morphology (MM). We propose the Discrete Morphological Neural Networks (DMNN) for binary image analysis to represent W-operators and estimate them via machine learning. A DMNN architecture, which is represented by a Morphological Computational Graph, is designed as in the classical heuristic design of morphological operators, in which the designer should combine a set of MM operators and Boolean operations based on prior information and theoretical knowledge. Then, once the architecture is fixed, instead of adjusting its parameters (i.e., structural elements or maximal intervals) by hand, we propose a lattice gradient descent algorithm (LGDA) to train these parameters based on a sample of input and output images under the usual machine learning approach. We also propose a stochastic version of the LGDA that is more efficient, is scalable and can obtain small error in practical problems. The class represented by a DMNN can be quite general or specialized according to expected properties of the target operator, i.e., prior information, and the semantic expressed by algebraic properties of classes of operators is a differential relative to other methods. The main contribution of this paper is the merger of the two main paradigms for designing morphological operators: classical heuristic design and automatic design via machine learning. Thus, conciliating classical heuristic morphological operator design with machine learning. We apply the DMNN to recognize the boundary of digits with noise, and we discuss many topics for future research.
翻译:暂无翻译