The Simplest Inflationary Potentials

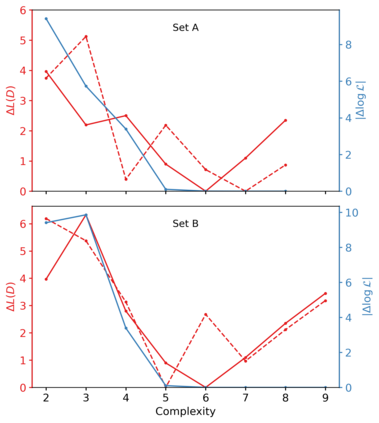
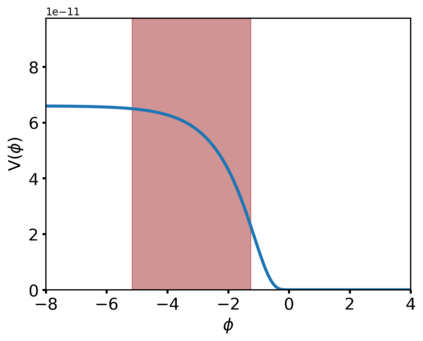
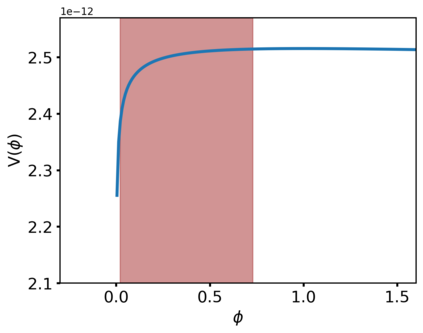
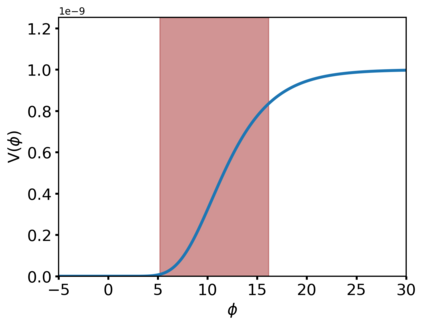
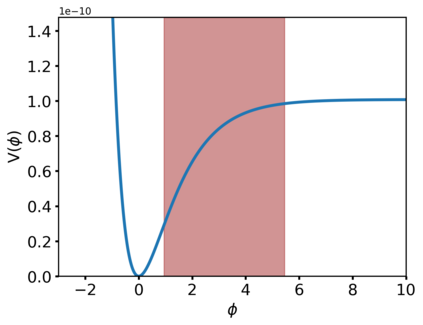
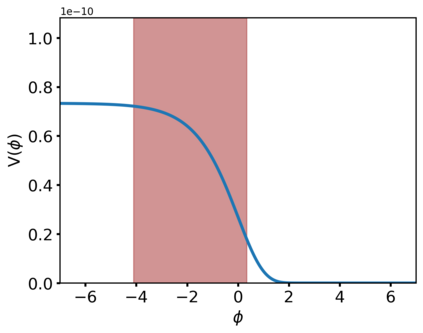
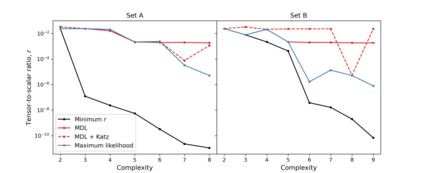
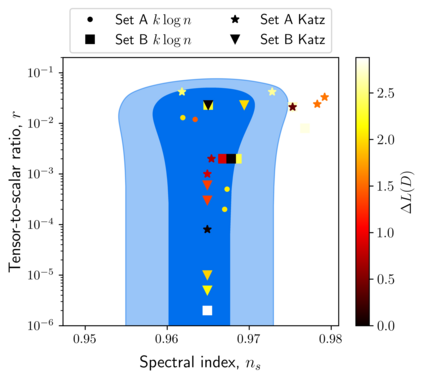
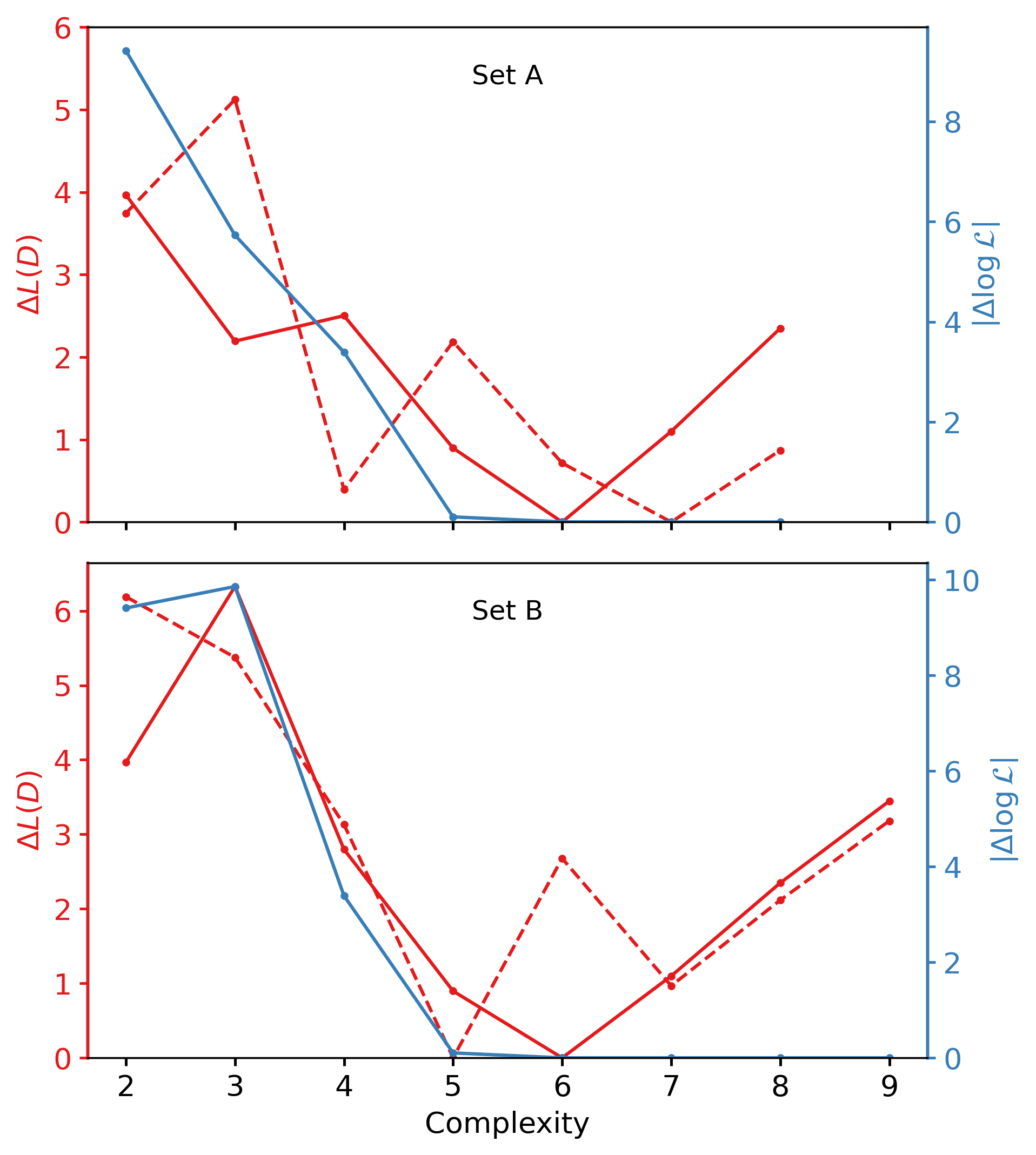
Inflation is a highly favoured theory for the early Universe. It is compatible with current observations of the cosmic microwave background and large scale structure and is a driver in the quest to detect primordial gravitational waves. It is also, given the current quality of the data, highly under-determined with a large number of candidate implementations. We use a new method in symbolic regression to generate all possible simple scalar field potentials for one of two possible basis sets of operators. Treating these as single-field, slow-roll inflationary models we then score them with an information-theoretic metric ("minimum description length") that quantifies their efficiency in compressing the information in the Planck data. We explore two possible priors on the parameter space of potentials, one related to the functions' structural complexity and one that uses a Katz back-off language model to prefer functions that may be theoretically motivated. This enables us to identify the inflaton potentials that optimally balance simplicity with accuracy at explaining the Planck data, which may subsequently find theoretical motivation. Our exploratory study opens the door to extraction of fundamental physics directly from data, and may be augmented with more refined theoretical priors in the quest for a complete understanding of the early Universe.