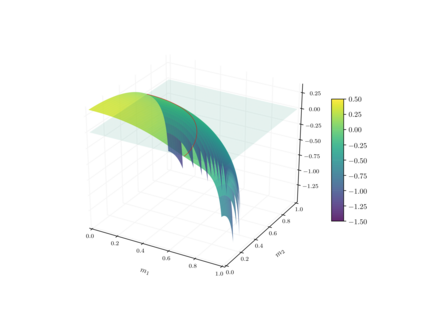
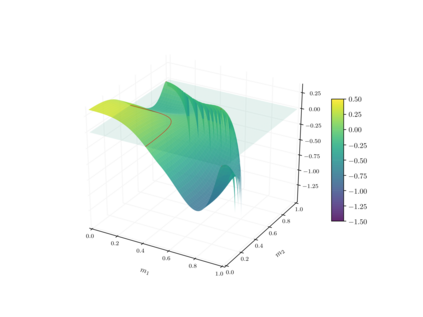
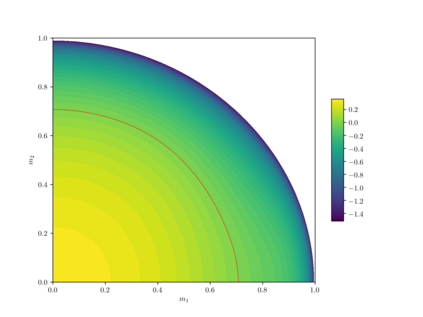
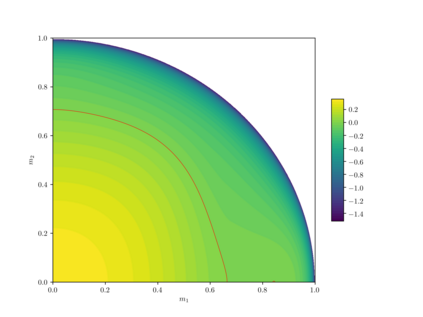
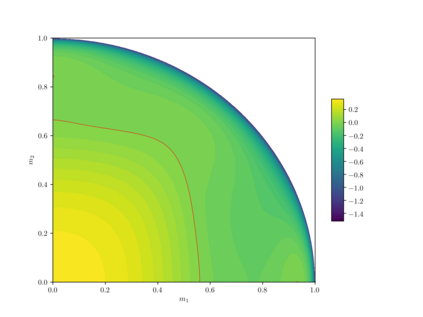
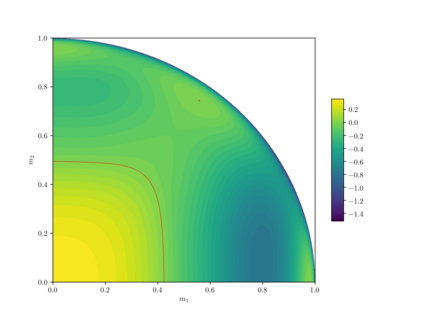
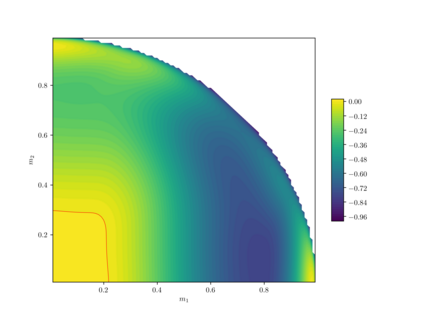
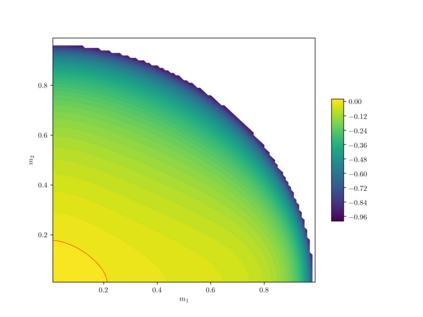
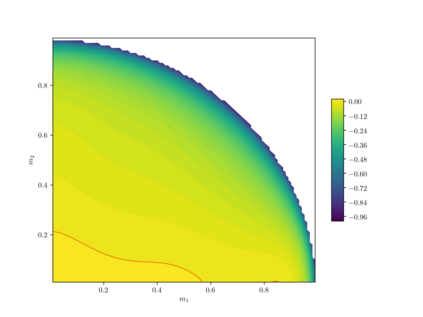
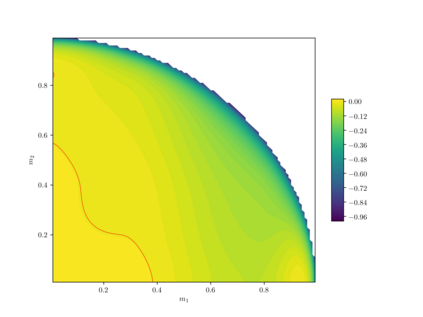
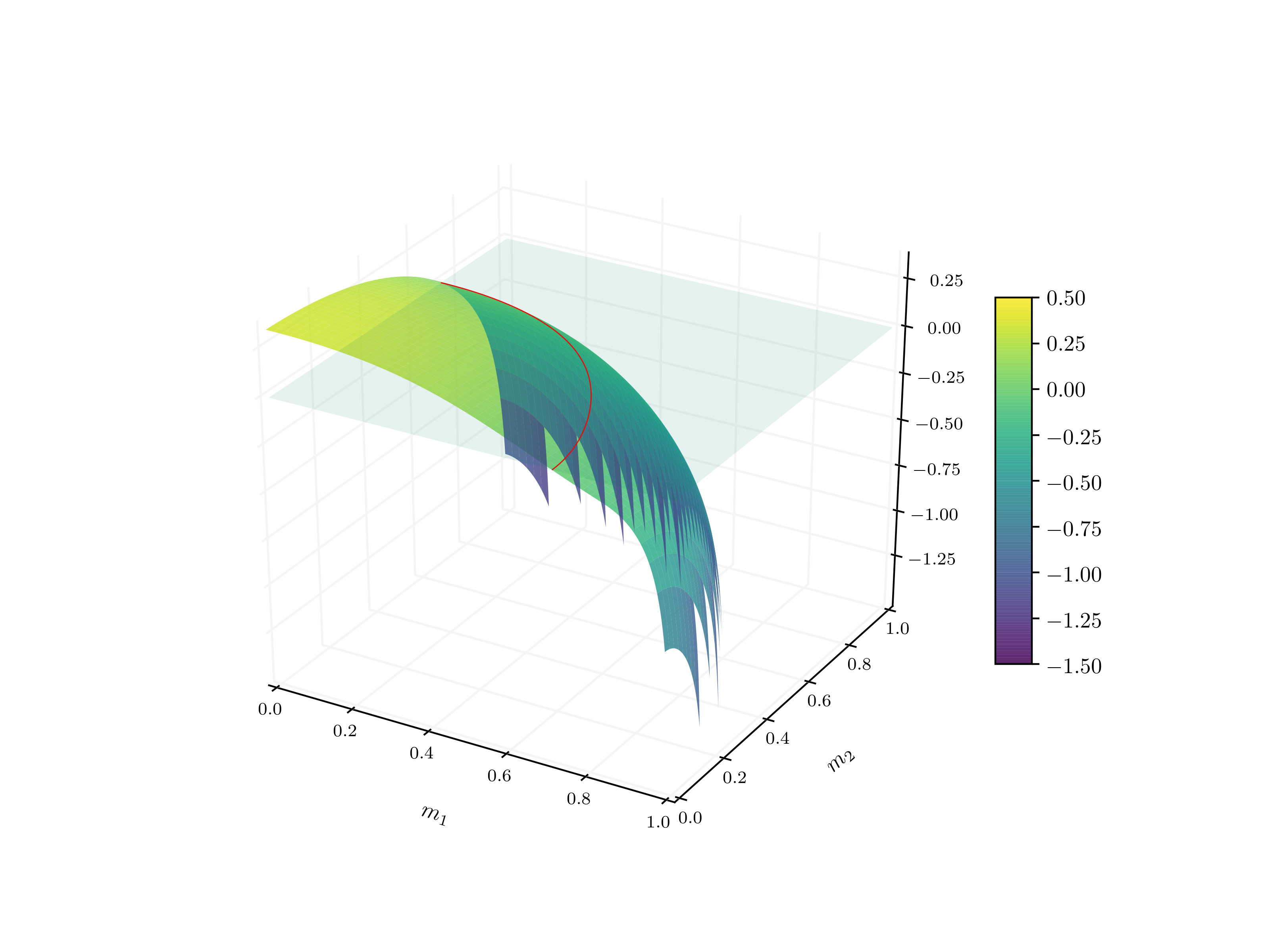
We study the annealed complexity of a random Gaussian homogeneous polynomial on the $N$-dimensional unit sphere in the presence of deterministic polynomials that depend on fixed unit vectors and external parameters. In particular, we establish variational formulas for the exponential asymptotics of the average number of total critical points and of local maxima. This is obtained through the Kac-Rice formula and the determinant asymptotics of a finite-rank perturbation of a Gaussian Wigner matrix. More precisely, the determinant analysis is based on recent advances on finite-rank spherical integrals by [Guionnet, Husson 2022] to study the large deviations of multi-rank spiked Gaussian Wigner matrices. The analysis of the variational problem identifies a topological phase transition. There is an exact threshold for the external parameters such that, once exceeded, the complexity function vanishes into new regions in which the critical points are close to the given vectors. Interestingly, these regions also include those where critical points are close to multiple vectors.
翻译:暂无翻译