Online Multivariate Regularized Distributional Regression for High-dimensional Probabilistic Electricity Price Forecasting

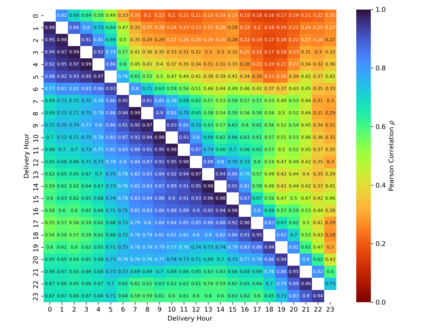
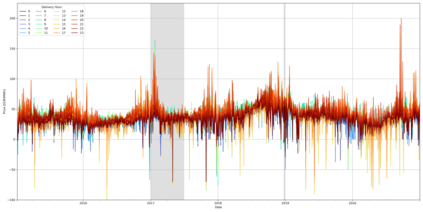
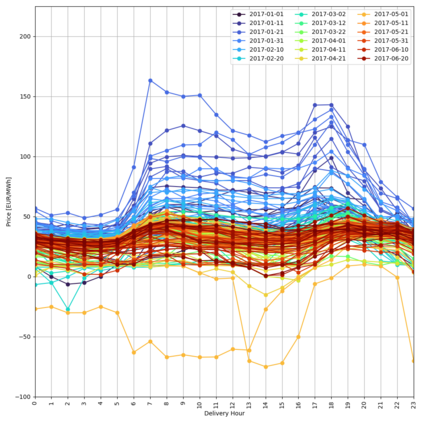
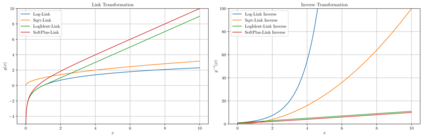
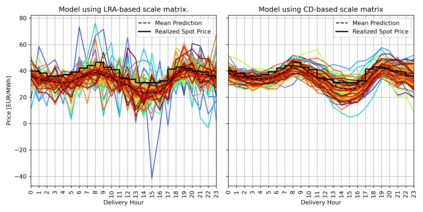
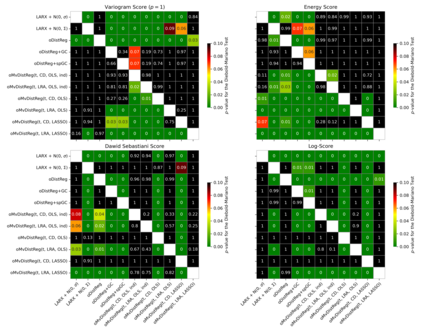
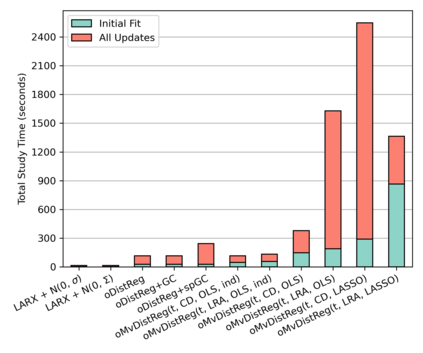
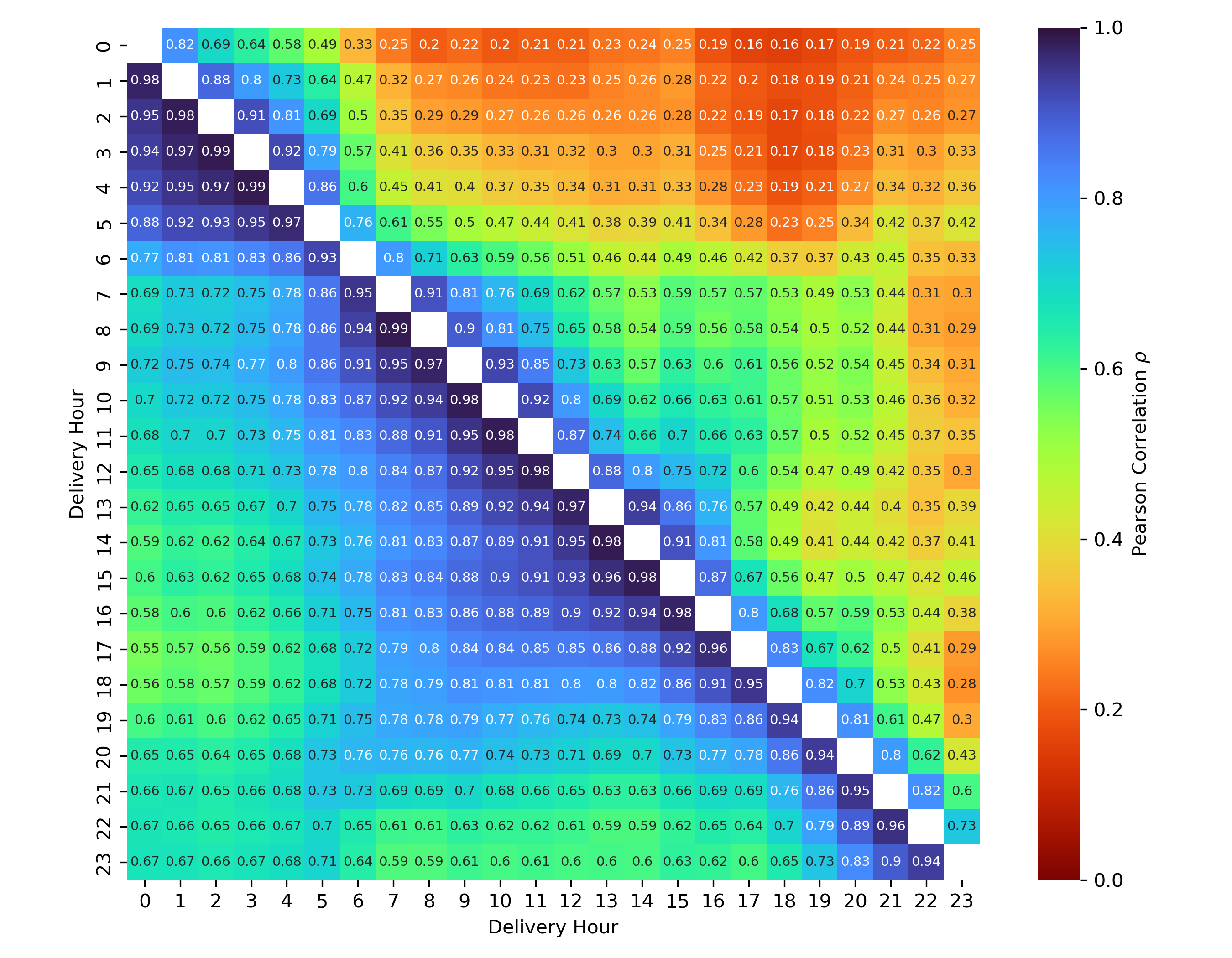
Probabilistic electricity price forecasting (PEPF) is a key task for market participants in short-term electricity markets. The increasing availability of high-frequency data and the need for real-time decision-making in energy markets require online estimation methods for efficient model updating. We present an online, multivariate, regularized distributional regression model, allowing for the modeling of all distribution parameters conditional on explanatory variables. Our approach is based on the combination of the multivariate distributional regression and an efficient online learning algorithm based on online coordinate descent for LASSO-type regularization. Additionally, we propose to regularize the estimation along a path of increasingly complex dependence structures of the multivariate distribution, allowing for parsimonious estimation and early stopping. We validate our approach through one of the first forecasting studies focusing on multivariate probabilistic forecasting in the German day-ahead electricity market while using only online estimation methods. We compare our approach to online LASSO-ARX-models with adaptive marginal distribution and to online univariate distributional models combined with an adaptive Copula. We show that the multivariate distributional regression, which allows modeling all distribution parameters - including the mean and the dependence structure - conditional on explanatory variables such as renewable in-feed or past prices provide superior forecasting performance compared to modeling of the marginals only and keeping a static/unconditional dependence structure. Additionally, online estimation yields a speed-up by a factor of 80 to over 400 times compared to batch fitting.