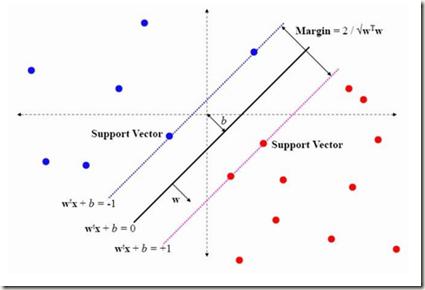
We investigate sublinear classical and quantum algorithms for matrix games, a fundamental problem in optimization and machine learning, with provable guarantees. Given a matrix $A\in\mathbb{R}^{n\times d}$, sublinear algorithms for the matrix game $\min_{x\in\mathcal{X}}\max_{y\in\mathcal{Y}} y^{\top} Ax$ were previously known only for two special cases: (1) $\mathcal{Y}$ being the $\ell_{1}$-norm unit ball, and (2) $\mathcal{X}$ being either the $\ell_{1}$- or the $\ell_{2}$-norm unit ball. We give a sublinear classical algorithm that can interpolate smoothly between these two cases: for any fixed $q\in (1,2]$, we solve the matrix game where $\mathcal{X}$ is a $\ell_{q}$-norm unit ball within additive error $\epsilon$ in time $\tilde{O}((n+d)/{\epsilon^{2}})$. We also provide a corresponding sublinear quantum algorithm that solves the same task in time $\tilde{O}((\sqrt{n}+\sqrt{d})\textrm{poly}(1/\epsilon))$ with a quadratic improvement in both $n$ and $d$. Both our classical and quantum algorithms are optimal in the dimension parameters $n$ and $d$ up to poly-logarithmic factors. Finally, we propose sublinear classical and quantum algorithms for the approximate Carath\'eodory problem and the $\ell_{q}$-margin support vector machines as applications.
翻译:我们调查矩阵游戏的亚线性古典算法和量子算法,这是优化和机器学习的一个根本问题,有可辨识的保证。根据一个矩阵 $A\ in\ mathb{R\\ n\\\ ntime d} 美元,矩阵游戏的亚线性古典算法 $\ min\\ x\\ nin\\ mathcal{Y\\\\\\\\\\\\\ y ⁇ { x} Ax 只有在两个特殊的情况下才知道:(1) $\ mathcal{Y} $( ell%1} 美元), 和 (2) $\ mathcal{X} 美元, 或 $\ ellclational2\ 美元 单位球。 我们给出一个子线性经典算法, 任何固定的 $q\ 美元(1, 2), 我们解决了矩阵游戏, $mexcal{q} 美元是 美元 美元 美元 美元, 美元是 美元 美元 美元 美元 美元 美元 和 美元 美元 美元 美元 和 美元 美元 美元 美元 美元 美元 美元 和 美元 美元 美元 也提供 美元 美元 美元 美元 和 美元 美元 美元 美元 美元 美元 美元 和 美元 美元 美元 美元 美元 美元 美元 美元 和 等 等 和 等 等 等 的 的 等 的 的 和 等 等 。