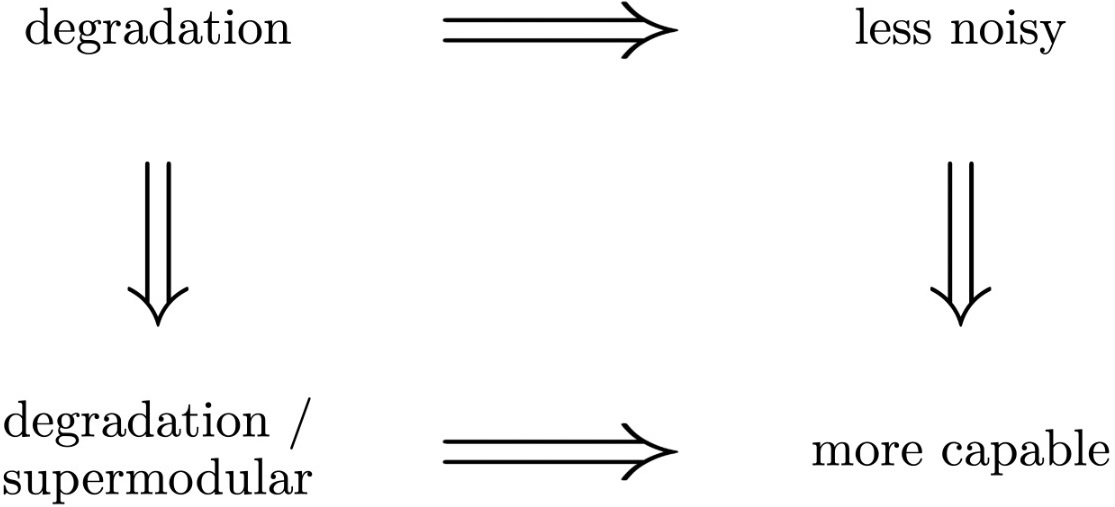
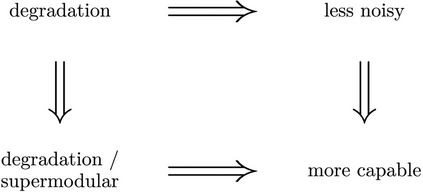
The partial information decomposition (PID) framework is concerned with decomposing the information that a set of random variables has with respect to a target variable into three types of components: redundant, synergistic, and unique. Classical information theory alone does not provide a unique way to decompose information in this manner and additional assumptions have to be made. Recently, Kolchinsky proposed a new general axiomatic approach to obtain measures of redundant information, based on choosing an order relation between information sources (equivalently, order between communication channels). In this paper, we exploit this approach to introduce three new measures of redundant information (and the resulting decompositions) based on well-known preorders between channels, thus contributing to the enrichment of the PID landscape. We relate the new decompositions to existing ones, study some of their properties, and provide examples illustrating their novelty. As a side result, we prove that any preorder that satisfies Kolchinsky's axioms yields a decomposition that meets the axioms originally introduced by Williams and Beer when they first propose the PID.
翻译:暂无翻译