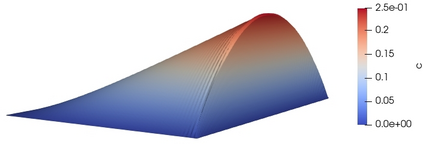
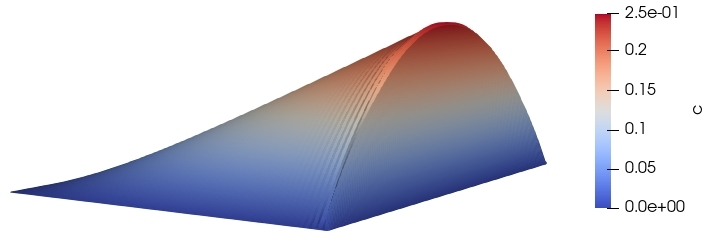
In this work, we propose a residual-based a posteriori error estimator for algebraic flux-corrected (AFC) schemes for stationary convection-diffusion equations. A global upper bound is derived for the error in the energy norm for a general choice of the limiter, which defines the nonlinear stabilization term. In the diffusion-dominated regime, the estimator has the same convergence properties as the true error. A second approach is discussed, where the upper bound is derived in a posteriori way using the Streamline Upwind Petrov Galerkin (SUPG) estimator proposed in \cite{JN13}. Numerical examples study the effectivity index and the adaptive grid refinement for two limiters in two dimensions.
翻译:在这项工作中,我们建议为固定对流扩散方程式的代数流变校正(AFC)方案提出一个基于残余的事后误差估计仪。为一般选择限制值,从能源规范错误中得出一个全球上限,以界定非线性稳定值。在以扩散为主的制度中,估计仪具有与真实错误相同的趋同特性。第二个办法是,利用在\cite{JN13}中提议的精锐Upple风 Petrov Galerkin(SUPG)估计仪,以事后方式得出上限值。数字实例研究两种限制值的效应指数和适应性电网改进的两个方面。